Use this referral: Hole No. Par Dustins Score Martins Score 1 4 2 5 3 3 4 4 5 3 6 5 7 5 8
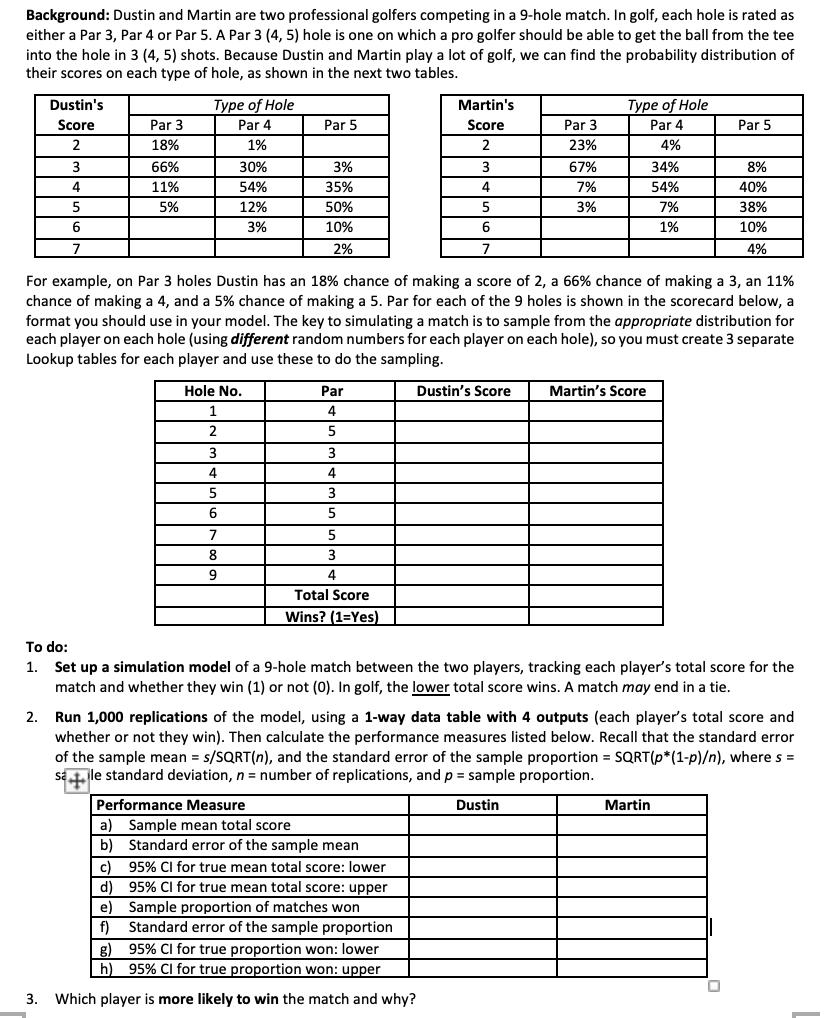 Use this referral:
Use this referral:
Hole No. | Par | Dustin’s Score | Martin’s Score |
1 | 4 | ||
2 | 5 | ||
3 | 3 | ||
4 | 4 | ||
5 | 3 | ||
6 | 5 | ||
7 | 5 | ||
8 | 3 | ||
9 | 4 | ||
Total Score | |||
Wins? (1=Yes) |
Background: Dustin and Martin are two professional golfers competing in a 9-hole match. In golf, each hole is rated as either a Par 3, Par 4 or Par 5. A Par 3 (4, 5) hole is one on which a pro golfer should be able to get the ball from the tee into the hole in 3 (4, 5) shots. Because Dustin and Martin play a lot of golf, we can find the probability distribution of their scores on each type of hole, as shown in the next two tables. Dustin's Score 2 3 4 5 6 7 Par 3 18% 66% 11% 5% 2. Type of Hole Par 4 1% Hole No. 1 2 3 4 30% 54% 12% 3% 5 6 7 8 9 Par 5 3% 35% 50% 10% 2% Par 4 5 3 4 3 5 For example, on Par 3 holes Dustin has an 18% chance of making a score of 2, a 66% chance of making a 3, an 11% chance of making a 4, and a 5% chance of making a 5. Par for each of the 9 holes is shown in the scorecard below, a format you should use in your model. The key to simulating a match is to sample from the appropriate distribution for each player on each hole (using different random numbers for each player on each hole), so you must create 3 separate Lookup tables for each player and use these to do the sampling. 5 3 4 Total Score Wins? (1-Yes) Martin's Score 2 3 4 5 6 7 Performance Measure a) Sample mean total score b) Standard error of the sample mean c) d Dustin's Score 95% CI for true mean total score: lower 95% CI for true mean total score: upper Par 3 23% 67% 7% 3% Type of Hole Par 4 4% e) Sample proportion of matches won f) Standard error of the sample proportion g) 95% CI for true proportion won: lower h) 95% CI for true proportion won: upper 3. Which player is more likely to win the match and why? Martin's Score 34% 54% To do: 1. Set up a simulation model of a 9-hole match between the two players, tracking each player's total score for the match and whether they win (1) or not (0). In golf, the lower total score wins. A match may end in a tie. 7% 1% Par 5 Run 1,000 replications of the model, using a 1-way data table with 4 outputs (each player's total score and whether or not they win). Then calculate the performance measures listed below. Recall that the standard error of the sample mean = s/SQRT(n), and the standard error of the sample proportion = SQRT (p*(1-p)/n), where s = sale standard deviation, n = number of replications, and p = sample proportion. 4+1 Dustin Martin 8% 40% 38% 10% 4%
Step by Step Solution
3.49 Rating (156 Votes )
There are 3 Steps involved in it
Step: 1

See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


