Question
Bayesian statistics provides an alternative approach to the classical inferential statistics that we studied in Stat 252 this semester. One important feature of Bayesian statistics
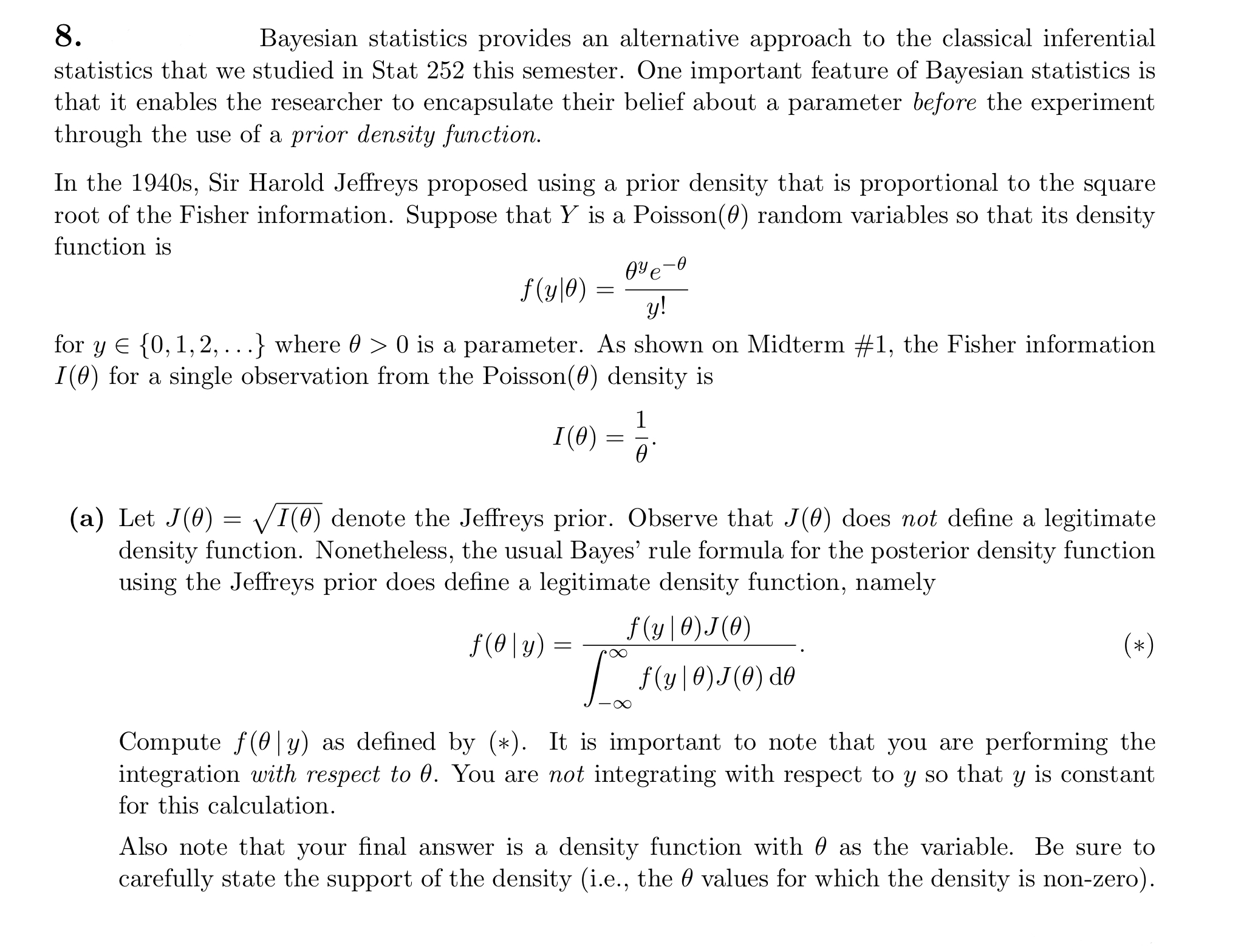
Bayesian statistics provides an alternative approach to the classical inferential statistics that we studied in Stat 252 this semester. One important feature of Bayesian statistics is that it enables the researcher to encapsulate their belief about a parameter before the experiment through the use of a prior density function. In the 1940s, Sir Harold Jeffreys proposed using a prior density that is proportional to the square root of the Fisher information. Suppose that Y is a Poisson(?) random variables so that its density function is f(y|?) = ?ye?? y! for y ? {0, 1, 2, . . .} where ? > 0 is a parameter. As shown on Midterm #1, the Fisher information I(?) for a single observation from the Poisson(?) density is I ( ? ) = 1? . (a) Let J(?) = ?I(?) denote the Jeffreys prior. Observe that J(?) does not define a legitimate density function. Nonetheless, the usual Bayes' rule formula for the posterior density function using the Jeffreys prior does define a legitimate density function, namely f(y|?)J(?) f(?|y)= ? ? f(y|?)J(?)d? ?? . (?) Compute f (? | y) as defined by (?). It is important to note that you are integration with respect to ?. You are not integrating with respect to y so that y is constant for this calculation. Also note that your final answer is a density function with ? as the variable. Be sure to carefully state the support of the density (i.e., the ? values for which the density is non-zero).
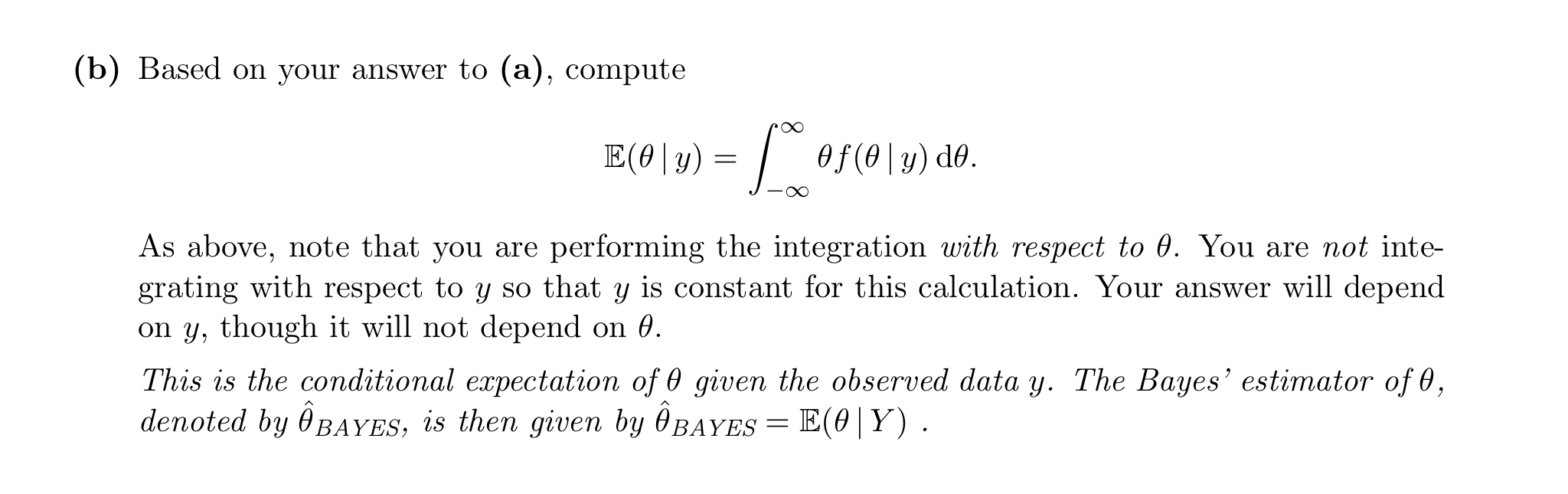
(b) Based on your answer to (a), compute E(?|y) = As above, note that you are performing the integration with respect to ?. You are not inte- grating with respect to y so that y is constant for this calculation. Your answer will depend on y, though it will not depend on ?. This is the conditional expectation of ? given the observed data y. The Bayes' estimator of ?, denoted by ??BAYES, is then given by ??BAYES = E(? | Y ) .
Step by Step Solution
There are 3 Steps involved in it
Step: 1

Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


