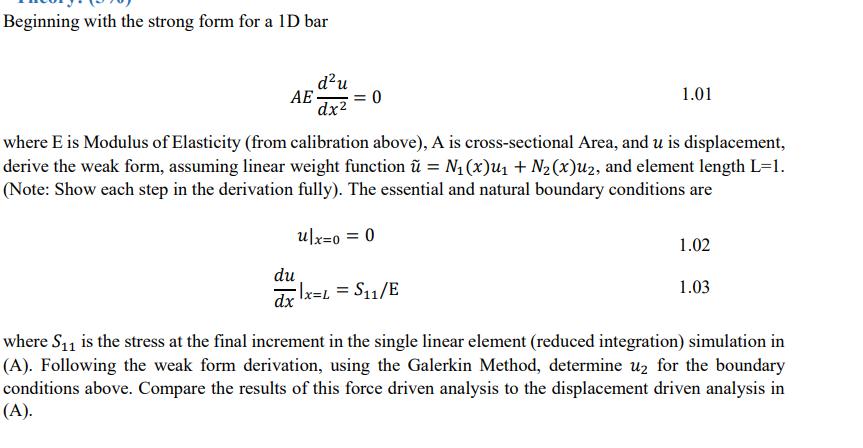
Beginning with the strong form for a 1D bar AE du dx = 0 1.01 where E is Modulus of Elasticity (from calibration above), A is cross-sectional Area, and u is displacement, derive the weak form, assuming linear weight function = N (x)U + N(x)u2, and element length L=1. (Note: Show each step in the derivation fully). The essential and natural boundary conditions are ulx=0 = 0 du dx x= L = S1/E 1.02 1.03 where S1 is the stress at the final increment in the single linear element (reduced integration) simulation in (A). Following the weak form derivation, using the Galerkin Method, determine u for the boundary conditions above. Compare the results of this force driven analysis to the displacement driven analysis in (A).