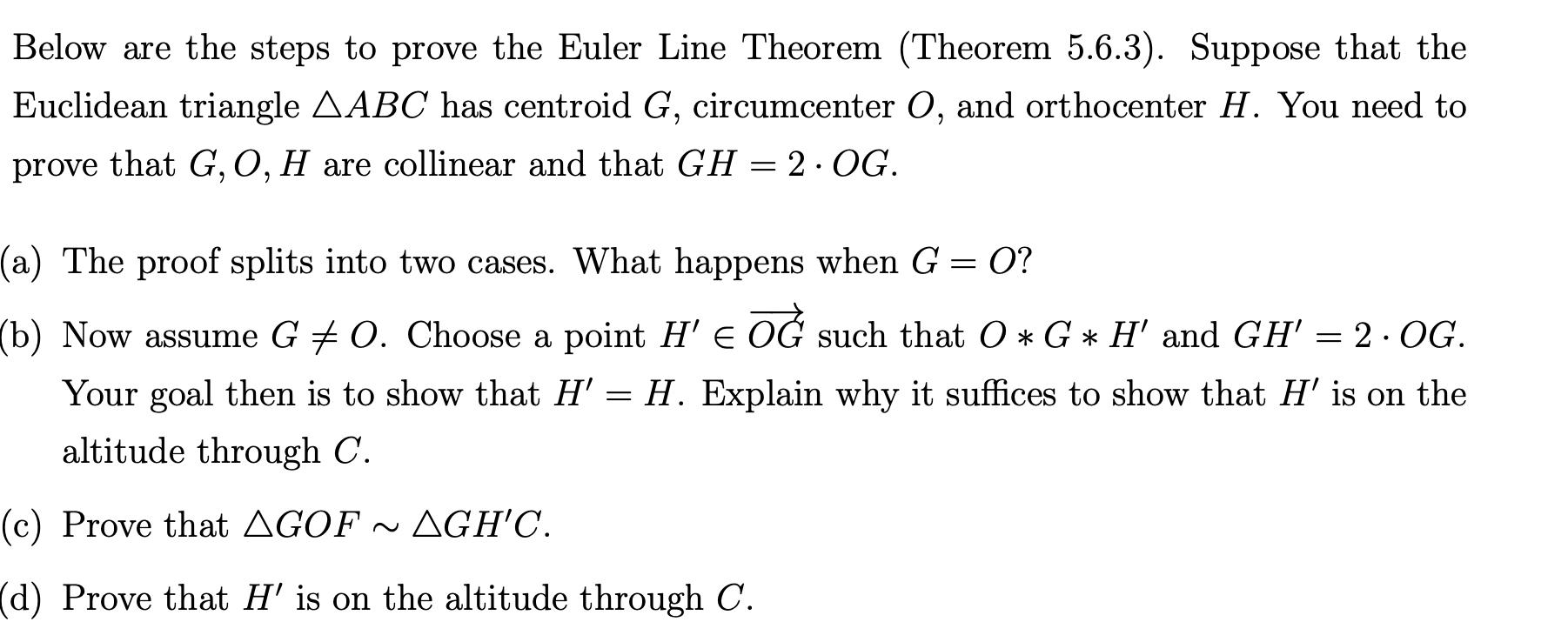
Below are the steps to prove the Euler Line Theorem (Theorem 5.6.3). Suppose that the Euclidean triangle AABC has centroid G, circumcenter O, and orthocenter H. You need to prove that G, O, H are collinear and that GH = 2.OG. (a) The proof splits into two cases. What happens when G = O? (b) Now assume G O. Choose a point H' O such that O * G* H' and GH' E 2.OG. Your goal then is to show that H' = H. Explain why it suffices to show that H' is on the altitude through C. (c) Prove that AGOF ~ AGH'C. (d) Prove that H' is on the altitude through C. = Theorem 5.6.3 (Euler Line Theorem). The orthocenter H, the circumcenter O, and the centroid G of any triangle are collinear. Furthermore, G is between H and 0 (unless the triangle is equilateral, in which case the three points coincide) and HG = 2GO.