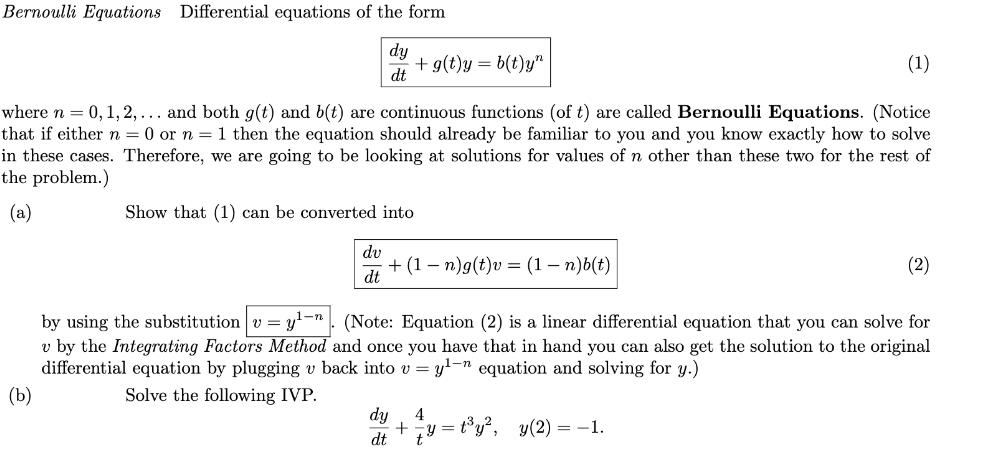
Bernoulli Equations Differential equations of the form dy + g(t)y = b(t)y" (1) dt where n = 0,1, 2, ... and both g(t) and b(t) are continuous functions (of t) are called Bernoulli Equations. (Notice that if either n = 0 or n = 1 then the equation should already be familiar to you and you know exactly how to solve in these cases. Therefore, we are going to be looking at solutions for values of n other than these two for the rest of the problem.) (a) Show that (1) can be converted into dv + (1 - n)g(t)v = (1 n)b(t) dt (2) by using the substitution v = yl-n v by the Integrating Factors Method and once you have that in hand you can also get the solution to the original differential equation by plugging v back into v = yl-n equation and solving for y.) (b) (Note: Equation (2) is a linear differential equation that you can solve for Solve the following IVP. dy 4 y = ty, y(2) = -1. dt