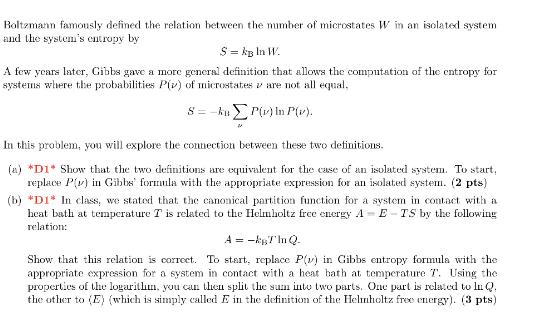
Boltzmann famously defined the relation between the number of microstates W in an isolated system and the system's entropy by S = kB ln W. A few years later, Gibbs gave a more general definition that allows the computation of the entropy for systems where the probabilities P() of microstates are not all equal. 8=-kuP(v) In P(v). 28 In this problem, you will explore the connection between these two definitions. (a) *D1* Show that the two definitions are equivalent for the case of an isolated system. To start, replace P() in Gibbs formula with the appropriate expression for an isolated system. (2 pts) (b) *DI* In class, we stated that the canonical partition function for a system in contact with a heat bath at temperature T is related to the Helmholtz free energy A-E-TS by the following relation: A = -T'In Q. Show that this relation is correct. To start, replace P() in Gibbs entropy formula with the appropriate expression for a system in contact with a heat bath at temperature T. Using the properties of the logarithm, you can then split the sum into two parts. One part is related to ln Q. the other to (E) (which is simply called E in the definition of the Helmholtz free energy). (3 pts)