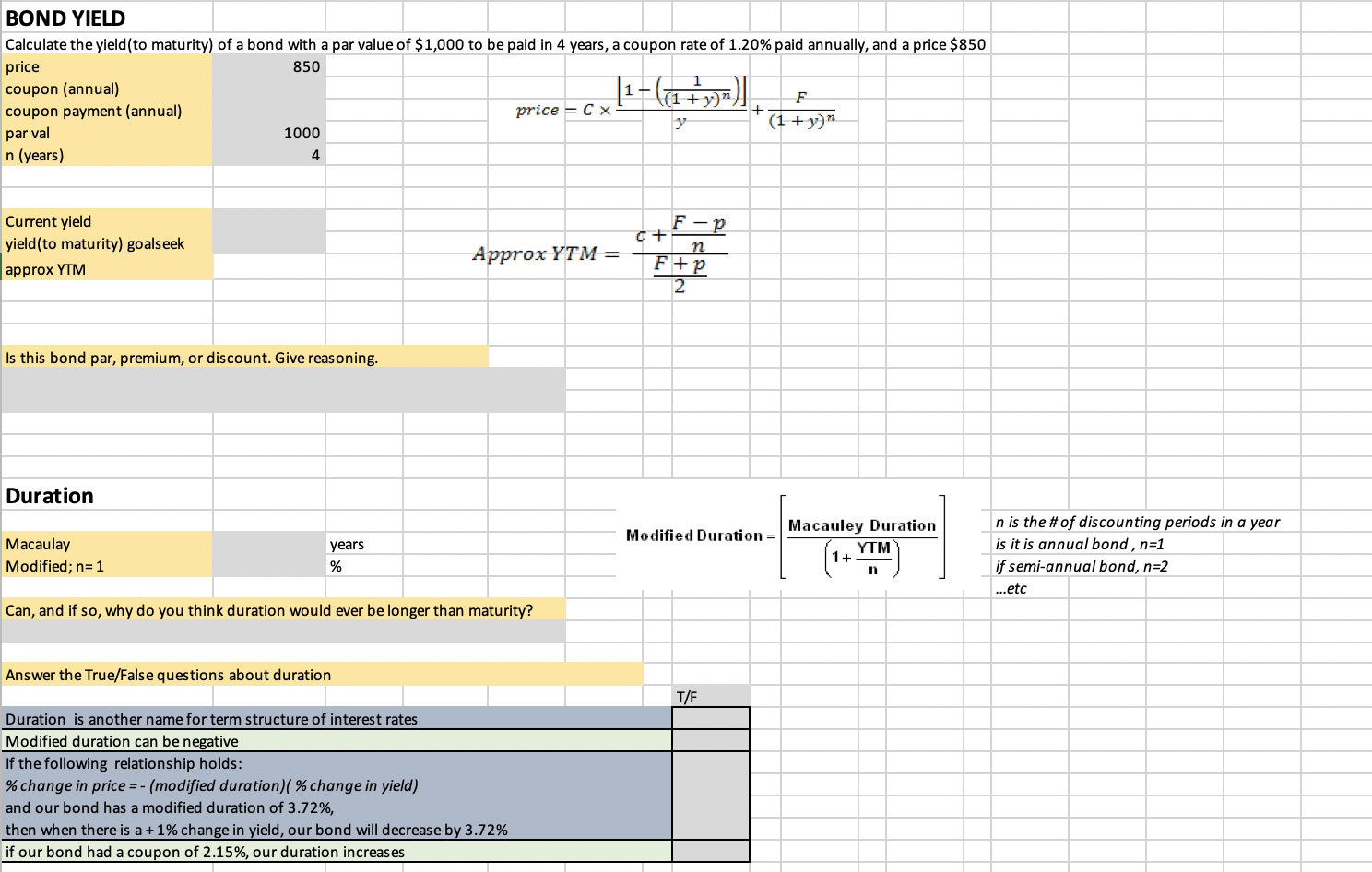
BOND YIELD Calculate the yield(to maturity) of a bond with a par value of $1,000 to be paid in 4 years, a coupon rate of 1.20% paid annually, and a price $850 price 850 coupon (annual) coupon payment (annual) F price = CX + par val 1000 y (1+y) n (years) 4 [1-(+ya] +5) F-p C+ Current yield yield(to maturity) goalseek approx YTM n Approx YTM= - PER F+p 2 Is this bond par, premium, or discount. Give reasoning. Duration Modified Duration = Macaulay Modified; n=1 years % Macauley Duration YTM 1+ n is the # of discounting periods in a year is it is annual bond, n=1 if semi-annual bond, n=2 ...etc n Can, and if so, why do you think duration would ever be longer than maturity? Answer the True/False questions about duration T/F Duration is another name for term structure of interest rates Modified duration can be negative If the following relationship holds: % change in price =- (modified duration)( % change in yield) and our bond has a modified duration of 3.72%, then when there is a +1% change in yield, our bond will decrease by 3.72% if our bond had a coupon of 2.15%, our duration increases BOND YIELD Calculate the yield(to maturity) of a bond with a par value of $1,000 to be paid in 4 years, a coupon rate of 1.20% paid annually, and a price $850 price 850 coupon (annual) coupon payment (annual) F price = CX + par val 1000 y (1+y) n (years) 4 [1-(+ya] +5) F-p C+ Current yield yield(to maturity) goalseek approx YTM n Approx YTM= - PER F+p 2 Is this bond par, premium, or discount. Give reasoning. Duration Modified Duration = Macaulay Modified; n=1 years % Macauley Duration YTM 1+ n is the # of discounting periods in a year is it is annual bond, n=1 if semi-annual bond, n=2 ...etc n Can, and if so, why do you think duration would ever be longer than maturity? Answer the True/False questions about duration T/F Duration is another name for term structure of interest rates Modified duration can be negative If the following relationship holds: % change in price =- (modified duration)( % change in yield) and our bond has a modified duration of 3.72%, then when there is a +1% change in yield, our bond will decrease by 3.72% if our bond had a coupon of 2.15%, our duration increases