Calculus 3 14.4 Reading Assignment: The Chain Rule

I am suck with this question. Please help!

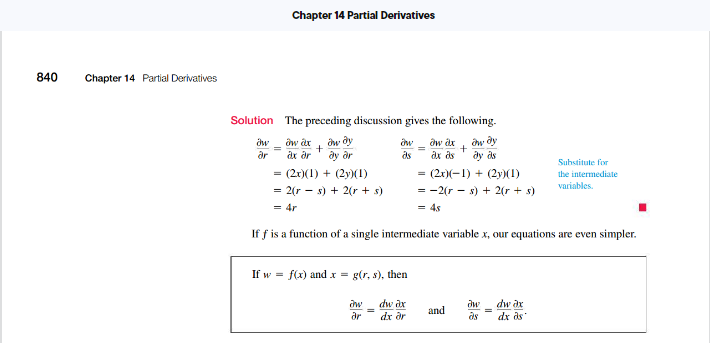
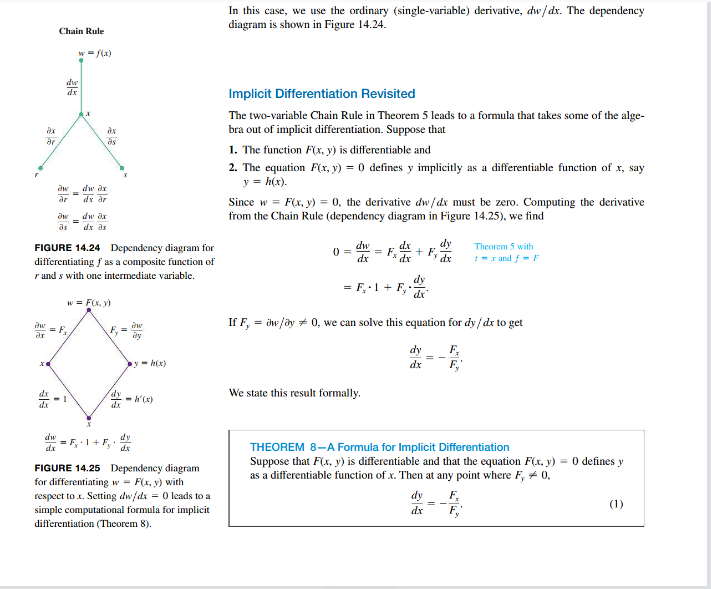
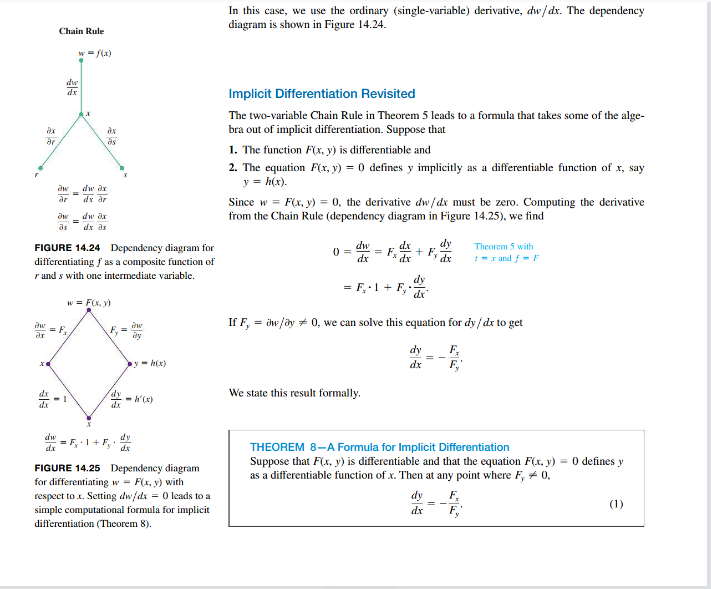
Question 3: Read the introduction to the subsection "Implicit Differentiation Revisited" (p. 840). Explain where the formula for implicit differentiation (boxed as Theorem 8) comes from. One thing to note about these formulas is that when we rewrite them using the fraction notation for partial derivatives, they will look like fraction cancellation. The minus sign means that the fraction cancellation isn't quite correct, but remembering this idea might help with remembering most of this formula.

Chapter 14 Partial Derivatives 840 Chapter 14 Partial Derivatives Solution The preceding discussion gives the following. aw _ow at , aw dy aw _ awar , aw dy ar ar ar ay ar ax as dy ds Substitute for = (20)(1) + (2y)(1) = (20)(-1) + (2))(1) the intermediate = 2(r - 5) + 2(r + 5) = -2(r - $) + 2(r + 5) variables. = 4r = 45 If f is a function of a single intermediate variable x, our equations are even simpler. If w = f(x) and x = g(r, s), then ow _dwax aw _dwax ar dx ar and as dx asIn this case, we use the ordinary (single-variable) derivative, dw/dx. The dependency Chain Rule diagram is shown in Figure 14.24. W =) Implicit Differentiation Revisited The two-variable Chain Rule in Theorem 5 leads to a formula that takes some of the alge- bra out of implicit differentiation. Suppose that 1. The function F(x, y) is differentiable and 2. The equation F(x, y) = 0 defines y implicitly as a differentiable function of x, say y = h(x). dw ax ar dx ar Since w = F(x, y) = 0. the derivative dw /dx must be zero. Computing the derivative ow _ dw ar from the Chain Rule (dependency diagram in Figure 14.25), we find dx as FIGURE 14.24 Dependency diagram for 0 = 41 dw = Fx dx dy Theorem 5 with differentiating f as a composite function of dx 1 = x and f = F / and s with one intermediate variable. dy If F, = dw /by # 0, we can solve this equation for dy / dx to get AT dy dx We state this result formally. ON = F . I+ F . By dx THEOREM 8-A Formula for Implicit Differentiation FIGURE 14.25 Dependency diagram Suppose that F(x, y) is differentiable and that the equation F(x. y) = 0 defines y for differentiating w = F(r, y) with as a differentiable function of x. Then at any point where F, - 0. respect to x. Setting dw/ dr = 0 leads to a dy F. simple computational formula for implicit dx (1) differentiation (Theorem 8)