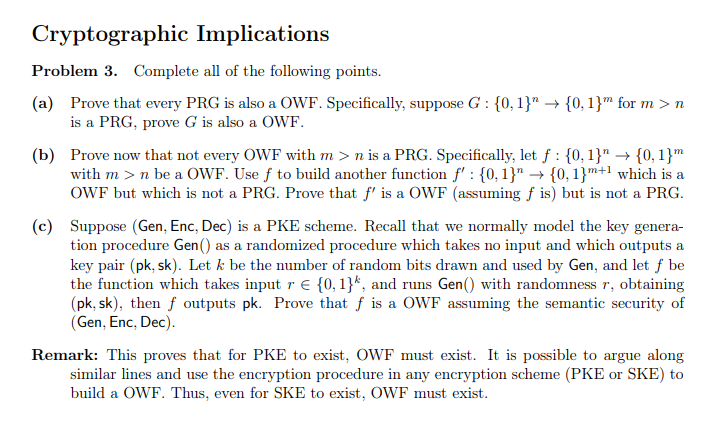
Can you give me detailed answers with mathematical derivations and notations? for all the above

Problem 3. Complete all of the following points. (a) Prove that every PRG is also a OWF. Specifically, suppose G:{0,1}n{0,1}m for m>n is a PRG, prove G is also a OWF. (b) Prove now that not every OWF with m>n is a PRG. Specifically, let f:{0,1}n{0,1}m with m>n be a OWF. Use f to build another function f:{0,1}n{0,1}m+1 which is a OWF but which is not a PRG. Prove that f is a OWF (assuming f is) but is not a PRG. (c) Suppose (Gen, Enc, Dec) is a PKE scheme. Recall that we normally model the key generation procedure Gen() as a randomized procedure which takes no input and which outputs a key pair (pk, sk). Let k be the number of random bits drawn and used by Gen, and let f be the function which takes input r{0,1}k, and runs Gen() with randomness r, obtaining (pk, sk), then f outputs pk. Prove that f is a OWF assuming the semantic security of (Gen, Enc, Dec). Remark: This proves that for PKE to exist, OWF must exist. It is possible to argue along similar lines and use the encryption procedure in any encryption scheme (PKE or SKE) to build a OWF. Thus, even for SKE to exist, OWF must exist