Question: The following ODE models the motion of a damped pendulum with forcing. y (t) + 5y' (t) + 6y(t) = 3 cost, y(0) =

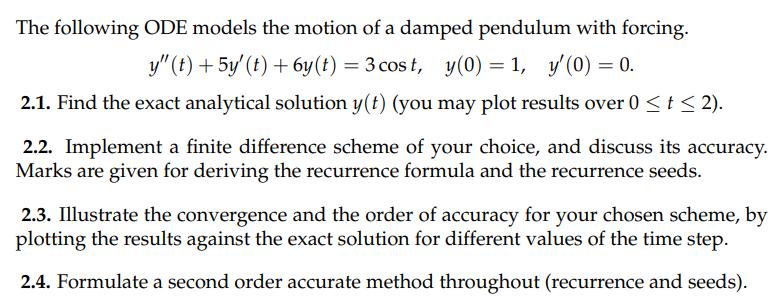
The following ODE models the motion of a damped pendulum with forcing.

The following ODE models the motion of a damped pendulum with forcing. y" (t) + 5y' (t) + 6y(t) = 3 cost, y(0) = 1, y'(0) = 0. 2.1. Find the exact analytical solution y(t) (you may plot results over 0 < t 2). 2.2. Implement a finite difference scheme of your choice, and discuss its accuracy. Marks are given for deriving the recurrence formula and the recurrence seeds. 2.3. Illustrate the convergence and the order of accuracy for your chosen scheme, by plotting the results against the exact solution for different values of the time step. 2.4. Formulate a second order accurate method throughout (recurrence and seeds).

Step by Step Solution

★★★★★

3.47 Rating (163 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

Solution Giv... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Can you please solve the below question (Task 1)? Task 1: Numerical Solution of Differential Equations Solve the equations, dy dx dy dx = 2 sin(x) + y, y(0) = 1 = x + 3x + 2-2y, y(0) = -1 using...

Please solve the below question in 4 to 5 hours it will be a big help please solve this question either using Matlab or Microsoft Excel Please solve this question in Matlab or In Microsoft Excel...

please solve the below question dy Find at t = 4 when y = 5x? x and r dt - Vt. dy 0.000 dt It-4 Round to three decimal places if necessary

This part of the question concerns the quadratic equation 4x 2 + 6x + 2 = 0. (i) Find the discriminant of the quadratic expression 4x 2 + 6x + 2. (ii) What does this discriminant tell you about the...

Is electromagnetic radiation from phone antennae associated with declining bird populations? This is one of the questions addressed by the authors of the paper The Urban Decline of the House Sparrow...

Write a method called reverseHalf that accepts a queue of integers as a parameter and reverses the order of all the elements in oddnumbered positions (position 1, 3, 5, etc.), assuming that the first...

Consider the thick slab of copper in Example 5.10, which is initially at a uniform temperature of 20oC and is suddenly exposed to a net radiant flux of 3 X 105 W/m2. Use the Finite-Difference...

The motion of a damped spring-mass system (Figure) is described by the following ordinary differential equation: where x = displacement from equilibrium position (m), t = time (s), m = 20-kg mass,...

The following ordinary differential equation describes the motion of a damped spring-mess system (Figure): where x = displacement from the equilibrium position, t = time, m = 1 kg mass, and = 5...

Kathleen Taylor is a freshman at Roanoke College, and she wants to develop her schedule for the spring semester. Courses are offered with class periods either on Monday and Wednesday or Tuesday and...

Simplify: (15). (2x)(3y) (16). (-3a 2 b 4 )(-4a 5 b) (17). (-2y 4 x 3 ) 3 (x 4 y)

A one-month budget allowance of factory overhead for the Fabrication Department of the Andromeda Company is as follows: Activity Base: 1,800 machine hours per month at normal capacity, which is 90%...

Use Simpson's rule with n = 4 to approximate the solution to part b at x = . 5 to three decimal places. please include all steps, will upvote. Thank you.

Market-share and market-size variances (continuation of 14-32). Chicago Infonautics senior vice president of marketing prepared his budget at the beginning of the third quarter assuming a 25% market...

Giordanos Electronics issues a $600,000, 8%, 10-year mortgage note on December 31, 2012, to help finance a plant expansion program. The terms provide for semiannual installment payments, not...

Selected worksheet data for Nicholson Company are presented below. Instructions(a) Fill in the missing amounts.(b) Prepare the adjusting entries that weremade. Adjusted Trial Balance Account Titles...

Disposal of assets answers the following questions. 1. A company has an inventory of 1,000 assorted parts for a line of missiles that has been discontinued. The inventory cost is $75,000. The parts...

Relevant and irrelevant costs. Answer the following questions. 1. Dalton computers makes 5,000 units of a circuit board, CB76 at a cost of $230 each. Variable cost pa? unit is $180 and fixed cost per...

What did you find most interesting in the chapter Unintended Consequences in Critical Issues in Crime and Justice: Thought, Policy, and Practice Edition: Third Edition By: Dan Okada, Mary Maguire, &...

DIFFERENTIAL EQUATION MATH Will rate for good work! Very appreciate! The extra credit project is to teach me about an differential equation that pops up in a field of interest, and then do the...

I need detailed solution to these. FINANCIAL CALCULUS (ii) I B = 0, and C(1,) = A(1,1)e-B(t,7), then show that A(1,7) and B(1,T) are solutions of the following ODE of boundary value problem:...

Using your preferred code editor (e.g. VSCode), in a Python script called " CR3.py ", write code to answer the following problem. Problem Consider the following ODE, describing the displacement u(t)...

Q4. Solve the following ODE. d'y dy dy -0 dx dx dx Q5. Find the particular solution of the following 2md order ODE: dy d'y -3+2y 4e2* sin x, dx dy dx 9 = dx. dx lo y(0) = 6, %3D

Help Question 2 (a) Check the exactness of the following ODE and solve accordingly: (x4 + y?) dx - xy dy = 0, y(2) = 1 (a) Solve the following ODE: x2y" + 3xy' + 0.75y = 0, y(1) = 1 and y'(

Graph particular solutions of the following ODE, proceeding as explained. (21) y cos x dx + 1/y dy = 0. (a) Test for exactness. If necessary, find an integrating factor. Find the general solution...