Question: Let u = u(x, y). (a) Consider the P.D.E. 0= y'u-2xyu +x'u, + yu, + xu, . Identify the equation type (hyperbolic, parabolic or

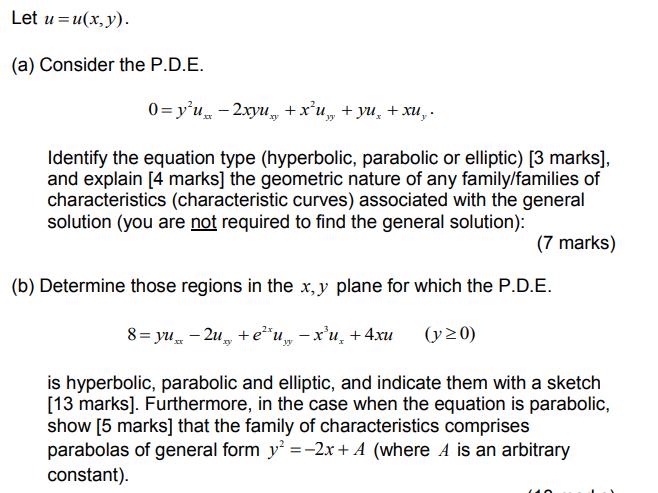
Let u = u(x, y). (a) Consider the P.D.E. 0= y'u-2xyu +x'u,

Let u = u(x, y). (a) Consider the P.D.E. 0= y'u-2xyu +x'u, + yu, + xu, . Identify the equation type (hyperbolic, parabolic or elliptic) [3 marks], and explain [4 marks] the geometric nature of any family/families of characteristics (characteristic curves) associated with the general solution (you are not required to find the general solution): (7 marks) (b) Determine those regions in the x, y plane for which the P.D.E. 8= yu - 2u, +e*u, -x'u, + 4xu (y20) is hyperbolic, parabolic and elliptic, and indicate them with a sketch [13 marks]. Furthermore, in the case when the equation is parabolic, show [5 marks] that the family of characteristics comprises parabolas of general form y =-2x+ A (where A is an arbitrary constant).

Step by Step Solution

★★★★★

3.42 Rating (161 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Write a report on Saga. You are not required to read the entire saga although it may help you to skim the plot. You may focus on any aspects which interest you in your report but must include a brief...

Governments are not required to accrue interest on long-term debt in governmental funds even if the interest is applicable to a current period and will be due the rst day of the following year....

Inexhaustible collections of ONPOs are not required to be capitalized or depreciated, if certain criteria are met. Why is this so, and what accounting and reporting recognition, if any, is required...

Let d be a dummy (binary) variable and let z be a quantitative variable. Consider the model y = (0 + (0d + (1z + (1d (z + u; this is a general version of a model with an interaction between a dummy...

Derive the Greens method solution for the response caused by an arbitrary forcing function. Consider the function to consist of a series of step functions-that is, start from Equation 3.105 rather...

Consider the simple regression model Y = 0 + 1 x + u and let z be a binary instrumental variable for x. Use (15.10) to show that the IV estimator 1, can be written as where 0 and 0 are the sample...

Consider the PDE: fx + 0.2fy = 0 with X [0, 1] and Y [0, 1]. (a) What is the unknown in this equation? (b) Explain this equation using plain English. (c) How many functions f (x, y) can you find...

Recall that the Fourier transform of x(t) = e j0 is X(j) = 2( 0 ) and the Fourier transform of: (a) Determine the Fourier transform Y( j ) of Y( t ) = p( t ) e j0t And roughly sketch |Y(j)| versus...

Let H be a Wiener process. Consider the geometric process S again: (a) Calculate dS t . (b) What is the ?expected rate of change? of S t ? (c) If the exponential term in the definition of S t , did...

Financial institutions have a legal and social responsibility to serve all communities. Do banks adequately serve both inner-city and suburban neighborhoods, both poor and wealthy communities? In New...

Let A = ? sin ? ap + ?2 a?. Evaluate ?L A ? dl given that(a) L is the contour of Figure (a)(b) L is the contour of Figure (b) (a) (b)

Chromium plating in this environment is observed to remain bright with no apparent oxide tarnish. Does this observation violate thermodynamic principles? Explainn

Find the Taylor series for f ( x ) centered at the given val R n ( x ) 0 . 1 f ( x ) = l n ( x ) , a = 2 f ) Find the Issociated radius of convergence, R . R =

From the following data, prepare in proper form an income statement showing departmental gross profit (assume a 30% tax rate) for Accelerated Stop for the year ended December 31, 201X: Cash...

On December 1, 2014, the Iaia Wholesale Co. is attempting to project cash receipts and disbursements through January 31, 2015. On this latter date, a note will be payable in the amount of $ 107,000....

Depletion and DepreciationMining Khamsah Mining Company has purchased a tract of mineral land for $900,000. It is estimated that this tract will yield 120,000 tons of ore with sufficient mineral...

Doan Company requested that you (1) Assign indirect expenses to its jewelry and shoes departments as appropriate (2) Prepare an income statement for July 201X showing departmental contribution margin...

Equipment with a cost of $160,000 has an estimated residual value of $10,000 and an estimated life of 5 years or 12,000 hours. It is to be depreciated by the straight-line method. What is the amount...

One common community-acquired infection is influenza (flu). It is caused by the influenza virus, which is a virus (microorganism). The usual reservoir is infected humans. The portal of exit is...

9. Which of the following outlines the most efficient process to evaluate / sin r cos' xdx? A. Split off cos x, use trig identity on remaining cosines, and let u = cos x B. Split off sin x, use trig...

1. Let X and Y be independent Geometric random variables, i.e., P(X = k) = P(Y = k) = (1 -p)pk, k = 0, 1, . ... Let U = min {X, Y}, V = max {X, Y } and W = V - U. (a) Compute P(U = i) and P(W = j)...

Let $u:[a, b] ightarrow \mathbb{R}$ be a continuous function. Show that $x \mapsto \int_{[a, x]} u(t) d t$ is everywhere differentiable and find its derivative. What happens if we assume only...

1) (From Hardcover Book, Marsden/Tromba, Vector Calculus, 6th ed., Section 1.1., # 37 or from your Ebook in the Supplementary Exercises for Section 11.1, #42) Find a line that lies entirely in the...

Let X and Y be random variables such that Var(X), Var(Y ) and Cov(X, Y ) exist, and let U = a X + bY and V = cX + dY with a, b, c, d constants. Find an expression for Cov(U, V) in terms of a, b, c,...

1 Evaluate dx. ac + x(In x 2 Let u = In x and note that du = dx. Thus, 1 x +x(In x 2 dx = 1 + (In x) 2 da - J du = =0 +