Capillary rise in a tube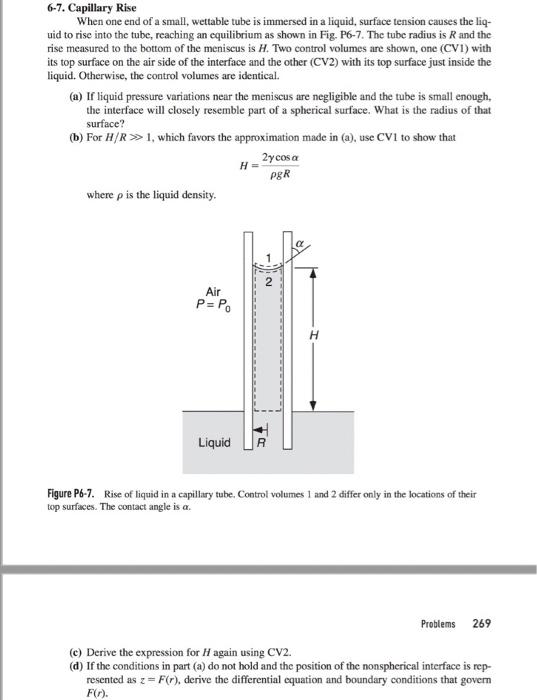

6-7. Capillary Rise When one end of a small, wettable tube is immersed in a liquid, surface tension causes the liquid to rise into the tube, reaching an equilibrium as shown in Fig. P6-7. The tube radius is R and the rise measured to the bottom of the meniscus is H. Two control volumes are shown, one (CVI) with its top surface on the air side of the interface and the other (CV2) with its top surface just inside the liquid. Otherwise, the control volumes are identical. (a) If liquid pressure variations near the meniscus are negligible and the tube is small enough, the interface will closely resemble part of a spherical surface. What is the radius of that surface? (b) For H/R1, which favors the approximation made in (a), use CV1 to show that H=gR2cos where is the liquid density. Figure P6-7. Rise of liquid in a capillary tube. Control volumes 1 and 2 differ only in the locations of their top surfaces. The contact angle is . Problems 269 (c) Derive the expression for H again using CV2. (d) If the conditions in part (a) do not hold and the position of the nonspherical interface is represented as z=F(r), derive the differential equation and boundary conditions that govern F(r). 6-7. Capillary Rise When one end of a small, wettable tube is immersed in a liquid, surface tension causes the liquid to rise into the tube, reaching an equilibrium as shown in Fig. P6-7. The tube radius is R and the rise measured to the bottom of the meniscus is H. Two control volumes are shown, one (CVI) with its top surface on the air side of the interface and the other (CV2) with its top surface just inside the liquid. Otherwise, the control volumes are identical. (a) If liquid pressure variations near the meniscus are negligible and the tube is small enough, the interface will closely resemble part of a spherical surface. What is the radius of that surface? (b) For H/R1, which favors the approximation made in (a), use CV1 to show that H=gR2cos where is the liquid density. Figure P6-7. Rise of liquid in a capillary tube. Control volumes 1 and 2 differ only in the locations of their top surfaces. The contact angle is . Problems 269 (c) Derive the expression for H again using CV2. (d) If the conditions in part (a) do not hold and the position of the nonspherical interface is represented as z=F(r), derive the differential equation and boundary conditions that govern F(r)