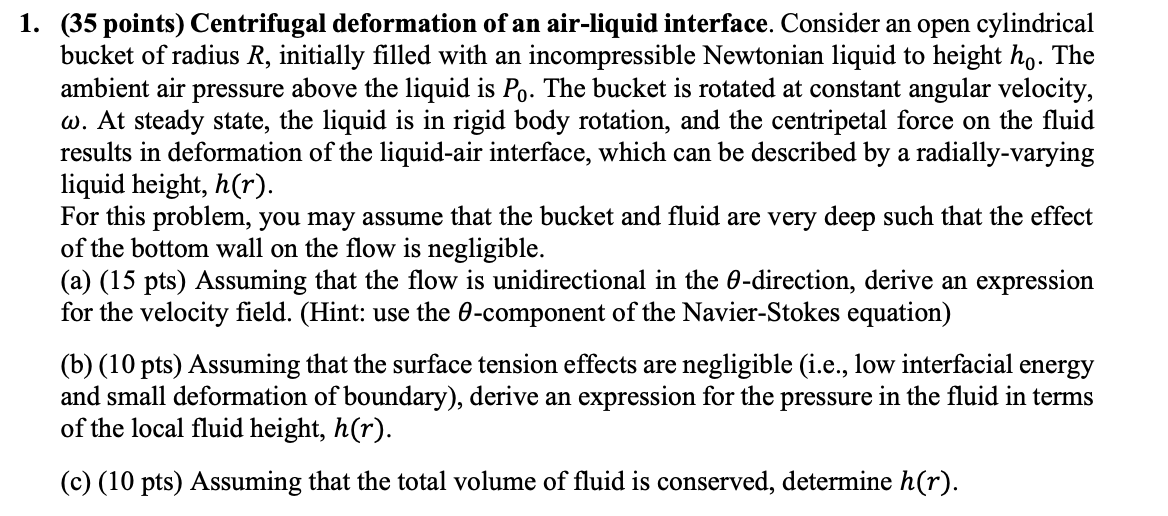
Centrifugal deformation of an air$-$liquid interface. Consider an open cylindrical

bucket of radius R$,$ initially filled with an incompressible Newtonian liquid to height $0.$ The

ambient air pressure above the liquid is $0.$ The bucket is rotated at constant angular velocity,

$.$ At steady state, the liquid is in rigid body rotation, and the centripetal force on the fluid

results in deformation of the liquid$-$air interface, which can be described by a radially$-$varying

liquid height, $().$

For this problem, you may assume that the bucket and fluid are very deep such that the effect

of the bottom wall on the flow is negligible.

$($a$)(15$ pts$)$ Assuming that the flow is unidirectional in the $-$direction, derive an expression

for the velocity field. $($Hint: use the $-$component of the Navier$-$Stokes equation$)$

$($b$)(10$ pts$)$ Assuming that the surface tension effects are negligible $($i$.$e$.,$ low interfacial energy

and small deformation of boundary$),$ derive an expression for the pressure in the fluid in terms

of the local fluid height, $().$

$($c$)(10$ pts$)$ Assuming that the total volume of fluid is conserved, determine $().(35$ points$)$ Centrifugal deformation of an air$-$liquid interface. Consider an open cylindrical

bucket of radius $R,$ initially filled with an incompressible Newtonian liquid to height $h_0.$ The

ambient air pressure above the liquid is $P_0.$ The bucket is rotated at constant angular velocity,

$.$ At steady state, the liquid is in rigid body rotation, and the centripetal force on the fluid

results in deformation of the liquid$-$air interface, which can be described by a radially$-$varying

liquid height, $h(r).$

For this problem, you may assume that the bucket and fluid are very deep such that the effect

of the bottom wall on the flow is negligible.

$($a$)(15$ pts$)$ Assuming that the flow is unidirectional in the $-$direction, derive an expression

for the velocity field. $($Hint: use the $-$component of the Navier$-$Stokes equation$)$

$($b$)(10$ pts$)$ Assuming that the surface tension effects are negligible $($i$.$e$.,$ low interfacial energy

and small deformation of boundary$),$ derive an expression for the pressure in the fluid in terms

of the local fluid height, $h(r).$

$($c$)(10$ pts$)$ Assuming that the total volume of fluid is conserved, determine $h(r).$