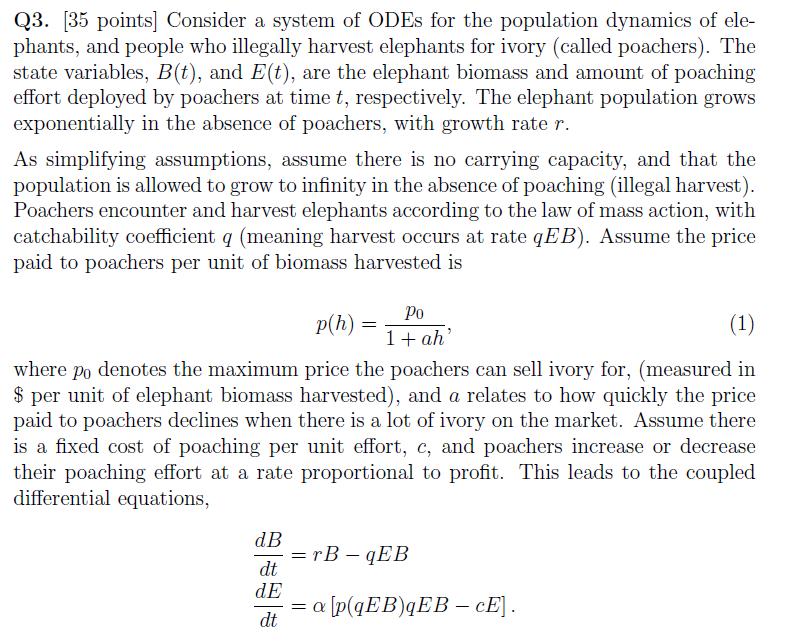
Q3. [35 points] Consider a system of ODEs for the population dynamics of ele- phants, and people who illegally harvest elephants for ivory (called poachers). The state variables, B(t), and E(t), are the elephant biomass and amount of poaching effort deployed by poachers at time t, respectively. The elephant population grows exponentially in the absence of poachers, with growth rate r. As simplifying assumptions, assume there is no carrying capacity, and that the population is allowed to grow to infinity in the absence of poaching (illegal harvest). Poachers encounter and harvest elephants according to the law of mass action, with catchability coefficient q (meaning harvest occurs at rate qEB). Assume the price paid to poachers per unit of biomass harvested is (1) where po denotes the maximum price the poachers can sell ivory for, (measured in $ per unit of elephant biomass harvested), and a relates to how quickly the price paid to poachers declines when there is a lot of ivory on the market. Assume there is a fixed cost of poaching per unit effort, c, and poachers increase or decrease their poaching effort at a rate proportional to profit. This leads to the coupled differential equations, dB dt dE dt = p(h): = Po 1+ ah' = rBqEB a [p(qEB)qEB - CE].