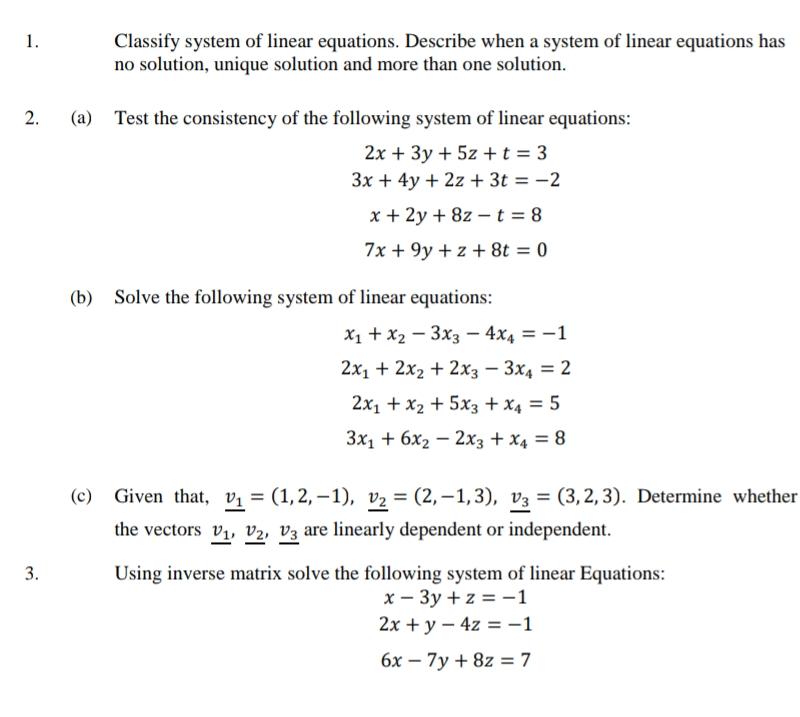
Classify system of linear equations. Describe when a system of linear equations has no solution, unique solution and more than one solution. 1. Test the consistency of the following system of linear equations: 2x + 3y + 5z +t = 3 3x + 4y + 2z + 3t = -2 x + 2y + 8z t = 8 7x + 9y + z + 8t = 0 (b) Solve the following system of linear equations: X1 + x2 - 3x3 - 4x4 = -1 2x1 + 2x2 + 2x3 - 3x4 = 2 2x1 + x2 + 5x3 + x4 = 5 3x1 + 6x2 2x3 + X4 = 8 Given that, v = (1,2, 1), v2 = (2,-1,3), v3 = (3,2, 3). Determine whether the vectors v1, v2, Vz are linearly dependent or independent. 3. Using inverse matrix solve the following system of linear Equations: x - 3y + z = -1 2x + y 4z = -1 6 7 + 82 3 7 2.