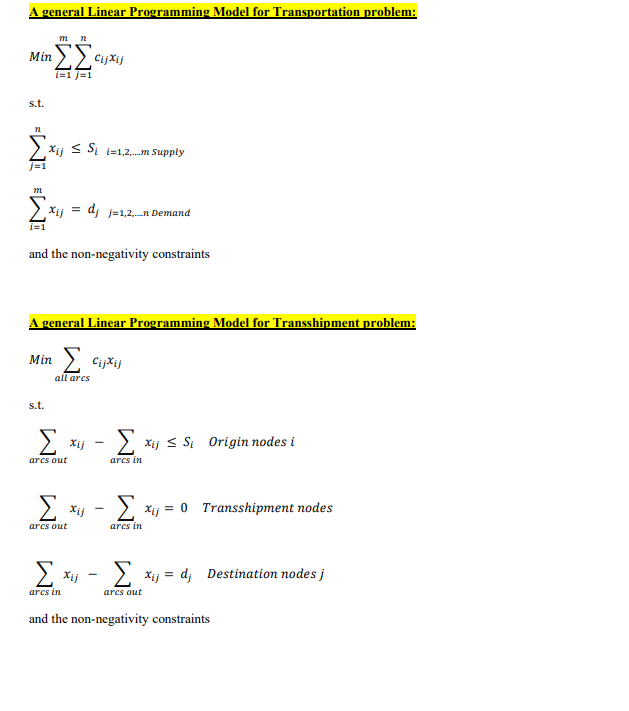
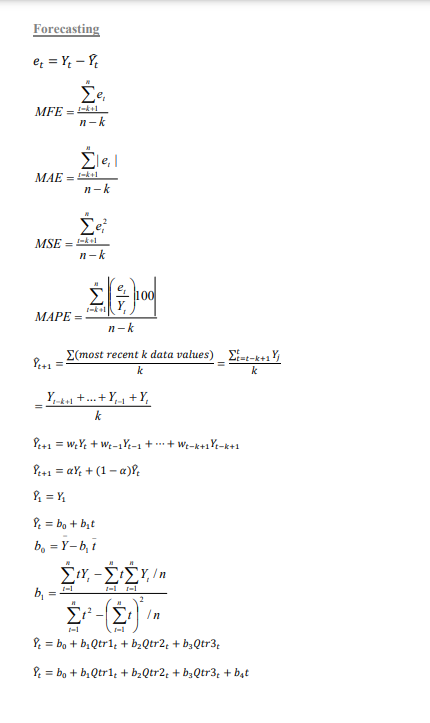
Clearly specify all the constraints such that only constants appear on the right-hand sides of constraints. Only use the variables defined in the problem. Use of any other variable can lead to a reduction in marks. Atkinson Apple Products (AAP) owns orchards in the El Dorado region where it grows apples. It then ships these apples to its three processing facilities in Appleton (A), Broadway (B) and Columbus (C) where a variety of apple products are manufactured. Recently, as the economy has improved, AAP has experienced a growth in its product demand so it wants to purchase one or more orchards to produce more apple products. AAP is considering six new orchards. Following binary variables are defined. Yi = 1 if orchard 'i' is purchased, 0 if not where i=1, 2, 3, 4, 5, 6 They are looking your help in adding constraint's for the LP formulation with following restrictions; answer each part independently. (II-a) Write a single constraint that if orchard-3 or orchard-5 or both are purchased, then they must also purchase orchard-2. (II-b) Write a single constraint that if orchard-1 is purchased, then they must also purchase orchard- 2 or orchard-3 or both. (II-c) Write a single constraint that if orchard-1 is purchased, then they must also purchase orchard- 4 and orchard-5. (II-d) Write a single constraint that orchard-4 can only be purchased if orchard-3 is purchased. A general Linear Programming Model for Transportation problem: Min mn i=1 j=1 s.t. IM IM- XS i=1,2m Supply = Xijd j=1,2n Demand and the non-negativity constraints A general Linear Programming Model for Transshipment problem: Min Cijij s.t. all arcs arcs out arcs out - xij S Origin nodes i - arcs in x) = 0 Transshipment nodes arcs in x() x = d; Destination nodes j arcs in arcs out and the non-negativity constraints A general Linear Programming Model for Assignment problem: m n in s.t. i=1 j=1 n j=1 WW xij 1 -1,2...m Agents m i=1 xj1j=1,2n Tasks and the non-negativity constraints A general Linear Programming Model for Shortest-Route problem: Min Cixi s.t. all arcs x = 1 Origin node i arcs out arcs out xij - - x() = 0 Transshipment nodes arcs in x) = 1 Destination node j arcs in and the non-negativity constraints Simulation: probabilistic inputs follow a uniform distribution Probabilistic input = a + r(b-a) where: a=smallest value, b=largest value, -random number. A general Linear Programming Model for Assignment problem: m n in s.t. i=1 j=1 n j=1 WW xij 1 -1,2...m Agents m i=1 xj1j=1,2n Tasks and the non-negativity constraints A general Linear Programming Model for Shortest-Route problem: Min Cixi s.t. all arcs x = 1 Origin node i arcs out arcs out xij - - x() = 0 Transshipment nodes arcs in x) = 1 Destination node j arcs in and the non-negativity constraints Simulation: probabilistic inputs follow a uniform distribution Probabilistic input = a + r(b-a) where: a=smallest value, b=largest value, -random number. Forecasting e = Y- MFE = Inkel n-k MAE n-k MSE n-k MAPE= n-k 82+1 (most recent k data values)t-k+1 k +...+Y+Y Y-+1+...+ k == k Pt+1 = We Ye + We-1-1 + + We-k+11-k+1 +1 =Y + (1-)& b =Y-bt b = - 1-1 2 1-1 - 10 1-1 - = bo + bQtr + bQtr2; + b3Qtr3 = bo + bQtr + bQtr2 + b3Qtr3 + bat