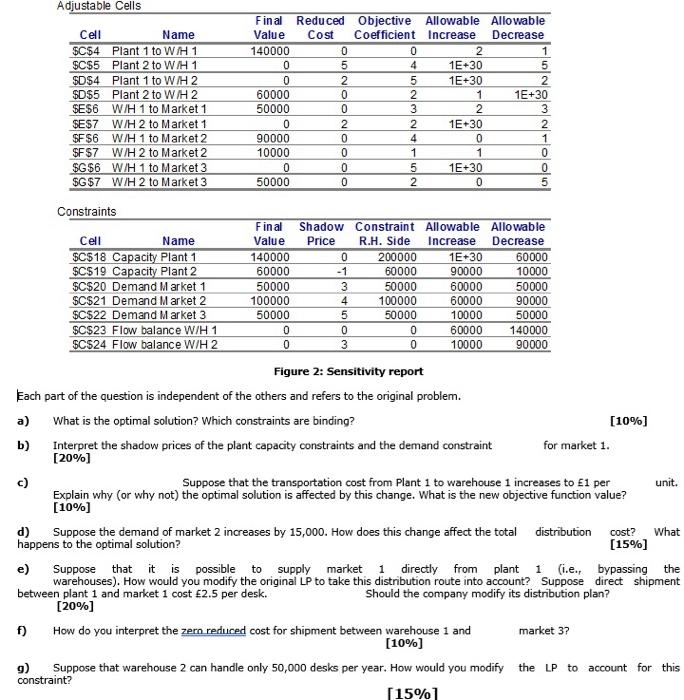
Company X produces office desks at two plants. Plant P1 has an annual capacity of 200,000 desks; plant P2 has an annual capacity of only 60,000 desks. There are three different market areas for desks. Each market requires at least the following shipments: market 1: 50,000 desks per year, market 2: 100,000 desks per year, and market 3: 50,000 desks per year. Desks can be shipped from the plants to the markets via two warehouses. The distribution cost per desk between plants and warehouses, and between warehouses and markets are shown in Table 1. (Note: in the Table means that this distribution route is not relevant or not possible at the moment.) Table 1: Distribution cost ( per desk) between plants and warehouses (W/H) and between warehouses and markets To: From: P1 P2 W/H 1 W/H 2 W/H 1 W/H 2 521 Market 1 Market 2 Market 3 51 The company's problem is to find out how many desks to produce at each plant, and how to ship the desks to the markets such that the total distribution cost is minimised. A linear programming model was formulated the Excel layout (with optimal solution) and the Solver sensitivity report are shown in Figure 1 and Figure 2. From: Plant 1 To: Warehouse 1 Warehouse 2 Market 1 Market 2 Market 3 Total flow out 140000 0 140000 Plant 2 0 60000 60000 Warehouse 1 50000 90000 140000 Warehouse 2 0 10000 50000 60000 Total flow in 140000 60000 50000 100000 50000 Unit cost: Plant 1 Warehouse 1 Warehouse 2 Market 1 Market 2 Market 3 0 Plant 2 4 Warehouse 1 Warehouse 2 5 2 TINGE - 132 1162 5 Constraints Ihs sign rhs Capacity Plant 1 140000 200000 Capacity Plant 2 60000 60000 Demand Market 1 50000 Demand Market 2 100000 Demand Market 3 50000 = < Flow balance W/H 1 Flow balance W/H 2 0 50000 100000 50000 0 0 Total cost- 740000 140000 0 0 60000 50000 0 W/H 1 to Market 2 90000 W/H 2 to Market 2 10000 Adjustable Cells Cell Name $C$4 Plant 1 to W/H 1 $C$5 Plant 2 to W/H 1 $D$4 Plant 1 to W/H2 $D$5 Plant 2 to W/H 2 $E$6 W/H 1 to Market 1 SE$7 W/H 2 to Market 1 $F$6 SF $7 Final Reduced Objective Allowable Allowable Value Cost Coefficient Increase Decrease 0 0 2 1 5 4 1E+30 5 2 5 1E+30 2 0 2 1 1E+30 0 3 2 3 2 2 1E+30 2 0 4 0 1 0 1 1 0 $G$6 W/H 1 to Market 3 0 0 5 1E+30 0 $G$7 W/H 2 to Market 3 50000 0 2 0 5 Constraints Final Shadow Constraint Allowable Allowable Cell Name Value Price R.H. Side Increase Decrease $C$18 Capacity Plant 1 140000 0 200000 1E+30 60000 $C$19 Capacity Plant 2 60000 -1 60000 90000 10000 $C$20 Demand Market 1 50000 3 50000 60000 50000 $C$21 Demand Market 2 100000 4 100000 60000 90000 $C$22 Demand Market 3 50000 5 50000 10000 50000 $C$23 Flow balance W/H 1 0 0 0 60000 140000 $C$24 Flow balance W/H 2 0 3 0 10000 90000 Figure 2: Sensitivity report Each part of the question is independent of the others and refers to the original problem. a) What is the optimal solution? Which constraints are binding? b) Interpret the shadow prices of the plant capacity constraints and the demand constraint [20%] [10%] for market 1. c) Suppose that the transportation cost from Plant 1 to warehouse 1 increases to 1 per Explain why (or why not) the optimal solution is affected by this change. What is the new objective function value? [10%] unit. d) Suppose the demand of market 2 increases by 15,000. How does this change affect the total distribution happens to the optimal solution? cost? What [15%] bypassing the e) Suppose that it is possible to supply market 1 directly from plant 1 (i.e., warehouses). How would you modify the original LP to take this distribution route into account? Suppose direct shipment. between plant 1 and market 1 cost 2.5 per desk. Should the company modify its distribution plan? f) g) [20%] How do you interpret the zern reduced cost for shipment between warehouse 1 and [10%] market 3? Suppose that warehouse 2 can handle only 50,000 desks per year. How would you modify the LP to account for this constraint? [15%]