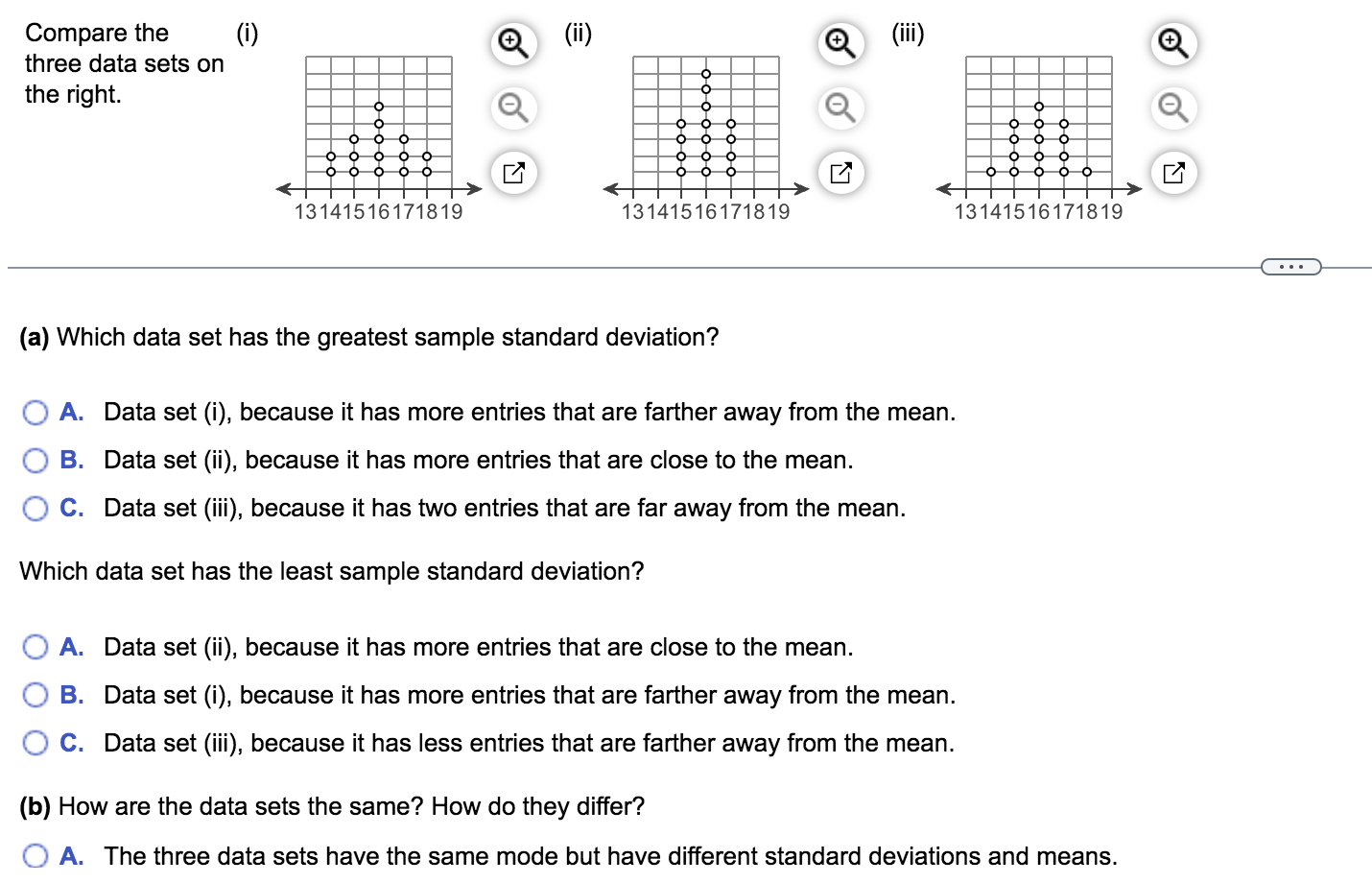
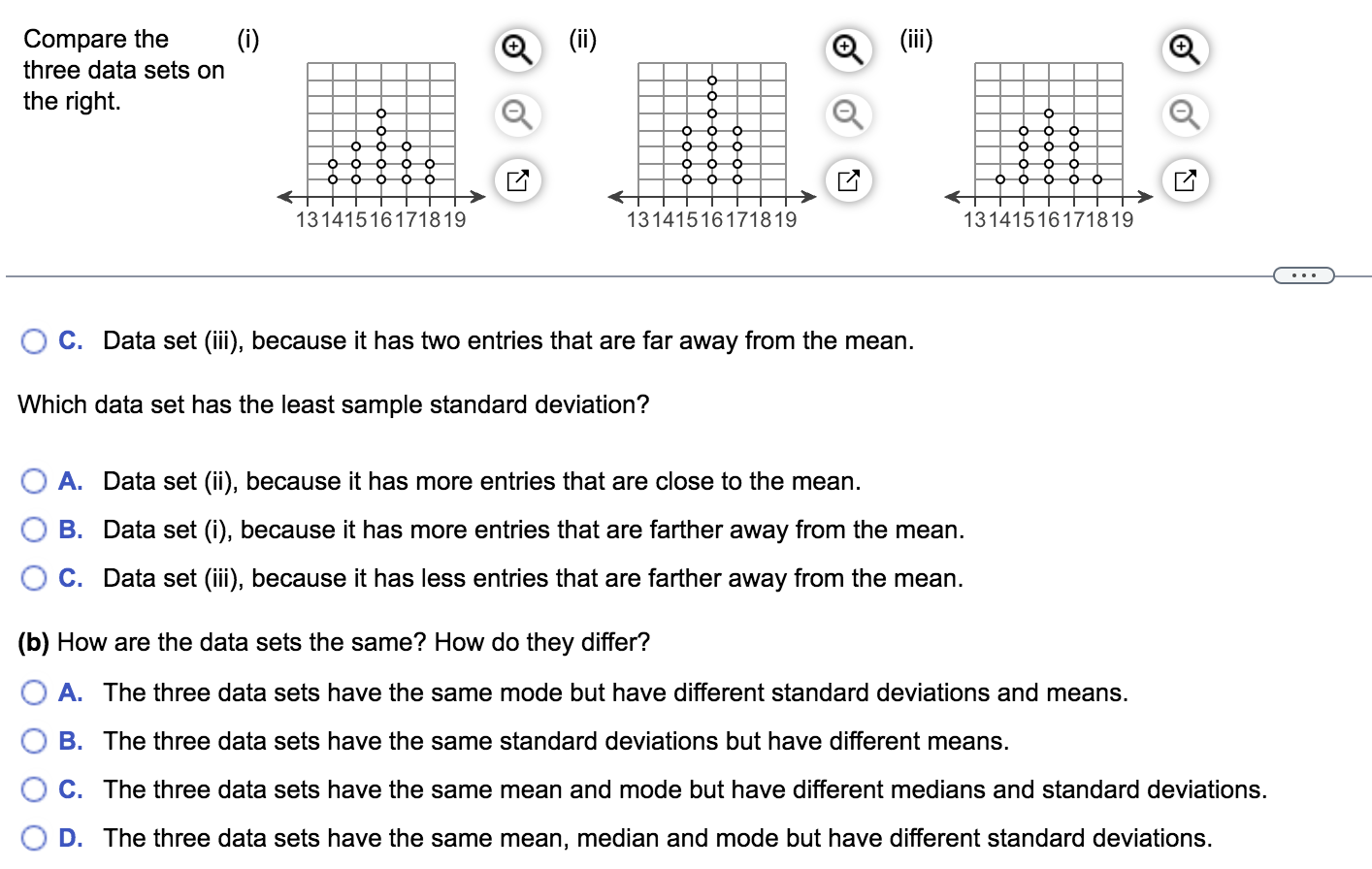
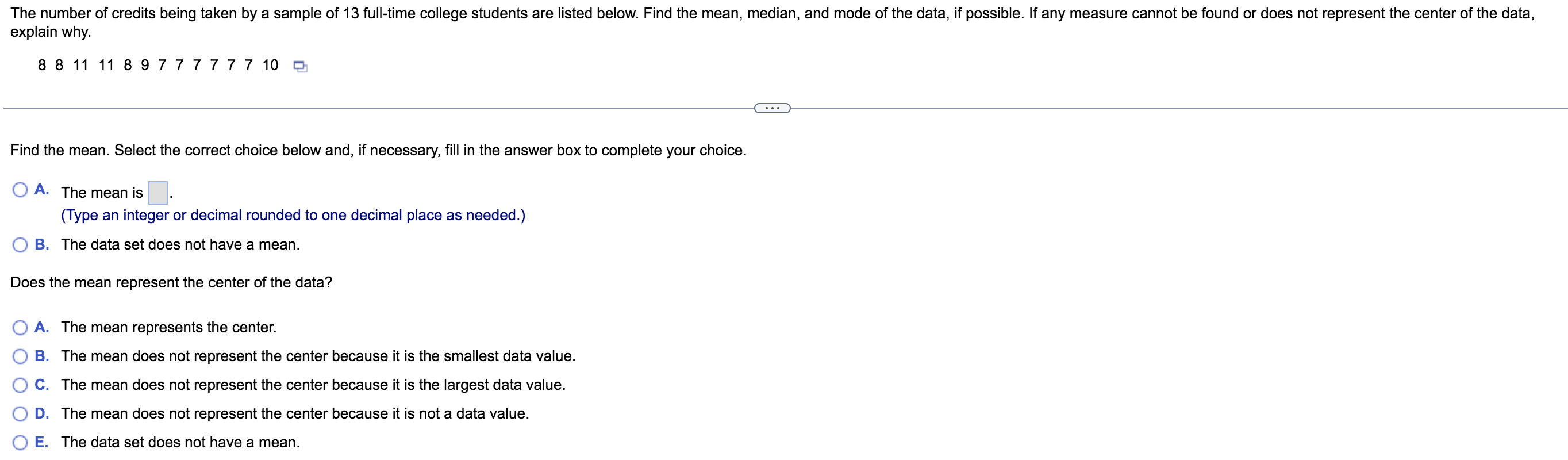
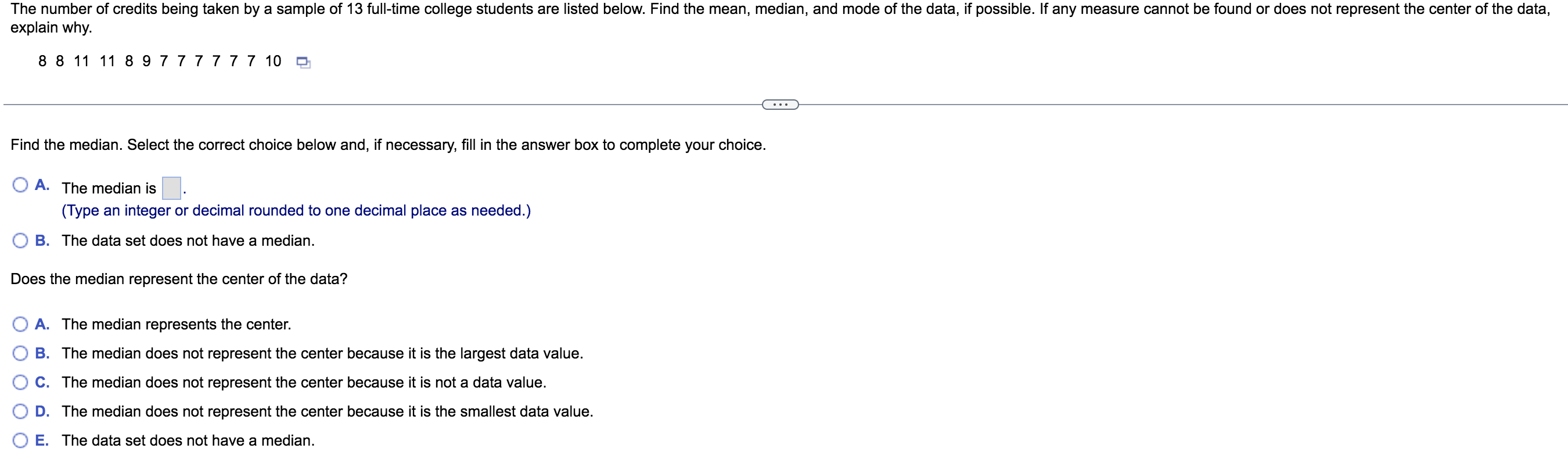
Compare the three data sets on the right. (i) 13141516171819 (ii) Q (iii) 13141516171819 13141516171819 (a) Which data set has the greatest sample standard deviation? A. Data set (i), because it has more entries that are farther away from the mean. B. Data set (ii), because it has more entries that are close to the mean. C. Data set (iii), because it has two entries that are far away from the mean. Which data set has the least sample standard deviation? A. Data set (ii), because it has more entries that are close to the mean. B. Data set (i), because it has more entries that are farther away from the mean. C. Data set (iii), because it has less entries that are farther away from the mean. (b) How are the data sets the same? How do they differ? OA. The three data sets have the same mode but have different standard deviations and means. Compare the three data sets on the right. (i) 13141516171819 (ii) 13141516171819 (iii) 13141516171819 C. Data set (iii), because it has two entries that are far away from the mean. Which data set has the least sample standard deviation? A. Data set (ii), because it has more entries that are close to the mean. B. Data set (i), because it has more entries that are farther away from the mean. C. Data set (iii), because it has less entries that are farther away from the mean. (b) How are the data sets the same? How do they differ? A. The three data sets have the same mode but have different standard deviations and means. B. The three data sets have the same standard deviations but have different means. C. The three data sets have the same mean and mode but have different medians and standard deviations. D. The three data sets have the same mean, median and mode but have different standard deviations. The state test scores for 12 randomly selected high school seniors are shown on the right. Complete parts (a) through (c) below. Assume the population is normally distributed. (a) Find the sample mean. x = (Round to one decimal place as needed.) (b) Find the sample standard deviation. S= (Round to one decimal place as needed.) (c) Construct a 99% confidence interval for the population mean . A 99% confidence interval for the population mean is ( , ). (Round to one decimal place as needed.) ... 1426 1223 990 695 722 830 721 746 541 627 1442 949 The number of credits being taken by a sample of 13 full-time college students are listed below. Find the mean, median, and mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. 88 11 11 8 9 7 7 7 7 7 7 10 Find the mean. Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. The mean is (Type an integer or decimal rounded to one decimal place as needed.) B. The data set does not have a mean. Does the mean represent the center of the data? A. The mean represents the center. B. The mean does not represent the center because it is the smallest data value. C. The mean does not represent the center because it is the largest data value. D. The mean does not represent the center because it is not a data value. E. The data set does not have a mean. The number of credits being taken by a sample of 13 full-time college students are listed below. Find the mean, median, and mode of the data, if possible. If any measure cannot be found or does not represent the center of the data, explain why. 8 8 11 11 8 9 7 7 7 7 7 7 10 Find the median. Select the correct choice below and, if necessary, fill in the answer box to complete your choice. A. The median is (Type an integer or decimal rounded to one decimal place as needed.) B. The data set does not have a median. Does the median represent the center of the data? A. The median represents the center. B. The median does not represent the center because it is the largest data value. C. The median does not represent the center because it is not a data value. D. The median does not represent the center because it is the smallest data value. E. The data set does not have a median.