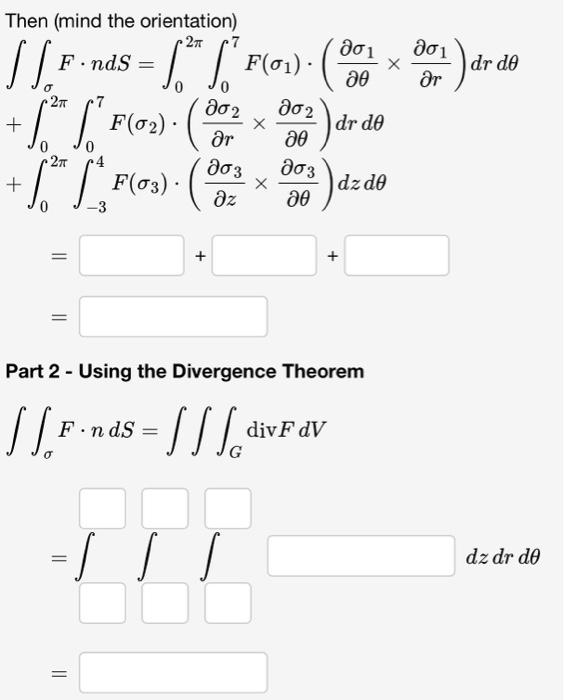
Compute the outward flux of the vector field F(x, y, z) = 5xi + 3yj +5ek across the boundary of the right cylinder with radius 7 with bottom edge at height z = -3 and upper edge at z = 4. Note: The vectors in this field point outwards from the origin, so we would expect the flux across each face of the cylinder to be positive. Part 1 - Using a Surface Integral First we parameterize the three faces of the cylinder. Note: in the following type as theta. The bottom face: 01(r, 0) = (x(r, 0), y(r,0), z(r, 0) where 0 0 2T, 0 r 7 and x1 (r, 0) = y (r, 0) = z1(r, 0) = y2 (r, 0) The top face: 0 (r, 0) = (x2(r, 0), 2(r, 0), 2(r, 0) where 00 2T, 0r7 and x (r, 0) = 22 (r, 0) == -3 = = 4 The lateral face: 03(0, 2) = (x3(0, 2), y3(0, 2), 23(0, 2) where 00 2, -3 z 4 and x3(0) = Y3 (0) = 23(z) = Then (mind the orientation) [[F.nds - 1 [ F (01) (801 - J P(0). F(02) - (307 2T 202 202 dr de + r 20 0 203 20 2 + - [* *, F(03). ( z 0 -3 = + = X dz de + Part 2 - Using the Divergence Theorem [[F.nas [//di = 1 div F dV 201 r dr de dz dr de