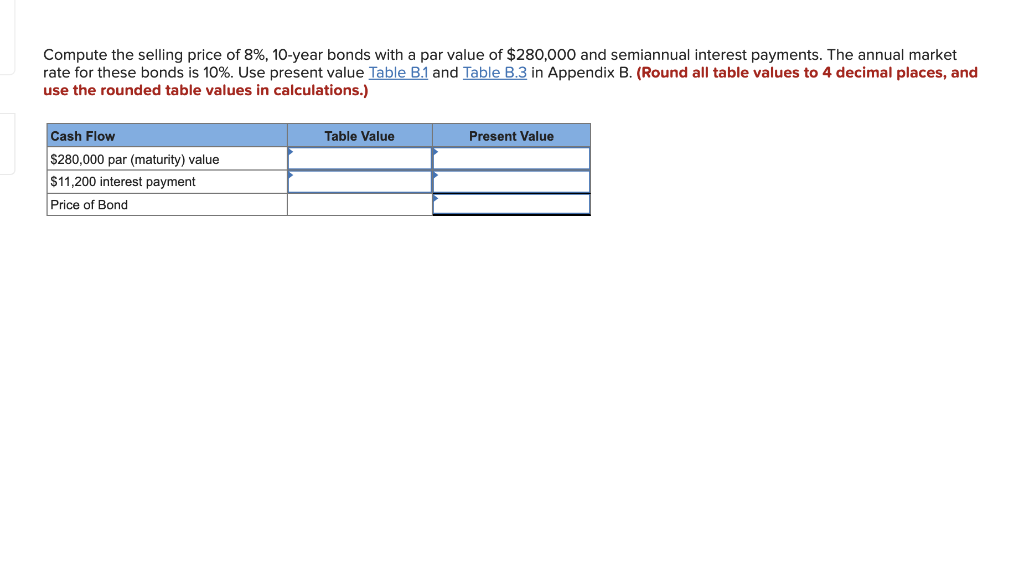
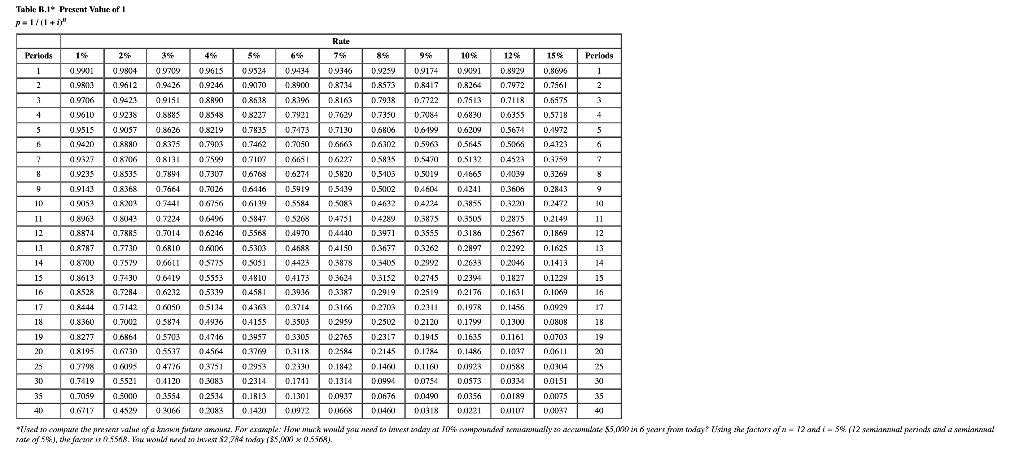
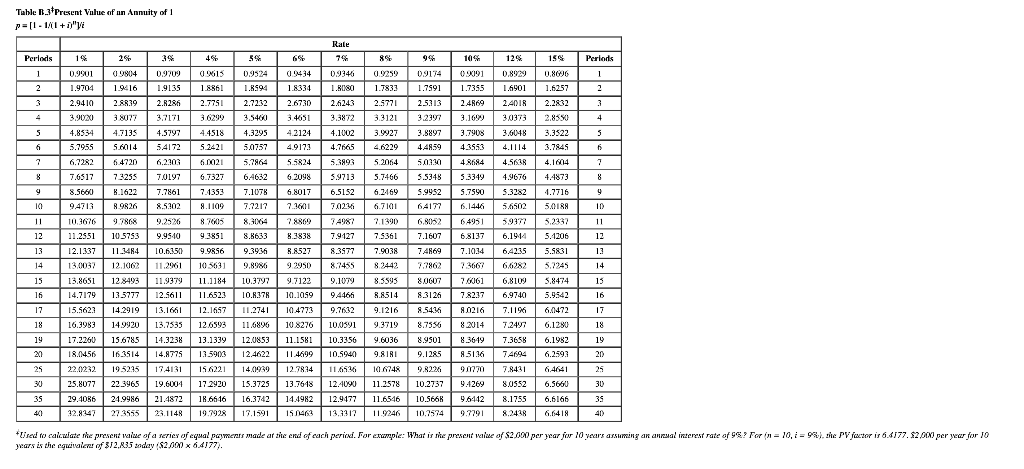
Compute the selling price of 8%, 10-year bonds with a par value of $280,000 and semiannual interest payments. The annual market rate for these bonds is 10%. Use present value Table B.1 and Table B.3 in Appendix B. (Round all table values to 4 decimal places, and use the rounded table values in calculations.) Table Value Present Value Cash Flow $280,000 par (maturity) value $11,200 interest payment Price of Bond Table B.1. Present Value of 1 0.7014 Rute Periods 1% 2% 546 6% 856 946 10% 12% 15% Periods 1 09901 09904 09709 0.9615 0.9524 0.9434 0.9346 0.9259 0.9174 0.9091 0.8929 0.86996 1 2 0.980.3 0.9612 0.9426 0.9246 0.9070 0.8900 0.87.34 08573 0.8417 0.8264 0.7972 0.7561 2 . 0.92016 0.9423 0.9151 0.8R90 0.86.18 0.8106 08163 0.79.18 0.7722 0.7513 0.6575 3 4 010 09238 08885 08548 0.8227 0.7921 0.7629 0.7330 0.7084 0.6830 0.6355 0.5728 4 S 0.9515 0.9057 0.8626 0.8219 0.7835 0.3473 0.7130 0.6806 0.6-19 0.6209 0.5674 0.4972 5 0.9420 QARAD 08375 0.7903 0.7462 0.7050 0.6663 0.6302 0.5963 0.5645 0.5066 04123 6 7 09927 (8206 08121 0.7107 0.6651 06227 0.5835 0.5470 0.5132 04523 0.3759 2 8 09235 0.8535 0.7894 0.7307 0.6768 0.6274 0.5830 0.5103 0.5019 0.1665 0.4039 0.3269 8 0.9143 0.8368 0.7664 0.7026 0.6416 0.5919 05439 05002 0.4604 0.4241 0.3606 0.2843 9 10 09153 (&N 0.3441 06199 0.5584 0.5183 0.3855 0.3220 D.2472 11 11 08963 08043 0.7224 0649 0.5847 0.5268 0.4751 0.4289 0.3875 0.350S 0.2875 0.2149 11 12 0.8374 0.7885 0.6246 0.5568 0.4970 0.4440 0.3971 0.3555 03186 0.2567 0.1869 12 08787 0.77.30 0.600K 0.5103 0.458 041.50 03677 03262 0.2897 0.2292 0.1625 13 14 08700 0.7579 06611 05775 0 5051 0.4423 0.3878 0.3405 0.2992 0.2623 0.2043 0.1413 14 15 0.8613 0.7430 06419 0.5553 0.4810 0:4173 0.3624 0.3152 0.2745 0.2394 0.1827 0.1229 15 0.8528 0.7284 0.62.12 053.19 0.4581 0.39.16 0.3387 0.2919 0.2519 0.2176 0.1631 0.1069 16 17 0.8444 0.7142 O NOSO 0.5114 04160 0.3714 0.3166 0.2211 0.1978 0.1455 0.0929 17 18 0.8360 0.7002 05824 0.4936 0.4135 0.3503 0.2939 0.2502 0.2120 0.1799 0.1300 ) 0.080 18 19 0.8277 0.6861 05703 0.4746 03957 0.3305 0.2765 0.2317 0.1915 0.1635 0.1161 0.0703 19 20: 08195 0.6730 0.5577 0.4504 0.3709 0.3118 0.2145 0.1784 0.14% 0.1037 0.0611 20 25 07798 ONIOS 01 4726 03751 0.2993 0230 0.1842 0.1401 0.110 00923 DA58 D/304 25 30 0.7419 0.552 0.4120 03083 0.2314 0.1711 0.1314 0.040 0.0754 0.0573 0.034 0.0151 35 0.7059 0.5000 0.3554 0.2514 0.1R13 0.1301 0.09.17 0.0576 0.0490 0.0356 0.0189 0.0075 35 40 06717 04529 02166 0.2183 0.1420 011972 006080140000318 00221 0107 00037 401 *Used to compare the presener value of a known future amoure. For example: Jiow muck would you need to invest inday at 10% compound mannelly20 awuudate $5,000 in years from today? Using re factors on-12 andi-58.(12 semiannual rinds and a semiannua! Tote of 59.), the four 100.5568. You would need an invest $2,784 riday 135 x 0.5568). 0.6810 Table 1.3 Present Value of an Annuity of 1 p= [1.1/1 + )" Rale 746 Periods 24 3% 44 5% 88 966 124 158 Periods 0.9709 0.9174 1 1 2 3 0.8989 1.6901 105 09191 1.7355 2.4860 0.866 1.6257 1.7591 2 09804 1.9416 2.8839 3 8077 0.9901 1.9704 2.9410 3.9020 4.8534 5.7955 1.9135 2.R286 3.7171 09259 1.7833 2.5771 3.312 2.2832 3 09346 1.8080 2.6243 3.3872 4.1002 4.7665 2.5313 3.2397 4 3.16 2.4DIR 30373 3.60418 4 5 4.7135 3.8897 4.5797 5.4172 3 3.9927 4.6229 2.8590 3.3522 3.7845 3.7908 4.3553 6 5.6014 4.48.59 4.1114 6 6.7282 6.4720 6.2.101 5.1993 5.3064 5020 48684 4.5678 7 7 8 8 4.1004 4.4873 73255 5.3349 4.9676 7.6517 8.566 7.0197 7.7861 8.5302 5.9713 6.5152 5.7466 6.1469 5.5348 5.9952 8 8 9 9 4.7716 ia 9.4713 7.02 6.7101 5.7590 6.1446 64951 5.3282 54502 59977 5.0188 10 11 10.3676 6.8052 11 8.1622 89826 97868 10.5753 11.4484 12.1062 12 11.2951 7.1607 6.8137 5.2332 5.1206 5.5831 12 0613 0.9524 09434 1.8861 1.6594 1.8334 2.7751 2.72.32 2.6730 36299 3.540 34651 4.4518 4.3295 4.2124 5.242 5.07.57 4.917. 6.1021 5.7864 S.5824 6.7327 6.4632 6.2098 7.1353 7.1078 6.8017 8.1109 7.7217 7.1001 8765 8,304 2 8360 9.3851 8.8633 8.3838 9.9856 9.3936 8.8527 10 3631 9.8986 9 1950 11.1184 10.3792 9.7122 116523 10.8.378 10.1959 12.1657 11.2741 10.4773 12.6593 11.6896 108276 13.1339 12.0853 11.1581 1.3.5903 12.4622 11.4699 15.6221 14.0939 12.7814 17 2920 15.3725 13.7618 18.6616 16.3742 14.4982 19.7928 17.1591 151463 12.1.337 7.4869 13 14 7.10.34 7.3667 6.1944 6.4235 6.6282 6.8109 8,7455 9.2526 9.9540 10.6.350) 11.2961 11.9379 12.5611 13.1661 13.7535 14.323 13 14 15 15 12 8493 16 13.5777 8.0607 8.3126 8.5425 6.9740 13.0032 13.8651 14.7179 15.5623 16.3983 17.2260 18.045 16 17 5.8474 5.9542 6.0472 6.1280 14.2919 7.4987 7.1390 7.9127 7.5361 8.3577 7.9038 8.2442 9.1079 8.3593 9.4466 8.8514 9.7632 9.1216 10.0391 9.3719 10.3356 9.6036 10.504 9.8IRI 11.6526 10.6748 12.100 11.2578 12.9477 11.6546 13,5217 11.9246 17 18 8.7556 18 7.6361 7.8237 1.1216 8.2014 8.3619 8.51.16 90770 9:4269 14.9920 15.6785 16.3514 7.1196 7.2497 7.3658 7.4194 19 8.9501 9.1285 6.1982 6.2593 19 20 20 14.8725 25 17,4131 9.8226 7.8431 6.4641 25 19.5235 22 3965 6.5660 30 30 35 22.0232 25.8077 19.1086 32.8247 24.9986 19.6001 21.4872 23.1148 10.2757 10.566 10.2574 9.6412 8.0552 8.1755 8.2438 35 6.6166 6.6418 40 27.3555 9.7291 40 *lised to calculate the present value of a series of equal payments made ar zile end of each period. For example: What is the present value of $2.00) per year for 10 years assuming an annual interest rate of 98? For i = 10,i = 93). Pjector is 6.4177. $2.00 per year for 10 years is the equivalent of 372,835 inday S2000x4177