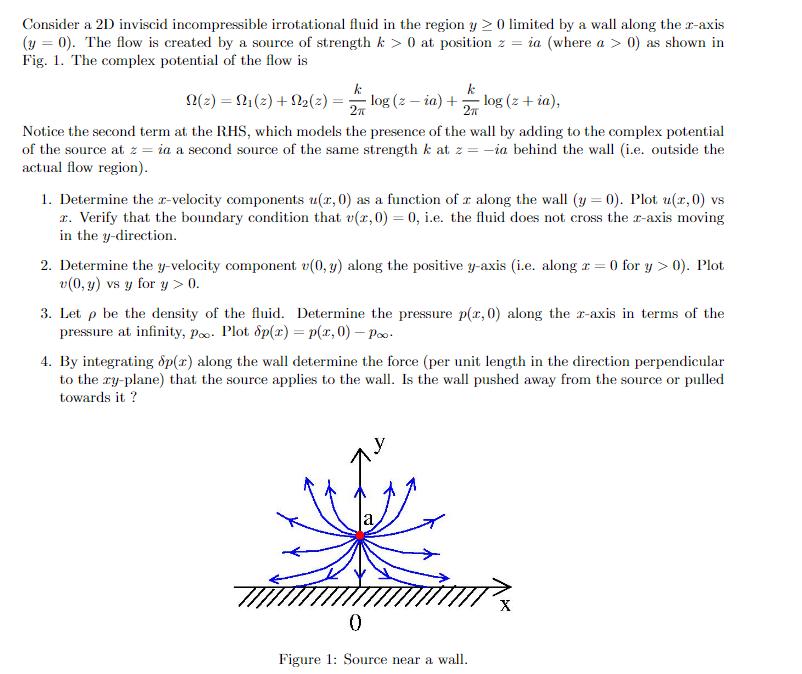
Consider a 2D inviscid incompressible irrotational fluid in the region y 20 limited by a wall along the r-axis (y=0). The flow is created by a source of strength k> 0 at position z = ia (where a > 0) as shown in Fig. 1. The complex potential of the flow is N(z) = N(z) + N(z) = log (z-ia) + k 2 Notice the second term at the RHS, which models the presence of the wall by adding to the complex potential of the source at z = ia a second source of the same strength k at z=-ia behind the wall (i.e. outside the actual flow region). k - log (z + ia), - 2 1. Determine the x-velocity components u(x,0) as a function of r along the wall (y=0). Plot u(x, 0) vs r. Verify that the boundary condition that v(x,0) = 0, i.e. the fluid does not cross the x-axis moving in the y-direction. 2. Determine the y-velocity component v(0, y) along the positive y-axis (i.e. along z = 0 for y> 0). Plot v(0, y) vs y for y > 0. 3. Let p be the density of the fluid. Determine the pressure p(x, 0) along the z-axis in terms of the pressure at infinity, Poo. Plot Sp(x) = p(x,0) - Poo. 4. By integrating Sp(r) along the wall determine the force (per unit length in the direction perpendicular to the ry-plane) that the source applies to the wall. Is the wall pushed away from the source or pulled towards it ? 0 Figure 1: Source near a wall.