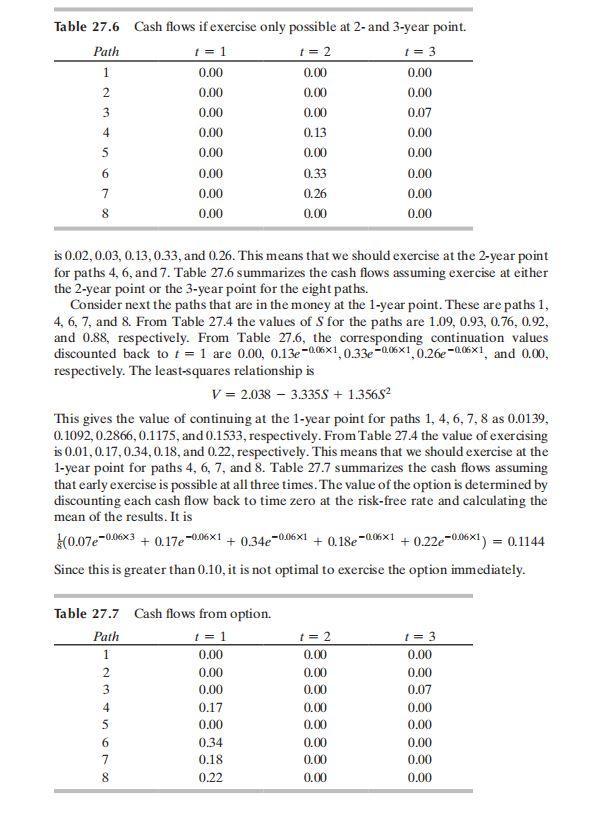
Consider a 3-year American put option on a non-dividend-paying stock that can be exercised at the end of year 1, the end of year 2, and the end of year 3. The risk-free rate is 6% per annum (continuously compounded). The current stock price is 1.00 and the strike price is 1.10. Assume that the eight paths shown in Table 27.4 are sampled for the stock price. (This example is for illustration only; in practice many more paths would be sampled.) If the option can be exercised only at the 3-year point, it provides a cash flow equal to its intrinsic value at that point. This is shown in the last column of Table 27.5. If the put option is in the money at the 2-year point, the option holder must decide whether to exercise. Table 27.4 shows that the option is in the money at the 2-year point for paths 1, 3, 4, 6, and 7. For these paths, we assume an approximate relationship: V = a + b + cS where S is the stock price at the 2-year point and V is the value of continuing, discounted back to the 2-year point. Our five observations on S are: 1.08, 1.07, 0.97, 0.77, and 0.84. From Table 27.5 the corresponding values for V are: 0.00, 0.07e-0.06x1, 0.18e-006x1,0.20e-0.06x1, and 0.09e-0.06x1. The values of a, b, and c that minimize (V-a - bS, cS}) where S, and V, are the ith observation on S and V, respectively, are a = -1.070, b = 2.983 and c = -1.813, so that the best-fit relationship is V = -1.070 + 2.983S - 1.8138 This gives the value at the 2-year point of continuing for paths 1, 3, 4, 6, and 7 of 0.0369, 0.0461,0.1176,0.1520, and 0.1565, respectively. From Table 27.4 the value of exercising Table 27.6 Path 1 N3450 2 679 8 is 0.02, 0.03, 0.13,0.33, and 0.26. This means that we should exercise at the 2-year point for paths 4, 6, and 7. Table 27.6 summarizes the cash flows assuming exercise at either the 2-year point or the 3-year point for the eight paths. Consider next the paths that are in the money at the 1-year point. These are paths 1, 4, 6, 7, and 8. From Table 27.4 the values of S for the paths are 1.09, 0.93, 0.76, 0.92, and 0.88, respectively. From Table 27.6, the corresponding continuation values discounted back to t= 1 are 0.00, 0.13e-006x1, 0.33e-0.06x1,0.26e-006x1, and 0.00, respectively. The least-squares relationship is V = 2.038 3.335S + 1.3565 Cash flows if exercise only possible at 2- and 3-year point. 1 = 1 t = 2 t = 3 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.07 0.00 0.13 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00 This gives the value of continuing at the 1-year point for paths 1, 4, 6, 7, 8 as 0.0139, 0.1092, 0.2866, 0.1175, and 0.1533, respectively. From Table 27.4 the value of exercising is 0.01, 0.17.0.34, 0.18, and 0.22, respectively. This means that we should exercise at the 1-year point for paths 4, 6, 7, and 8. Table 27.7 summarizes the cash flows assuming that early exercise is possible at all three times. The value of the option is determined by discounting each cash flow back to time zero at the risk-free rate and calculating the mean of the results. It is (0.07e-0.06x3 + 0.17e-0.06x1 + 0.34e-0.06x1 + 0.18e-006x1 +0.22e-0.06x1) = 0.1144 Since this is greater than 0.10, it is not optimal to exercise the option immediately. Table 27.7 Cash flows from option. Path t = 1 1 0.00 0.00 0.00 0.17 0.00 23 4 56780 0.33 0.26 0.00 0.34 0.18 0.22 t=2 0.00 0.00 0.00 0.00 0.00 0.00 0.00 0.00 1=3 0.00 0.00 0.07 0.00 0.00 0.00 0.00 0.00 For the Least Squares Monte Carlo example in Hull section 27.8, redo the exercise using a 100 or more scenarios. Generate your own risk-neutral random stock prices with r = 3% and o = 20%. Strike price is 110 and initial stock price is 100.