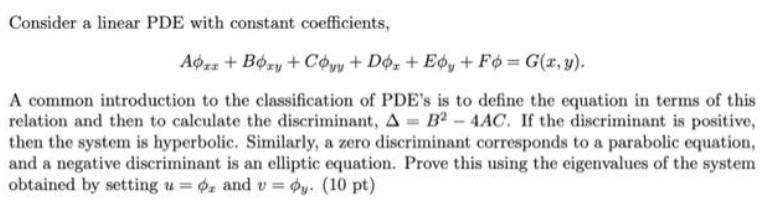
Consider a linear PDE with constant coefficients, Az + Boxy + Coyy + Do + Edy + Fo=G(x, y). A common introduction to the classification of PDE's is to define the equation in terms of this relation and then to calculate the discriminant, A= B2-4AC. If the discriminant is positive, then the system is hyperbolic. Similarly, a zero discriminant corresponds to a parabolic equation, and a negative discriminant is an elliptic equation. Prove this using the eigenvalues of the system obtained by setting up, and v=y. (10 pt)