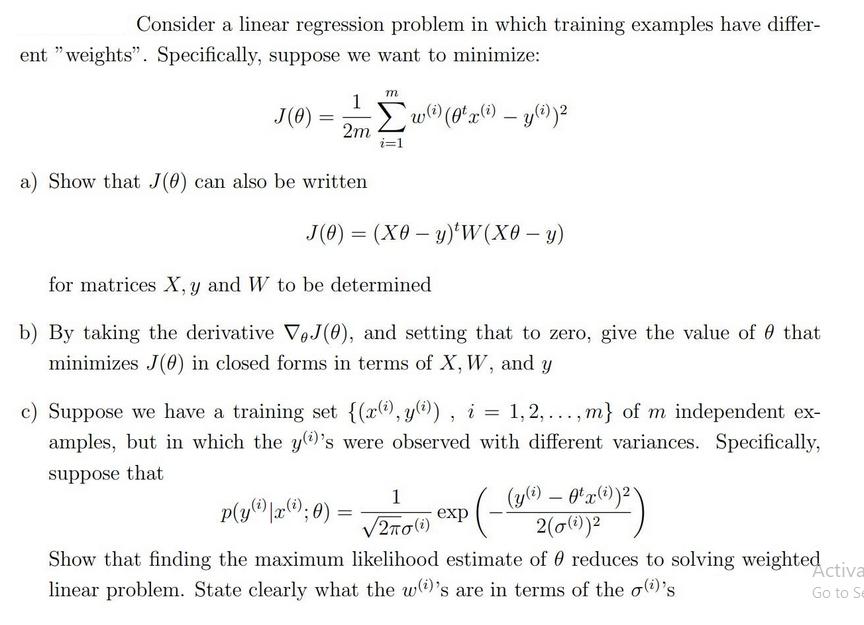
Consider a linear regression problem in which training examples have differ- ent "weights". Specifically, suppose we want to minimize: J(0): = 1 - w(i) (0 x(i) - y(i)) 2m a) Show that J(0) can also be written m J(0) = (X0 y)*W(X0y) p(y () |x (1); 0) for matrices X, y and W to be determined b) By taking the derivative VJ(0), and setting that to zero, give the value of 0 that minimizes J(0) in closed forms in terms of X, W, and y c) Suppose we have a training set {(r(), y)), i = 1,2,...,m} of m independent ex- amples, but in which the y(i)'s were observed with different variances. Specifically, suppose that = 1 27010) exp (- (2(i) (y(i) - Otx(i))2) 2(())2 Show that finding the maximum likelihood estimate of reduces to solving weighted linear problem. State clearly what the w()'s are in terms of the o()'s Activa Go to Se