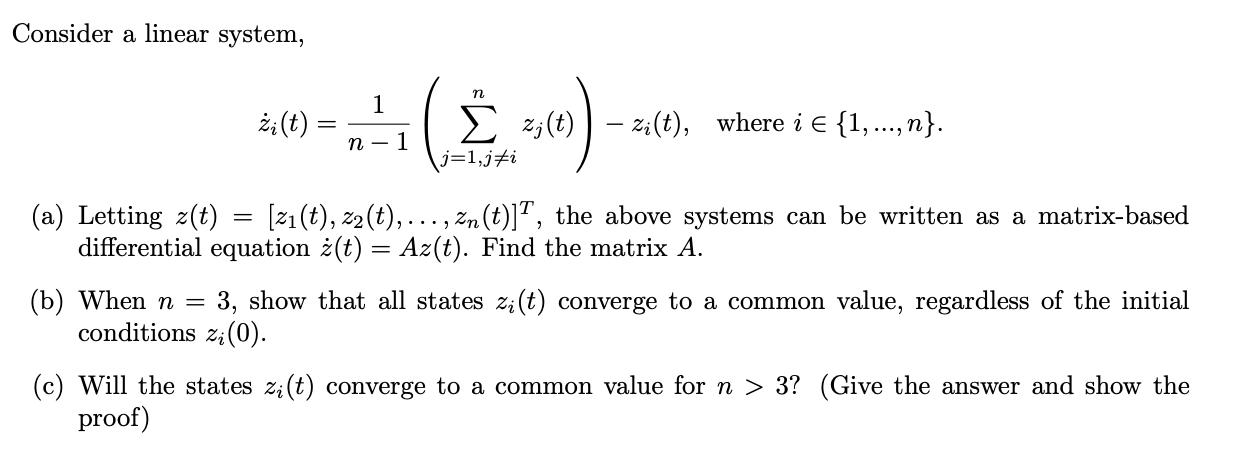
Consider a linear system, n 1 i(t) = n-1 2; (1) -zi(t), where i {1, ..., n}. j=1,ji (a) Letting z(t) = [z1(t), z2(t), ,..., Zn(t)], the above systems can be written as a matrix-based differential equation (t) = Az(t). Find the matrix A. (b) When n = = 3, show that all states zi (t) converge to a common value, regardless of the initial conditions zi(0). (c) Will the states zi(t) converge to a common value for n > 3? (Give the answer and show the proof)