Question: Consider a nonsymmetric real matrix A which has the Schur decomposition A = QRAH where Q is unitary, and R is upper trian- gular.

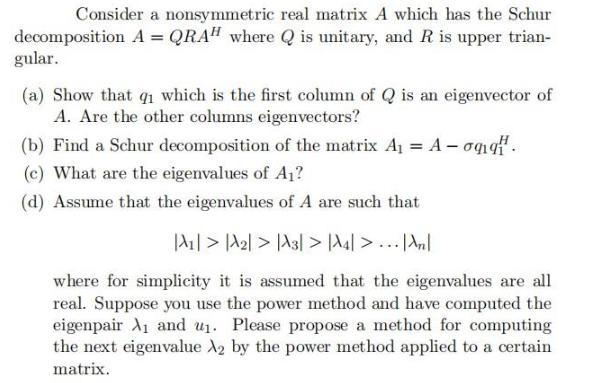
Consider a nonsymmetric real matrix A which has the Schur decomposition A

Consider a nonsymmetric real matrix A which has the Schur decomposition A = QRAH where Q is unitary, and R is upper trian- gular. (a) Show that q which is the first column of Q is an eigenvector of A. Are the other columns eigenvectors? (b) Find a Schur decomposition of the matrix A = A -oggf. (c) What are the eigenvalues of A? (d) Assume that the eigenvalues of A are such that A1 A2 A3| > |A4|>... |n| where for simplicity it is assumed that the eigenvalues are all real. Suppose you use the power method and have computed the eigenpair A and u. Please propose a method for computing the next eigenvalue A2 by the power method applied to a certain matrix.

Step by Step Solution

★★★★★

3.50 Rating (147 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

a Since Q is unitary its columns are orthonormal and thus q1 is a unit vector From the Schur decompo... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Accounting Questions!

Show that an arbitrary n Ã n unitary matrix has n 2 real parameters, and hence that is the most general form of a 2 Ã 2 unitary matrix. The most general form of (d, s) mixing is where U...

Suppose that A1, A2 , A3 , and B are events where A1 , A2 , and A3 are mutually exclusive and P (A1) =.2 P (A2) =.5 P (A3) = .3 P (B A1) = .02 P (B A2) =.05 P( B A3 )=.04 Use this information to find...

Show that if U is an n Ã n unitary matrix and |z1| = |z2| = ... = |zn| = 1, then the product is also unitary. Z1 0 0.0 0 z200 0 0 0 Zn

An experiment was carried out to investigate the effect of species (factor A, with I = 4) and grade (factor B, with J = 3) on breaking strength of wood specimens. One observation was made for each...

For each of the following tables of cell means, calculate marginal means (X A and X B ) and the total mean (X T ). a. b. Factor A a a2 bu 3.00 4.00 Factor B b2 2.00 2.00 Factor A a1 a2 b 8.50 6.50...

13.12 Following are response times (in milliseconds) obtained under four different conditions for eight subjects: Subject A1 A2 A3 A4 1 2036 2220 2211 2316 2 2034 2042 2094 2077 3 2198 2612 2272 2348...

Let X and Y be two random variables for which only 6 possible events, A1, A2, A3 A4, A5, A6,are defined:i 1 2 3 4 5 6P(Ai) 0.3 0.1 0.1 0.2 0.2 0.1Xi -1 2 2 -1 -1 2Yi 0 2 0 1 2 1a) [5 marks] What is...

Electricity: $20,000, Depreciation: $10,000, Rent: $30,000 Overhead costs will be assigned to activities on the basis of the factors given below OH COST DRIVER Electricity ($20,000) No. of lamp...

10 pts D Question 1 List the first five terms of the sequence {an } defined by an = cos ( If any answer is not a whole number, enter it as a decimal rounded to three places. . a1 = . a2 . a3 . a4 = ....

Prove that A n is simple for n 5, following the steps given. a. Show A n contains every 3-cycle if n 3. b. Show An is generated by the 3-cycles for n 3. c. Let r and s be fixed elements of {l, 2, ...

The truss ABC shown in the figure supports a horizontal load P1 = 300 lb and a vertical load P2 = 900 lb. Both bars have cross-sectional area A = 2.4 in.2 and are made of steel with E = 30 Ã...

Compute the Darcy velocity and seepage velocity for water flowing through a sand column with the following characteristics: K = 10 - 4 cm/s dh/dl = 0.01, Area = 75 cm2, n = 0.20.

how do you make the journal entries for year 1 - 4

What is an advantage that the Du-Pont system of analysis offers over traditional ratio analysis? (Try and identify something original that your classmates have not yet mentioned.) Are there any...

Eastman, Inc., manufactures and sells three products: R, S, and T. In January, Eastman, Inc., budgeted sales of the following. At the end of the year, actual sales revenue for Product R and Product S...

Presented below are data on three promissory notes. Determine the missingamounts. Date of Note Total Maturity Annual Interest Rate Principal Terms 60 days 30 days 6 months Date Interest (a) April 1...

Nicoloff Corporation reported the following information (in thousands) at December 31, 2012: Additional information: 1. The bank loan was increased by additional borrowings of $300 to partially...

In 1971, Harry Gordon turned over $40,000 to his son, Murray Gordon. Murray opened two $20,000 custodial bank accounts under the Uniform Gifts to Minors Act for his minor children, Eden and...

The questionnaire item "Did you file federal and state income tax reports last year?" with yes/no/don't remember categories is an example of Group of answer choices An open-ended question. An...

Can anybody help me out on this question? please explain nicely and clearly. Thank you for the help 2. Consider the following real matrix A (all calculations are ordinary real number calculations) 1...

Could you please help with question 326? Thanks Exercise 309 Exercise 310 Theorem 3 Exercise 311 Exercise 312 75 5.8 Real symmetric matrices Real symmetric matrices play the role of real numbers in...

Could you please help with question 324 and 325? Thanks Exercise 309 Exercise 310 Theorem 3 Exercise 311 Exercise 312 75 5.8 Real symmetric matrices Real symmetric matrices play the role of real...

Could you please help with question 317 and 318? Thanks Exercise 309 Exercise 310 Theorem 3 Exercise 311 Exercise 312 75 5.8 Real symmetric matrices Real symmetric matrices play the role of real...

Could you please help with question 319 and 320? Thanks Exercise 309 Exercise 310 Theorem 3 Exercise 311 Exercise 312 75 5.8 Real symmetric matrices Real symmetric matrices play the role of real...

ENGI 398: Engineering Mathematics Project 3: Application of Singular Value Decomposition to Image Compression1 1 Background The singular value decomposition (SVD) is a fundamental concept in linear...