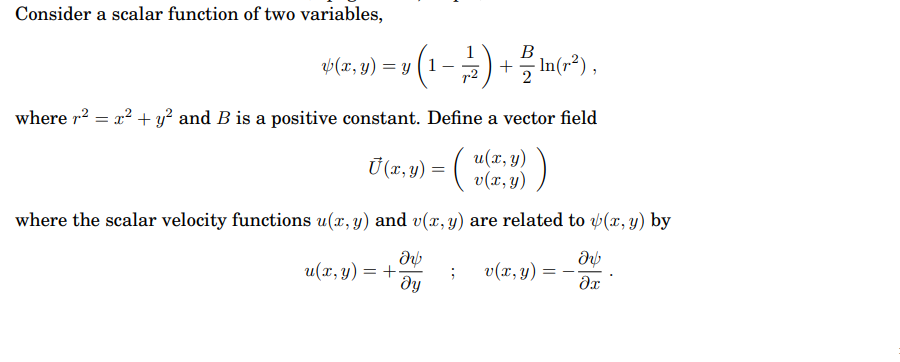
Consider a scalar function of two variables, v(x, y) = y (1 - + ln(r), where r = x + y and B is a positive constant. Define a vector field U (x,y) = ( u(x,y) ) where the scalar velocity functions u(x, y) and v(x, y) are related to (x, y) by u(x, y) = + ; v(x, y) = Calculate these velocities and evaluate explicitly the divergence of the velocity field. Carry out this calculation in (i) mixed Cartesian variables {x,y & r} where r derivatives are done implicitly, and in (ii) purely polar coordinates {r, 0}. (You need not show the calculations, just a summary of the key results this is not Calc 3 anymore.). Explain why the end result could have been anticipated without the explicit calculation, and that (x, y) is perpendicular to (x, y). It is also simple to show that (x, y) can be represented as a curl (VX). But there is more, show (x, y) can also be represented a gradient, Vo(x, y) by constructing the simplest (x, y). (Could this result have been anticipated, or is there something really special going on?) Lastly, your (x, y) will seem to be multi- valued, but do you think this is a serious issue? (Hint: if you have difficulty getting started, try setting B = 0 first.)