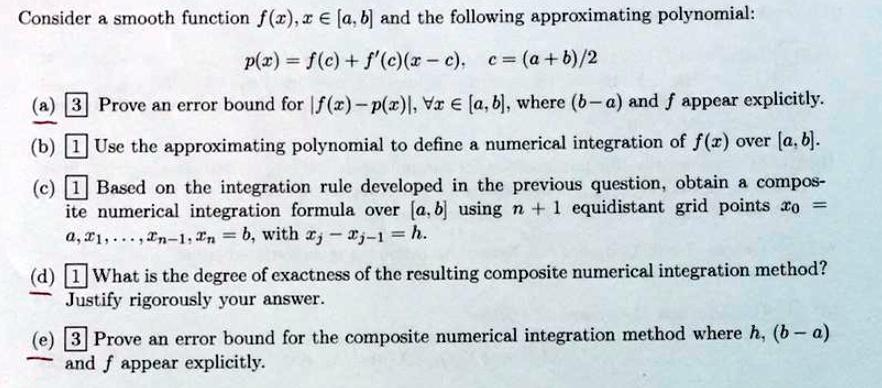
Consider a smooth function f(x), x E [a, b] and the following approximating polynomial: p(x) = f(c) + f'(c)(x-c), c=(a+b)/2 (a) 3 Prove an error bound for f(x)-p(x)\, Vxe [a, b], where (b-a) and f appear explicitly. (b) Use the approximating polynomial to define a numerical integration of f(x) over [a, b]. (c) 1 Based on the integration rule developed in the previous question, obtain a compos- ite numerical integration formula over [a, b] using n + 1 equidistant grid points x0 = a, 21,2n-1, n = b, with x-x-1= h. (d) What is the degree of exactness of the resulting composite numerical integration method? Justify rigorously your answer. (e) 3 Prove an error bound for the composite numerical integration method where h, (b-a) and f appear explicitly.