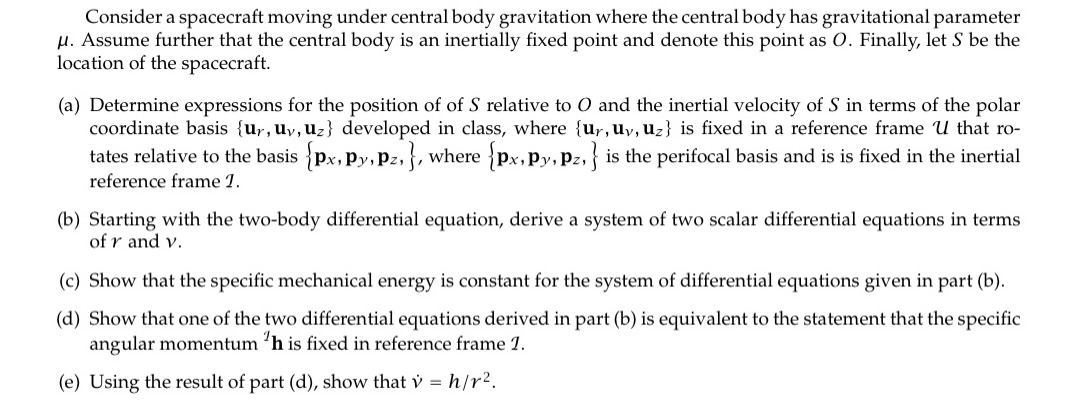
Consider a spacecraft moving under central body gravitation where the central body has gravitational parameter . Assume further that the central body is an inertially fixed point and denote this point as O. Finally, let S be the location of the spacecraft. (a) Determine expressions for the position of of S relative to O and the inertial velocity of S in terms of the polar coordinate basis {ur, uv, uz} developed in class, where {ur, uv, uz} is fixed in a reference frame U that ro- tates relative to the basis {px, py, pz, }, where {px,py, pz,} is the perifocal basis and is is fixed in the inertial reference frame 1. (b) Starting with the two-body differential equation, derive a system of two scalar differential equations in terms of r and v. (c) Show that the specific mechanical energy is constant for the system of differential equations given in part (b). (d) Show that one of the two differential equations derived in part (b) is equivalent to the statement that the specific angular momentum h is fixed in reference frame 1. (e) Using the result of part (d), show that v = h/r.