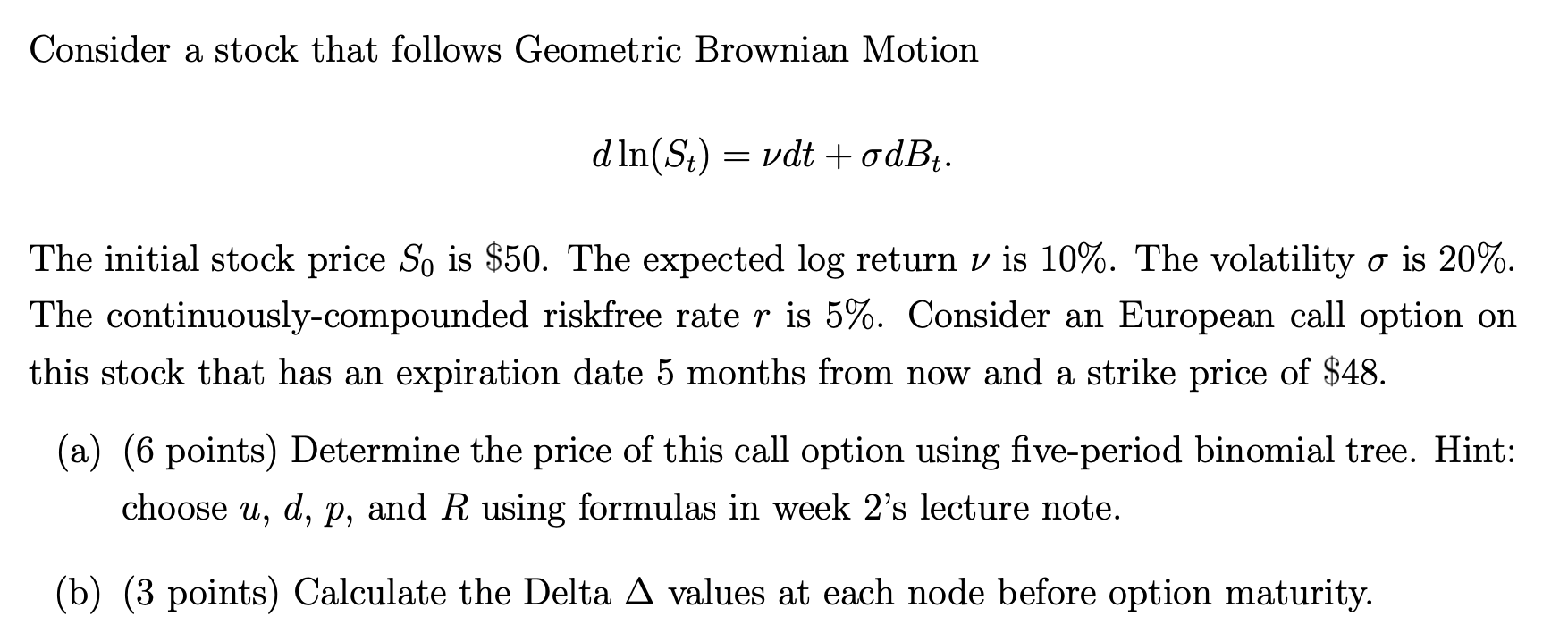
Consider a stock that follows Geometric Brownian Motion dIn(St) =vdt + odBt. The initial stock price So is $50. The expected log return v is 10%. The volatility o is 20%. The continuously-compounded riskfree rate r is 5%. Consider an European call option on this stock that has an expiration date 5 months from now and a strike price of $48. (a) (6 points) Determine the price of this call option using five-period binomial tree. Hint: choose u, d, p, and R using formulas in week 2's lecture note. (b) (3 points) Calculate the Delta A values at each node before option maturity. This problem uses the same assumption as the Problem 2 above. (a) (3 points) Calculate the value of the same European call option considered in the Problem 2 using Black-Scholes call option valuation formula. (b) (3 points) Use the Black-Scholes formula to calculate the price of a European put option with the same maturity of 5 months and the same strike price of $48. (c) (3 points) Numerically verify the put-call parity for this pair of call and put options. Consider a stock that follows Geometric Brownian Motion dIn(St) =vdt + odBt. The initial stock price So is $50. The expected log return v is 10%. The volatility o is 20%. The continuously-compounded riskfree rate r is 5%. Consider an European call option on this stock that has an expiration date 5 months from now and a strike price of $48. (a) (6 points) Determine the price of this call option using five-period binomial tree. Hint: choose u, d, p, and R using formulas in week 2's lecture note. (b) (3 points) Calculate the Delta A values at each node before option maturity. This problem uses the same assumption as the Problem 2 above. (a) (3 points) Calculate the value of the same European call option considered in the Problem 2 using Black-Scholes call option valuation formula. (b) (3 points) Use the Black-Scholes formula to calculate the price of a European put option with the same maturity of 5 months and the same strike price of $48. (c) (3 points) Numerically verify the put-call parity for this pair of call and put options