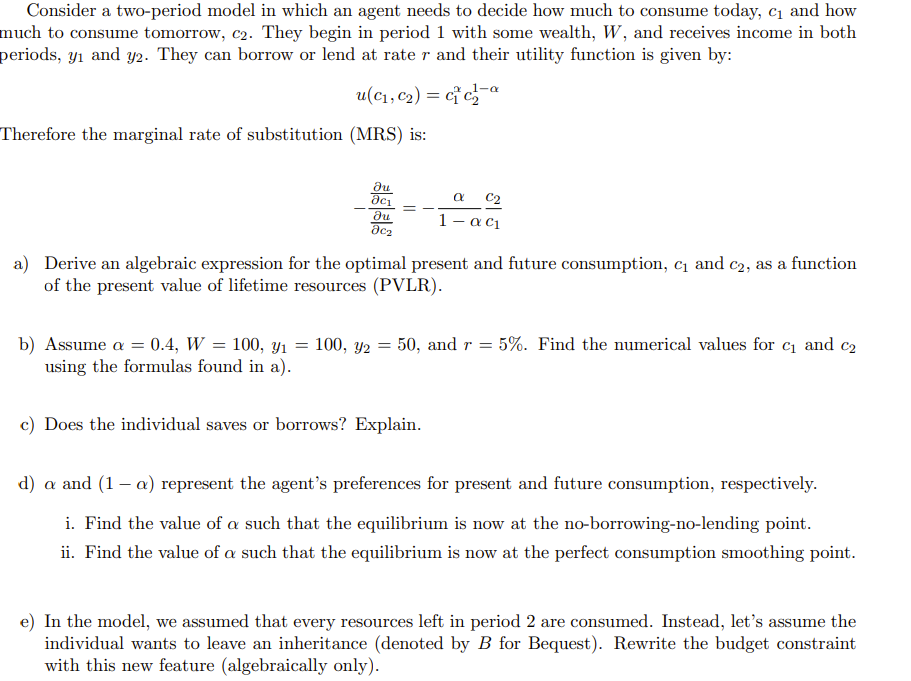
Consider a two-period model in which an agent needs to decide how much to consume today, c and how much to consume tomorrow, c2. They begin in period 1 with some wealth, W, and receives income in both periods, y and y2. They can borrow or lend at rate r and their utility function is given by: u(c1, c2) = cca Therefore the marginal rate of substitution (MRS) is: C2 = 1- a c Ju c2 a) Derive an algebraic expression for the optimal present and future consumption, c and c2, as a function of the present value of lifetime resources (PVLR). b) Assume a = 0.4, W = 100, y1 = 100, y2 = using the formulas found in a). 50, and r 5%. Find the numerical values for c and c c) Does the individual saves or borrows? Explain. = d) a and (1a) represent the agent's preferences for present and future consumption, respectively. i. Find the value of a such that the equilibrium is now at the no-borrowing-no-lending point. ii. Find the value of a such that the equilibrium is now at the perfect consumption smoothing point. e) In the model, we assumed that every resources left in period 2 are consumed. Instead, let's assume the individual wants to leave an inheritance (denoted by B for Bequest). Rewrite the budget constraint with this new feature (algebraically only).