Question: Consider a zero-mean random vector X = (X X) corrupted by a zero- mean noise vector y = (y y2) that is statistically independent

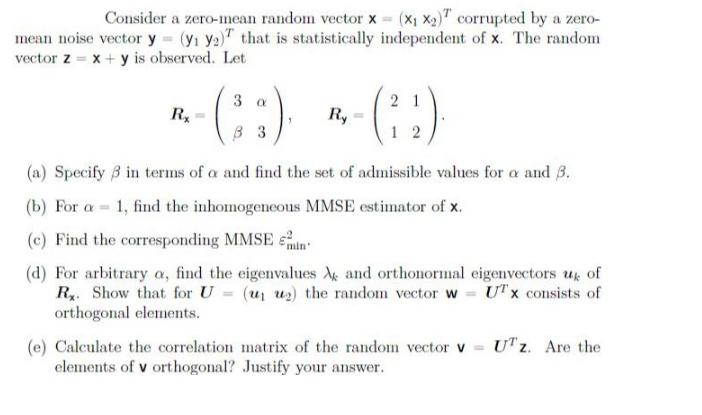
Consider a zero-mean random vector X = (X X) corrupted by a

Consider a zero-mean random vector X = (X X) corrupted by a zero- mean noise vector y = (y y2) that is statistically independent of x. The random vector z = x + y is observed. Let Rx - 3 a B3 Ry 21 (a) Specify 3 in terms of a and find the set of admissible values for a and 3. (b) For a 1, find the inhomogeneous MMSE estimator of x. (c) Find the corresponding MMSE in (d) For arbitrary a, find the eigenvalues A and orthonormal eigenvectors u of R.. Show that for U= (uus) the random vector w = UT x consists of orthogonal elements. (e) Calculate the correlation matrix of the random vector v = UTz. Are the elements of v orthogonal? Justify your answer.

Step by Step Solution

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

You have to show all the for each of these questions ,Please use sheet of paper to solve. (DON'T USE Ai) compute the energy of a photon at 0.6,0.8 and 1.3um .which has more energy , a visible or...

QUIZ... Let D be a poset and let f : D D be a monotone function. (i) Give the definition of the least pre-fixed point, fix (f), of f. Show that fix (f) is a fixed point of f. [5 marks] (ii) Show that...

If you dissolve 8.14 grams of calcium chloride, CaCl 2 , in enough water to make 125.0 mL of solution, what is the molarity of the solution?

. Consider the arbitrary random vector X = [X1, X2, X3, X4, X5] with mean vector = [1, 2, 3, 4, 5]. Partition X into X = X(1) X(2) where X(1) = X1 X2 and X(2) = X3 X4 X5 Let be the covariance matrix...

Question 2. [26 marks] (a) [6 marks] Consider a p-dimensional random vector X where X-Np(0px1, [pxp). Answer the follow questions. (i) What is the distribution of X1 - 2X2, where X] and X2 are both...

. Consider the arbitrary random vector X? = [X1, X2, X3, X4,X5] with mean vector ? = [1, 2, 3, 4, 5]. Partition X into X= X(1) X(2) where X(1) = X1 X2 and X(2) = ?? X3 X4 X5 ? 3. Consider the...

13. (3 points) Consider a scalar-valued random variable X that takes values {1,2,3,4} with probabilities Px(1) = 0.2, px (2) = 0.3, px(3) = 0.25, and px (4) = 0.25. Compute the mean and variance of...

independent. A random vector (X, Y ) is said to be standard bivariate Gaussian if its joint density is given by: T f(x, y) = X exp E (27) V(1 + (EXY ) 2) Here E is the covariance matrix given by EXY...

Please resolve. Jack and Sam are both taking a statistics class this summer. They decided to study separately for their exam. The probability that Jack will make an "A" in statistics is 0.6, and the...

A PC research office in a school has 33 PCs. All of the 33 PCs has 90% relentless quality. Considering 10% of the PCs to be down, an instructor shows an enrollment top of 30 for his gathering. Expect...

Consider the following information: Rate of Return if State Occurs State of Economy Probability of State of Economy Stock A Stock B Recession .10 .04 .23 Normal .60 .08 .15 Boom .30 .12 .32 Calculate...

Save & Exit Brandon, an individual, began business four years ago and has sold 1231 assets with $5,900 of losses within the last five years. Brandon owned each of the assets for several years. In the...

The Lim Company has a plant that manufacturers transistor radios. The production time is only a few minutes per unit. The company uses a just-in- time production system and a backflush costing system...

Llungby AB spent 1,000,000 krone in 2017 on the development of a new product. The company determined that 25 percent of this amount was incurred after the criteria in IAS 36 for capitalization as an...

Hill Country Handyman Services has total assets for the year of $ 12,500 and total liabilities of $ 8,240. Requirements 1. Use the accounting equation to solve for equity. 2. If next year assets...

As the manager of Mighty Fine Mexican Restaurant, you must deal with a variety of business transactions. Provide an explanation for the following transactions: a. Debit Equipment and credit Cash. b....

When is a PERT diagram useful for systems projects?

The alternative to investing in the new production line in Problem 11.35 is to overhaul the existing line, which currently has both a book value and a salvage value of $0. It would cost $300,000 to...

your father is about to retire and he wants to buy an annuity that will provide him with 7 8 0 0 0 of income a year for 2 5 years with the first payment coming immediatly the going rate on such...

Prove that if f is analytic on R and f(x0) 0 for some x0 R, then f Cc(R).

Find the interval of convergence of each of the following power series. a) b) c) d) (2k +1)2 0

Let {ak} and be real sequences. Decide which of the following statements are true and which are false. Prove the true ones and give counterexamples to the false ones. a) If ak is strictly decreasing...

IBM Inc. is going to award a contract for fine-line pens to be used nationally in its offices. Two suppliers, Bic and Pilot, have submitted bids. To determine the preference of office employees,...

The Citrus Council of America wants to determine whether consumers prefer plain orange juice or juice with some orange pulp in it. A random sample of 212 consumers was selected. Each member of the...

Refer to the Baseball 2012 data, which report information on the 30 Major League Baseball teams for the 2012 season. Set up a variable that divides the teams into two groups, those that had a winning...