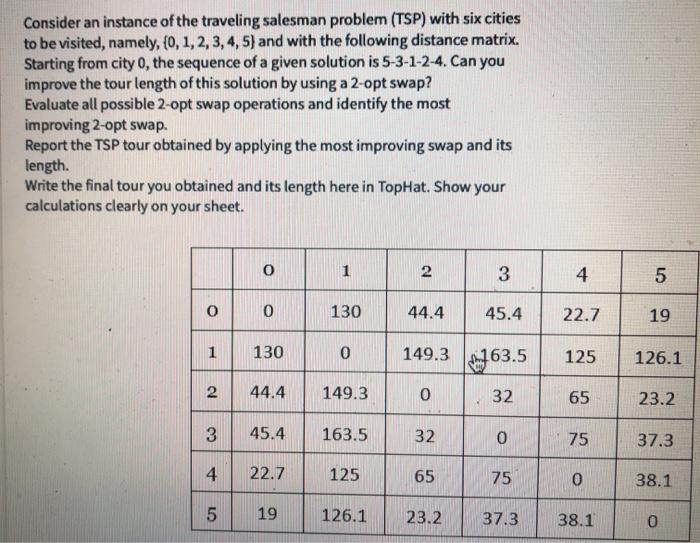
Consider an instance of the traveling salesman problem (TSP) with six cities to be visited, namely, (0, 1, 2, 3, 4, 5) and with the following distance matrix. Starting from city 0, the sequence of a given solution is 5-3-1-2-4. Can you improve the tour length of this solution by using a 2-opt swap? Evaluate all possible 2-opt swap operations and identify the most improving 2-opt swap. Report the TSP tour obtained by applying the most improving swap and its length. Write the final tour you obtained and its length here in TopHat. Show your calculations clearly on your sheet. 0 1 2 3 4 5 0 0 130 44.4 45.4 22.7 19 1 130 0 149.3 163.5 125 126.1 2 44.4 32 149.3 163.5 0 32 65 3 23.2 45.4 0 75 37.3 4 22.7 125 65 75 0 38.1 5 19 126.1 23.2 37.3 38.1 0