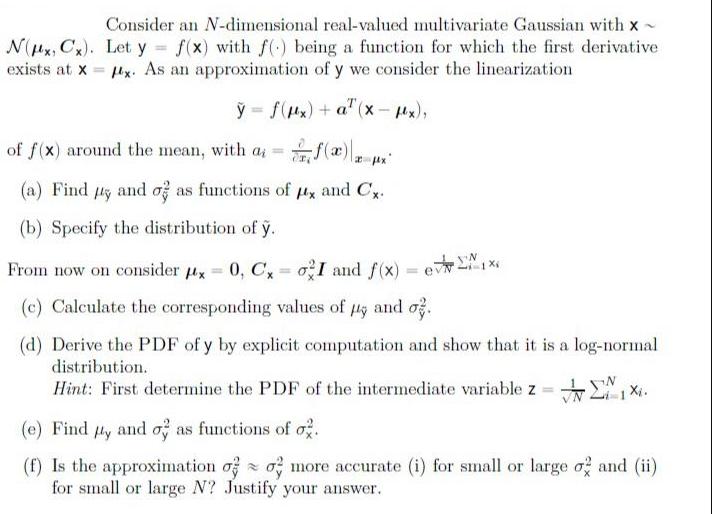
Consider an N-dimensional real-valued multivariate Gaussian with x N(x, Cx). Let y = f(x) with f() being a function for which the first derivative exists at x = x. As an approximation of y we consider the linearization y = f(x) + a (x-x), f(x)\xx of f(x) around the mean, with a = (a) Find uy and o as functions of x and Cx. (b) Specify the distribution of y. From now on consider x = 0, Cx = I and f(x) (c) Calculate the corresponding values of g and o (d) Derive the PDF of y by explicit computation and show that it is a log-normal distribution. Hint: First determine the PDF of the intermediate variable z (e) Find uy and o and o as functions of o N e 1 X( 1 XI. RE (f) Is the approximation of more accurate (i) for small or large o and (ii) for small or large N? Justify your answer.