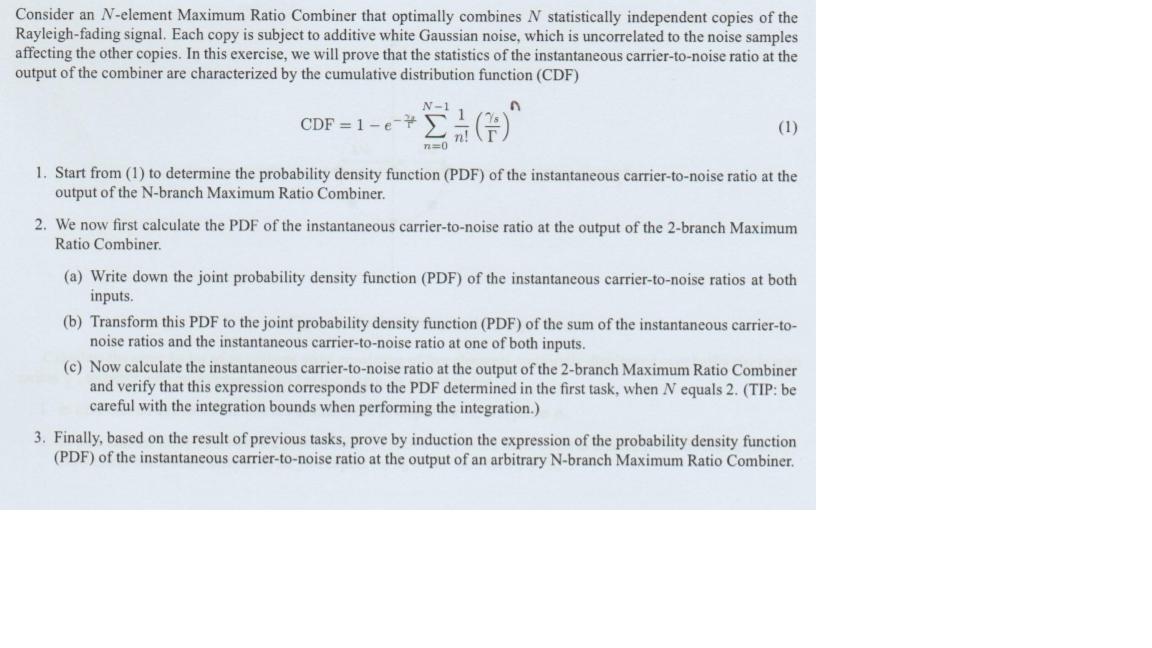
Consider an N-element Maximum Ratio Combiner that optimally combines N statistically independent copies of the Rayleigh-fading signal. Each copy is subject to additive white Gaussian noise, which is uncorrelated to the noise samples affecting the other copies. In this exercise, we will prove that the statistics of the instantaneous carrier-to-noise ratio at the output of the combiner are characterized by the cumulative distribution function (CDF) CDF = 1-e() n=0 (1) 1. Start from (1) to determine the probability density function (PDF) of the instantaneous carrier-to-noise ratio at the output of the N-branch Maximum Ratio Combiner. 2. We now first calculate the PDF of the instantaneous carrier-to-noise ratio at the output of the 2-branch Maximum Ratio Combiner. (a) Write down the joint probability density function (PDF) of the instantaneous carrier-to-noise ratios at both inputs. (b) Transform this PDF to the joint probability density function (PDF) of the sum of the instantaneous carrier-to- noise ratios and the instantaneous carrier-to-noise ratio at one of both inputs. (c) Now calculate the instantaneous carrier-to-noise ratio at the output of the 2-branch Maximum Ratio Combiner and verify that this expression corresponds to the PDF determined in the first task, when N equals 2. (TIP: be careful with the integration bounds when performing the integration.) 3. Finally, based on the result of previous tasks, prove by induction the expression of the probability density function (PDF) of the instantaneous carrier-to-noise ratio at the output of an arbitrary N-branch Maximum Ratio Combiner.