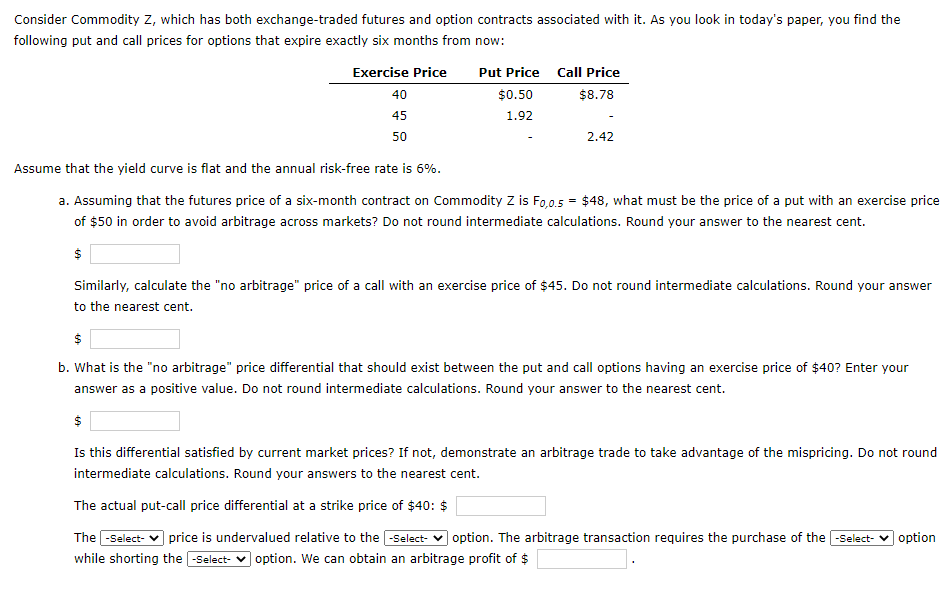
Consider Commodity Z, which has both exchange-traded futures and option contracts associated with it. As you look in today's paper, you find the following put and call prices for options that expire exactly six months from now: Exercise Price 40 45 50 Put Price Call Price $0.50 $8.78 1.92 2.42 Assume that the yield curve is flat and the annual risk-free rate is 5%. a. Assuming that the futures price of a six-month contract on Commodity Z is F0,0.5 = $48, what must be the price of a put with an exercise price of $50 in order to avoid arbitrage across markets? Do not round intermediate calculations. Round your answer to the nearest cent. $ Similarly, calculate the "no arbitrage" price of a call with an exercise price of $45. Do not round intermediate calculations. Round your answer to the nearest cent. b. What is the "no arbitrage" price differential that should exist between the put and call options having an exercise price of $40? Enter your answer as a positive value. Do not round intermediate calculations. Round your answer to the nearest cent. S Is this differential satisfied by current market prices? If not, demonstrate an arbitrage trade to take advantage of the mispricing. Do not round intermediate calculations. Round your answers to the nearest cent. The actual put-call price differential at a strike price of $40: $ The -Select price is undervalued relative to the - Select option. The arbitrage transaction requires the purchase of the - Select option while shorting the - Select option. We can obtain an arbitrage profit of $ Consider Commodity Z, which has both exchange-traded futures and option contracts associated with it. As you look in today's paper, you find the following put and call prices for options that expire exactly six months from now: Exercise Price 40 45 50 Put Price Call Price $0.50 $8.78 1.92 2.42 Assume that the yield curve is flat and the annual risk-free rate is 5%. a. Assuming that the futures price of a six-month contract on Commodity Z is F0,0.5 = $48, what must be the price of a put with an exercise price of $50 in order to avoid arbitrage across markets? Do not round intermediate calculations. Round your answer to the nearest cent. $ Similarly, calculate the "no arbitrage" price of a call with an exercise price of $45. Do not round intermediate calculations. Round your answer to the nearest cent. b. What is the "no arbitrage" price differential that should exist between the put and call options having an exercise price of $40? Enter your answer as a positive value. Do not round intermediate calculations. Round your answer to the nearest cent. S Is this differential satisfied by current market prices? If not, demonstrate an arbitrage trade to take advantage of the mispricing. Do not round intermediate calculations. Round your answers to the nearest cent. The actual put-call price differential at a strike price of $40: $ The -Select price is undervalued relative to the - Select option. The arbitrage transaction requires the purchase of the - Select option while shorting the - Select option. We can obtain an arbitrage profit of $







