Question: Consider general Gaussian basis function of the form: 1 = 2 = /1 (2) xp { - 1 (2 - 1 ) 8 -

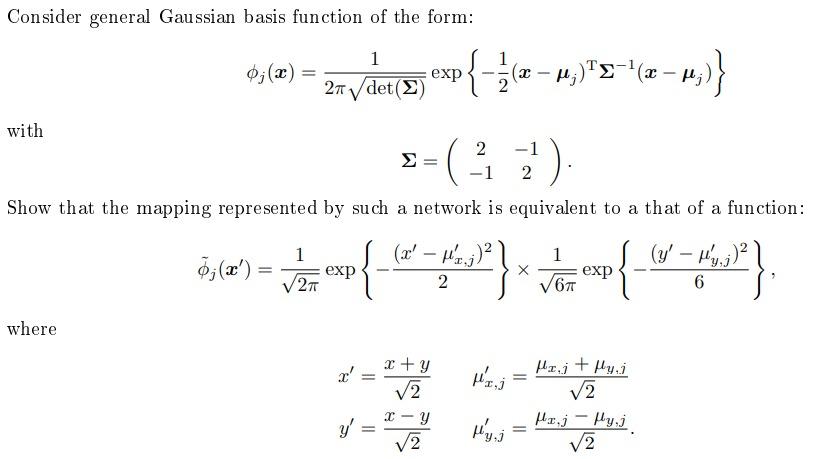
Consider general Gaussian basis function of the form: 1 = 2 =

Consider general Gaussian basis function of the form: 1 = 2 = /1 (2) "xp { - 1 (2 - 1 ) 8 - (2- exp{-1/(x-)TE-(x - -1)} with j(x): (32) Show that the mapping represented by such a network is equivalent to a that of a function: where 1 $(x) = -2/2/ /2T 1 (x' - Pr.j) {*}{*-*. X exp 6 exp x' y' = = x+y 2 X- y 2 2 Hy.j = = Haj + Hyj 2 Haj Hyj 2 (y' - 'y,j) 6

Step by Step Solution

★★★★★

3.33 Rating (150 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To show that the mapping represented by the first function is equivalent to the second fu... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

A polynomial on Rn is a function of the form where the aj1,. . . . . .. j n 's are scalars and N1,...,Nn N. Prove that if A is compact in Rn and f C(A), then there is a sequence of polynomials Pk on...

Is there any function of the form y = 0

A competitive firm has a production function of the form Y = 2L + 5K. If w = $2 and r = $3, what will be the minimum cost of producing 10 units of output?

QUESTION 3 CPI-U in 1970 was 38.8. CPI-U in 1980 was 82.4. CPI-U in 1990 was 130.7 What is the percentage change in price level from 1970 to 1990? O 43.6% O 48.3% O 58.6% O 91.9% O 112.4% 236.9%

Please #1, 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25 1-4 Evaluate the integral using integration by parts with the 9. f w In w dw 10. In x -2 . dx indicated choices of u and du. 1. (xedx; u = x, du...

Please #27, 29, 31, 33, 35, 37, 39, 41,45, 57, 61, 69, 77 1-4 Evaluate the integral using integration by parts with the 9. f w In w dw 10. In x -2 . dx indicated choices of u and du. 1. (xedx; u = x,...

I'm having issues with the itemized deductions section - I am coming up with $22,778 when the answer should be $23,238. Can you assist me? INDIVIDUAL TAX RETURN PROBLEM 5 Required: Use the following...

Bivariate gaussian Problem 1. Consider the problem [3 points] 1 + 13 =0 0 0 u(x, 0) = uo(X) 0 0 3 %Upwind method is used clear , clf , hold off %clear variables and graph N=100; %%number of...

During the depression of the 1930s, Ben Jenkins, Sr., a wealthy, expansion-oriented lumberman whose family had been in the lumber business in the southeaster United States for several generations,...

This will be an individual rather than group assignment. Design a first-generation knowledge management system for your library. You may choose either the library from your group assingments or from...

1. What does that mean? 2. Does that leave the Lees without any legal remedy?

Which of the following is true with regards to progressive taxes: a . Tax rate is at a foxed rate b . Tax rate decreases with the amount of income earned c . The tax rate increases with the amount of...

How much money should be deposited today in an account that earns 4.5% compounded monthly so that it will accumulate to $14,000 in 2 years? Click the icon to view some finance formulas. The amount of...

37m left H ALL O 1 2 3 4 5 7 8 5. Minimum Processing Time A computing cluster has multiple processors, each with 4 cores. The number of tasks to handle is equal to the total number of cores in the...

The production planning period for flat-screen monitors at Louisiana's Roa Electronics. Inc., is 4 months. Cost data are as follows: Regular-time cost per monitor $ 70 Overtime cost per monitor $110...

Summarize the percentage-of-sales technique used to forecast the required assets short-term liabilities in the Projected Balance Sheets

Identify and describe your current life stage. How does your current age or life stage affect your financial thinking and behavior? To what extent and in what ways does your financial thinking...

Seeding is the process of finding the key decision maker within a buying center. Group of answer choices true false

As a variation of the gambler's ruin problem, suppose that on each play, the probability of winning is ½ and the gambler stops playing if he accumulates $4 or goes broke. (a) What is the...

According to an article in the New York Times on August 9, 2008, economists were predicting that the average interest rate for a 30-year mortgage would increase from 6.7% to 7.1% during the next year...

Find n (S T), given that n (S) = 4, n (T) = 12, and n (S T) = 15.

Three points are identified on the graph in Figure 4P-1. a. At point A, demand is ____. b. At point B, demand is ____. c. At point C, demand is ____.

You have noticed that the price of tickets to your universitys basketball games keeps increasing but the supply of tickets remains the same. Why might supply be unresponsive to changes in price?

When the price of a bar of chocolate is $1, demand is 100,000 bars. When the price rises to $1.50, demand falls to 60,000 bars. Calculate the price elasticity of demand according to the instructions...