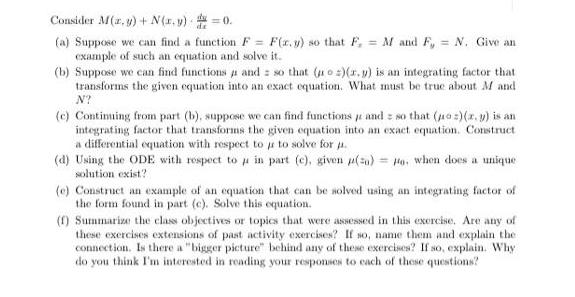
Consider M(x, y) + N(x,y)=0. (a) Suppose we can find a function F = F(x,y) so that F, = M and F, N. Give an example of such an equation and solve it. (b) Suppose we can find functions and so that (o)(r.) is an integrating factor that transforms the given equation into an exact equation. What must be true about M and N? (c) Continuing from part (b), suppose we can find functions and so that (o)(x, y) is an integrating factor that transforms the given equation into an exact equation. Construct a differential equation with respect to to solve for p. (d) Using the ODE with respect to u in part (c), given p(a) Ho, when does a unique solution exist? (e) Construct an example of an equation that can be solved using an integrating factor of the form found in part (c). Solve this equation. (f) Summarize the class objectives or topics that were assessed in this exercise. Are any of these exercises extensions of past activity exercises? If so, name them and explain the connection. Is there a "bigger picture" behind any of these exercises? If so, explain. Why do you think I'm interested in reading your responses to each of these questions?