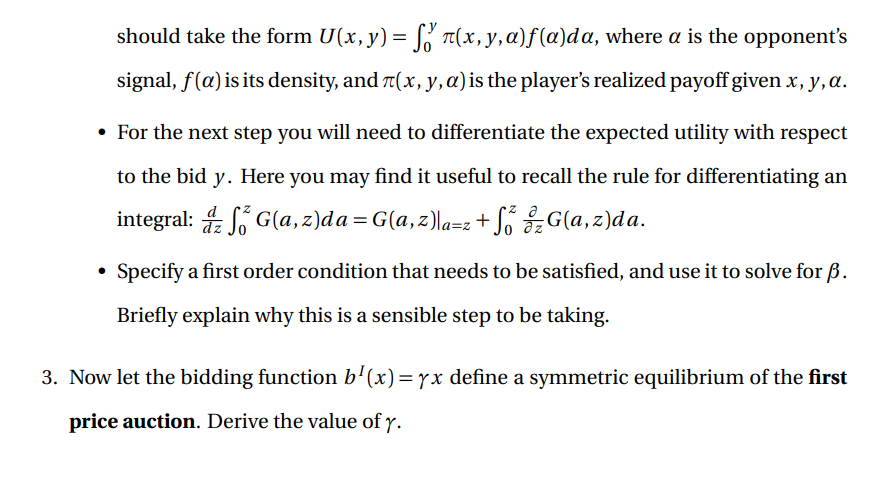
Consider the auction of a single indivisible object to two risk neutral bidders, each of whom receives a private signal X; (i = {1,2}) which is independently and uniformly distributed on the interval [1,3]. Suppose bidders have a common value V where V = X+X2. 1. Illustrate the Winner's curse in the context of this example and second price auctions. In particular, if all bidders bid "naively" are there values of a bidder's signal for which a bidder experiences "expected" losses? 2. Let the bidding function b (x) = x define a symmetric equilibrium of the second price auction. Derive the value of . To help you out, you are advised to structure your answer in accordance with the fol- lowing format: Fixing her opponent's strategy, express the expected payoff for one player when she has received signal x and bids as if she has received signal y. Your answer should take the form U(x, y) = f(x, y,)f (a)da, where a is the opponent's signal, f(a) is its density, and (x, y, ) is the player's realized payoff given x, y, a. For the next step you will need to differentiate the expected utility with respect to the bid y. Here you may find it useful to recall the rule for differentiating an integral: G(a,z)da = G(a,z)|a=z + fG(a,z)da. Specify a first order condition that needs to be satisfied, and use it to solve for . Briefly explain why this is a sensible step to be taking. 3. Now let the bidding function b(x) = yx define a symmetric equilibrium of the first price auction. Derive the value of y.