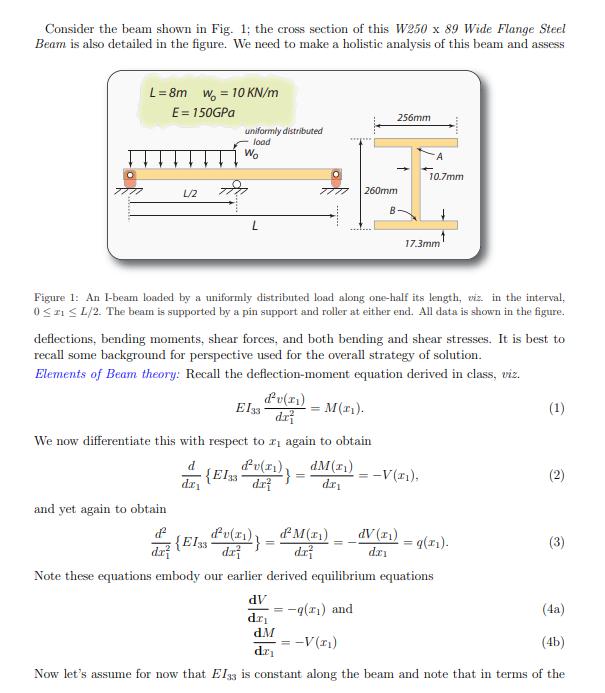
Consider the beam shown in Fig. 1; the cross section of this W250 x 89 Wide Flange Steel Beam is also detailed in the figure. We need to make a holistic analysis of this beam and assess L=8m w = 10 KN/m E = 150GPa L/2 uniformly distributed load W and yet again to obtain L engin EI33 We now differentiate this with respect to again to obtain dv(x). dM () {EI33 dr dr dv(21) Figure 1: An I-beam loaded by a uniformly distributed load along one-half its length, viz. in the interval, 021 L/2. The beam is supported by a pin support and roller at either end. All data is shown in the figure. = M(x). &M () dr 260mm deflections, bending moments, shear forces, and both bending and shear stresses. It is best to recall some background for perspective used for the overall strategy of solution. Elements of Beam theory: Recall the deflection-moment equation derived in class, viz. d'v(1) 256mm B = -V() 17.3mm A 10.7mm =-V(x). dV (2) dri {Elss Note these equations embody our earlier derived equilibrium equations dv -q() and dr = =q(x). (1) (2) (3) (4a) dM (4b) dz Now let's assume for now that EI33 is constant along the beam and note that in terms of the deflection () we then have M(2) = EI33 (5a) (5b) =q(*). (5c) The reason the last of these equations is written in the orientation it has is due to our strategy of solution that is adopted for this analysis. Note that eqs. 5a,b are equations that give shear force and bending moment, respectively. Let us define the tasks and procedure. d'v(x) dzi dv(x1) V(2) = - E133 d'v(2) da El 33 1a. Since the beam has no interior supports, write the expression for q(z) for the applied loading using singularity functions such as (ra)" and use it to construct eq. 5c. 1b. Integrate the 4th order ordinary differential equation obtained from eq. 5c, and express the various integration constants along the way. At each integration bear in mind eqs. 5a.b and ask yourself if you can identify values for any such constants due to knowledge of either moments or shear forces. Note, however, you have not yet performed any pre-analysis via the usual methods of statics. Note that as you began you knew all information about the "applied forces" and it is only the integration constants that require evaluation. 1b-a. Bearing in mind eqs. 5a.b, write down equations for shear force and bending moment as soon as you can even though they may contain integration constants yet unknown. 1c. Invoke all boundary conditions (bc's) and evaluate all integration constants and construct the shear force and bending moment diagrams and sketch them neatly. Identify key points such as maxima and minima along these diagrams. Plot these diagrams in terms of wo and L; you will insert actual numbers later when evaluating stresses. Note you should have been evaluating integration constants as you performed integrations as soon as you were able to. 1d. Evaluate the complete deflection curve, i.e. v(2), and sketch it in terms of the parameters, wo, L, and EI33. However, now put in numbers and compute and indicate the actual maximum deflection at the point it occurs. 1e. Evaluate the moment of inertia for this cross section and evaluate the maximum bending stresses, both the extreme tensile and compressive bending stresses and indicate their locations along the beam. 1f. Evaluate the maximum shear stress, and indicate its location. Also, evaluate the shear stress in the web just below the points at the top and bottom of the beam where the web meets the flanges; these points are labeled A and B in Fig. 1. Evaluate the shear stress at the neutral axis of the beam. Perform all evaluations such as Q(C) as original calculations without appealing to canned formulas. 1g. Compute the ratio of maximum (in magnitude) shear stress to bending stress.