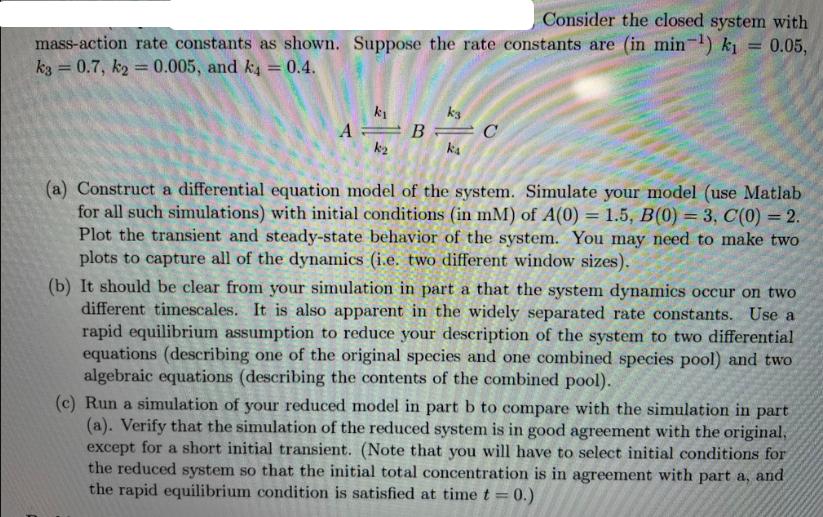
Consider the closed system with mass-action rate constants as shown. Suppose the rate constants are (in min-1) k = 0.05, kg = 0.7, k2= 0.005, and k4 = 0.4. ki k3 A B C k2 ka (a) Construct a differential equation model of the system. Simulate your model (use Matlab for all such simulations) with initial conditions (in mM) of A(0) = 1.5, B(0) = 3, C(0) = 2. Plot the transient and steady-state behavior of the system. You may need to make two plots to capture all of the dynamics (i.e. two different window sizes). (b) It should be clear from your simulation in part a that the system dynamics occur on two different timescales. It is also apparent in the widely separated rate constants. Use a rapid equilibrium assumption to reduce your description of the system to two differential equations (describing one of the original species and one combined species pool) and two algebraic equations (describing the contents of the combined pool). (c) Run a simulation of your reduced model in part b to compare with the simulation in part (a). Verify that the simulation of the reduced system is in good agreement with the original, except for a short initial transient. (Note that you will have to select initial conditions for the reduced system so that the initial total concentration is in agreement with part a, and the rapid equilibrium condition is satisfied at time t = 0.)