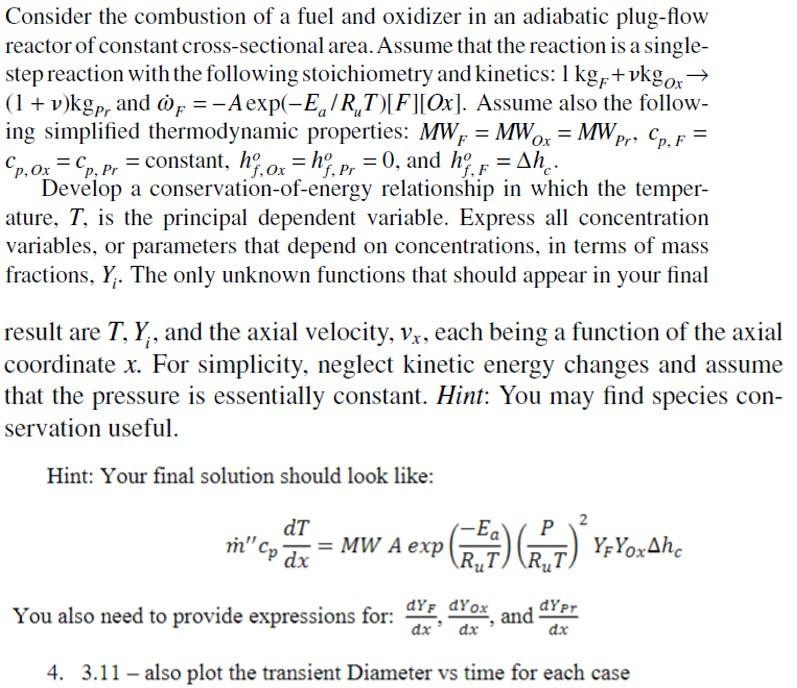
Consider the combustion of a fuel and oxidizer in an adiabatic plug-flow reactor of constant cross-sectional area. Assume that the reaction is a singlestep reaction with the following stoichiometry and kinetics: 1kgF+vkgOx (1+v)kgPr and F=Aexp(Ea/RuT)[F][Ox]. Assume also the following simplified thermodynamic properties: MWF=MWOx=MWPr,cp,F= cp,Ox=cp,Pr= constant, hf,Oxo=hf,Pro=0, and hf,Fo=hc. Develop a conservation-of-energy relationship in which the temperature, T, is the principal dependent variable. Express all concentration variables, or parameters that depend on concentrations, in terms of mass fractions, Yi. The only unknown functions that should appear in your final result are T,Yi, and the axial velocity, vx, each being a function of the axia coordinate x. For simplicity, neglect kinetic energy changes and assume that the pressure is essentially constant. Hint: You may find species conservation useful. Hint: Your final solution should look like: mcpdxdT=MWAexp(RuTEa)(RuTP)2YFYOxhc You also need to provide expressions for: dxdYF,dxdYox, and dxdYPr 4. 3.11 - also plot the transient Diameter vs time for each case Consider the combustion of a fuel and oxidizer in an adiabatic plug-flow reactor of constant cross-sectional area. Assume that the reaction is a singlestep reaction with the following stoichiometry and kinetics: 1kgF+vkgOx (1+v)kgPr and F=Aexp(Ea/RuT)[F][Ox]. Assume also the following simplified thermodynamic properties: MWF=MWOx=MWPr,cp,F= cp,Ox=cp,Pr= constant, hf,Oxo=hf,Pro=0, and hf,Fo=hc. Develop a conservation-of-energy relationship in which the temperature, T, is the principal dependent variable. Express all concentration variables, or parameters that depend on concentrations, in terms of mass fractions, Yi. The only unknown functions that should appear in your final result are T,Yi, and the axial velocity, vx, each being a function of the axia coordinate x. For simplicity, neglect kinetic energy changes and assume that the pressure is essentially constant. Hint: You may find species conservation useful. Hint: Your final solution should look like: mcpdxdT=MWAexp(RuTEa)(RuTP)2YFYOxhc You also need to provide expressions for: dxdYF,dxdYox, and dxdYPr 4. 3.11 - also plot the transient Diameter vs time for each case