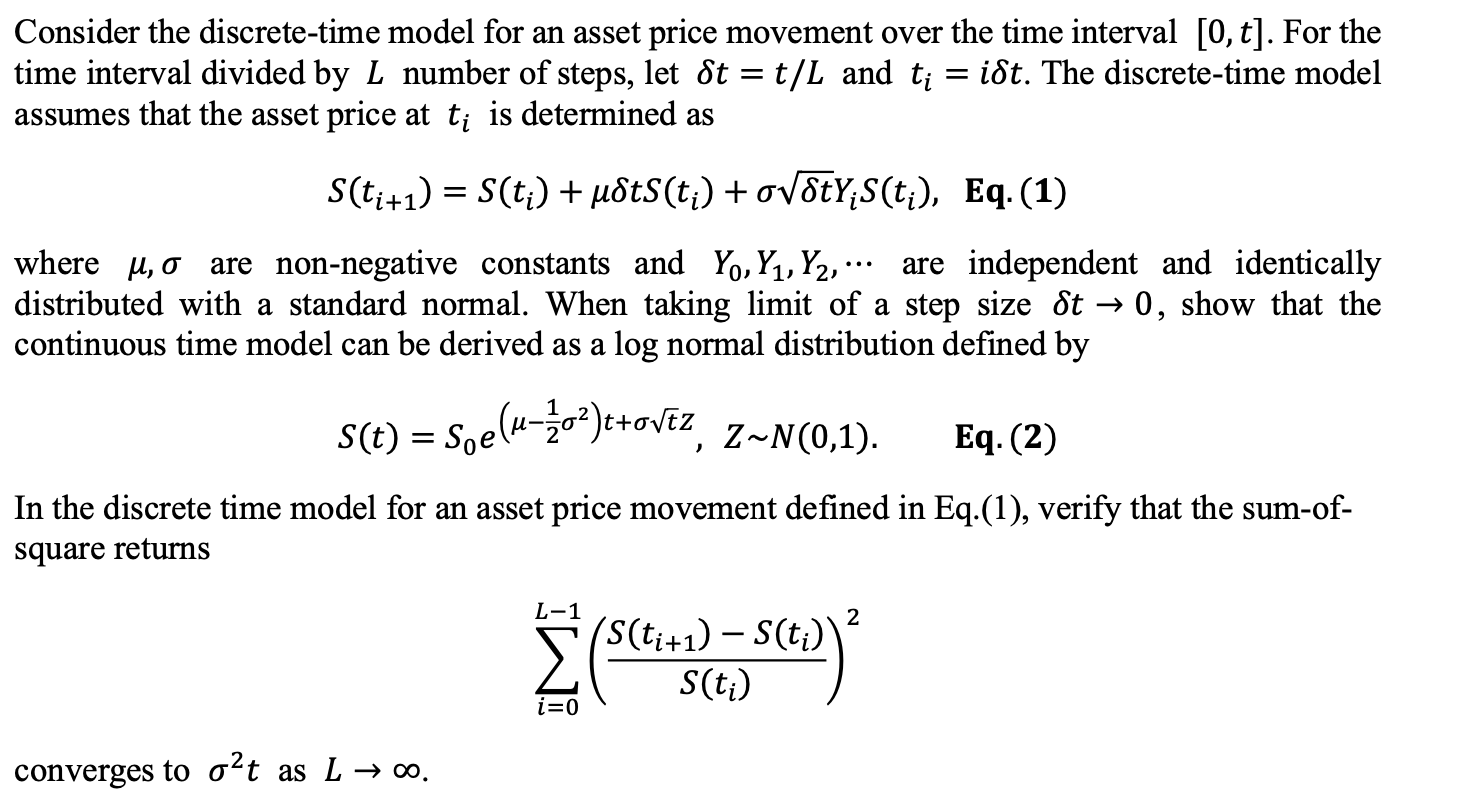
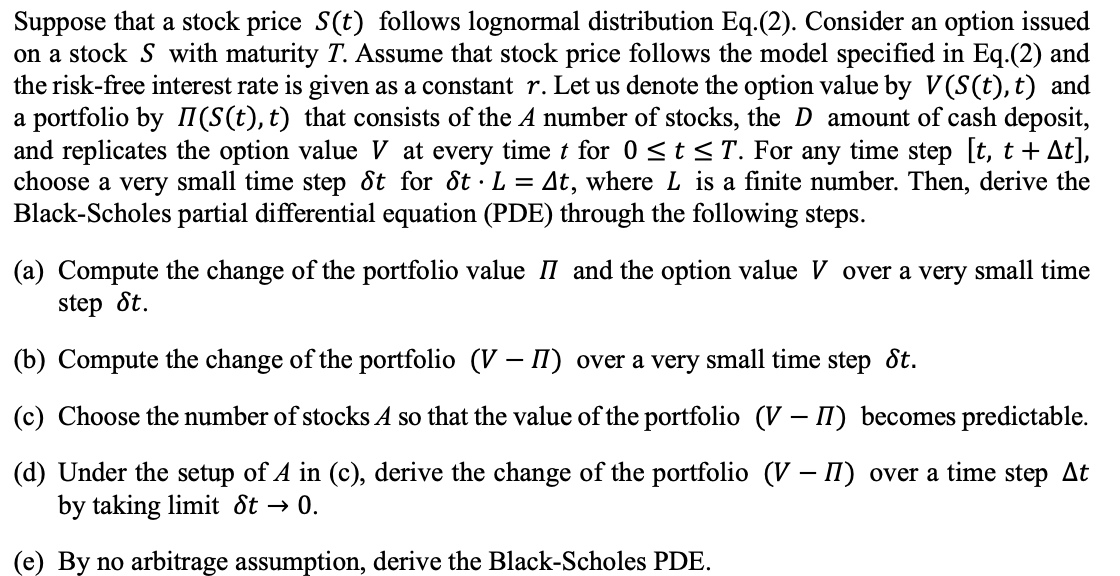
Consider the discrete-time model for an asset price movement over the time interval [0, t]. For the time interval divided by L number of steps, let St = t/L and t idt. The discrete-time model assumes that the asset price at ti is determined as = S(ti+1) = S(ti) + udts(t:) +ovStys(t;), Eq. (1) where u, o are non-negative constants and Yo, Y,Y2,... are independent and identically distributed with a standard normal. When taking limit of a step size St 0, show that the continuous time model can be derived as a log normal distribution defined by S(t) = Soek-202)e+ovez, 2-N(0,1). Z~) Eq.(2) In the discrete time model for an asset price movement defined in Eq.(1), verify that the sum-of- square returns L-1 2 (S(ti+1) S(t;) s(t) i=0 converges to o2t as L 700. Suppose that a stock price S(t) follows lognormal distribution Eq.(2). Consider an option issued on a stock S with maturity T. Assume that stock price follows the model specified in Eq.(2) and the risk-free interest rate is given as a constant r. Let us denote the option value by V(S(t), t) and a portfolio by I(S(t), t) that consists of the A number of stocks, the D amount of cash deposit, and replicates the option value V at every time t for 0 st ST. For any time step [t, t + At], choose a very small time step dt for dt. L = At, where L is a finite number. Then, derive the Black-Scholes partial differential equation (PDE) through the following steps. (a) Compute the change of the portfolio value II and the option value V over a very small time step dt. (b) Compute the change of the portfolio (V II) over a very small time step St. (c) Choose the number of stocks A so that the value of the portfolio (V II) becomes predictable. (d) Under the setup of A in (c), derive the change of the portfolio (V II) over a time step At by taking limit St 0. (e) By no arbitrage assumption, derive the Black-Scholes PDE. Consider the discrete-time model for an asset price movement over the time interval [0, t]. For the time interval divided by L number of steps, let St = t/L and t idt. The discrete-time model assumes that the asset price at ti is determined as = S(ti+1) = S(ti) + udts(t:) +ovStys(t;), Eq. (1) where u, o are non-negative constants and Yo, Y,Y2,... are independent and identically distributed with a standard normal. When taking limit of a step size St 0, show that the continuous time model can be derived as a log normal distribution defined by S(t) = Soek-202)e+ovez, 2-N(0,1). Z~) Eq.(2) In the discrete time model for an asset price movement defined in Eq.(1), verify that the sum-of- square returns L-1 2 (S(ti+1) S(t;) s(t) i=0 converges to o2t as L 700. Suppose that a stock price S(t) follows lognormal distribution Eq.(2). Consider an option issued on a stock S with maturity T. Assume that stock price follows the model specified in Eq.(2) and the risk-free interest rate is given as a constant r. Let us denote the option value by V(S(t), t) and a portfolio by I(S(t), t) that consists of the A number of stocks, the D amount of cash deposit, and replicates the option value V at every time t for 0 st ST. For any time step [t, t + At], choose a very small time step dt for dt. L = At, where L is a finite number. Then, derive the Black-Scholes partial differential equation (PDE) through the following steps. (a) Compute the change of the portfolio value II and the option value V over a very small time step dt. (b) Compute the change of the portfolio (V II) over a very small time step St. (c) Choose the number of stocks A so that the value of the portfolio (V II) becomes predictable. (d) Under the setup of A in (c), derive the change of the portfolio (V II) over a time step At by taking limit St 0. (e) By no arbitrage assumption, derive the Black-Scholes PDE








