Question: Consider the following 2 by 3 matrix, A = 1 0 -2 1 0 (a) Find the right singular vectors i.e. eigenvectors of ATA.

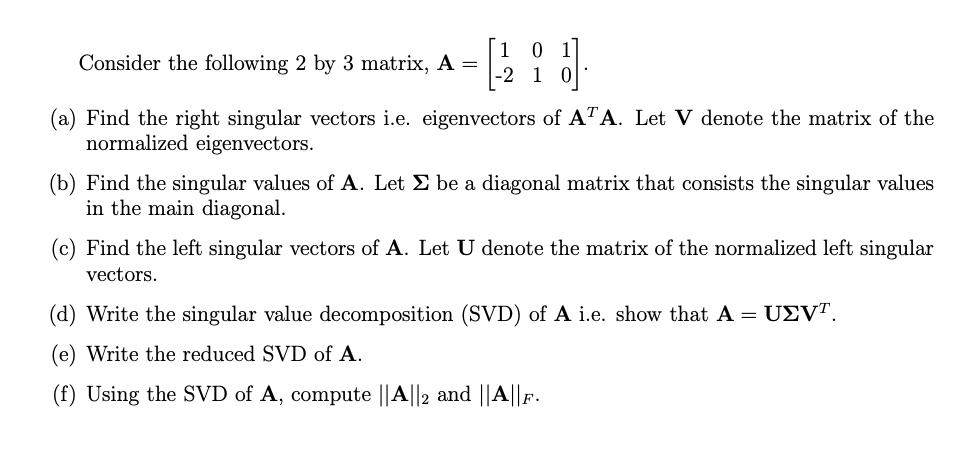
Consider the following 2 by 3 matrix, A = 1 0 -2

Consider the following 2 by 3 matrix, A = 1 0 -2 1 0 (a) Find the right singular vectors i.e. eigenvectors of ATA. Let V denote the matrix of the normalized eigenvectors. (b) Find the singular values of A. Let be a diagonal matrix that consists the singular values in the main diagonal. (c) Find the left singular vectors of A. Let U denote the matrix of the normalized left singular vectors. (d) Write the singular value decomposition (SVD) of A i.e. show that A = UZVT. (e) Write the reduced SVD of A. (f) Using the SVD of A, compute ||A||2 and ||A||F.

Step by Step Solution

★★★★★

3.37 Rating (153 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock blur-text-image

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Consider the following 2 x 3 (i.e., r = 2 and c = 3) contingency table: a. Specify the null and alternative hypotheses that should be used in testing the independence of the row and column...

Let A be a matrix whose singular value decomposition is given by Make use of the singular value decomposition to do each of the following. (a) Determine the rank of A. (b) Find an orthonormal basis...

The matrix has singular value decomposition Use the singular value decomposition to find orthonormal bases for R(A) and N(AT). 4004 5335 2662 1-3 2-3 2-3 2-3 2-3 1-3 0600 2000

Using MATLAB!!! Consider the following 6 times 6 matrix A = (1 0 1 -2 -4 7 -2 1 0 1 1 -5 3 -2 -3 -1 3 -3 -2 -1 2 0 -5 2 -1 5 1 3 2 5 -2 1 2 2 5 -4) (a) Use rref to find the solution to the...

3.1.5 Matrix transpose Matrix transpose is the operation consisting in swapping the rows of a matrix for its columns. It is a basic matrix operation used in many real-life applications such as image...

Q.1. Due to an aggressive marketing strategy, Balanced Body, an OEM of fitness equipment, has recently seen an increase in the number of demand for their MOTR series fitness tool. The production...

CSC108 Assignment 1: The Slide Game In Assignment 1, you write Python code that will be used by a game called The Slide Game. You can complete the whole assignment with only the concepts from Weeks...

Which of the following statements are true about the F-ratio in ANOVA? It is the ratio of the sum of the squares between to the sum of the squares within it can be either positive or negative...

Submit Answer 4. [-/1 Points] DETAILS LARCALC11 11.2.042. Find the standard equation of the sphere with the given characteristics. Center: (-6, 0, 0), tangent to the yz-plane Need Help? Read It...

(a) Find the fundamental frequency and second harmonic if the tension in the wire is increased to116N. f fundamental =Hz f 2 nd harmonic =Hz (b) Using a sound speed of 343 m/s, find the wavelengths...

Marshalls has several departments that occupy all floors of a two-story building that includes a basement floor. Marshall rented this building under a long-term lease negotiated when rental rates...

How would any management fees charged by a parent company to its subsidiary be accounted for during the consoldation proced Mutiple Choice The parent company would enly mecord th pro uts share of any...

Show that Reynold number, pud/, is non-dimensional. If the discharge Q through an orifice is a function of the diameter d, the pressure difference p, the density p, and the viscosity u 1/2 2 show...

6. Evaluate the following integrals: Vx dx a) J Tt/2 e2x sin x dx 1+ Vx

4. Find the derivatives of the following functions: a) y2 sin x - tan (x + y) +3x = 0 b) y = x Inx

2. Given, A-1 = a) Use the above inverse matrix to solve the system of linear equations AX = b, if b" = [-1 7]. b) Write the linear equations themselves.

5. Compute the volume of the solid obtained by revolving the curve y = vx sin x about the x-axis between x = 0 and x = n / 2.

9 More Trusted Sites for Buying Verified Binance Accounts: Secure Choices Do you want a verified Binance account? A verified account lets you send and receive money. You can do it easily and safely....

(a) Find the monic Legendre polynomial of degree 5 using the Gram-Schmidt process. Check your answer by using the Rodrigues formula. (b) Use orthogonality to write t5 as linear combinations of...

Use the projection matrix method of Exercise 5.5.26 to find the orthogonal projection of v = (1,0, 0,0)T onto the range of the following matrices: (a) (b) (c) (d) 5571 0212 1101 1122 2311 1210 0012

Show that the subspace of skew-symmetric n n matrices forms a complementary subspace to the space of symmetric n n matrices. Explain why this implies that every square matrix can be uniquely...

Describe three ways in which businesses are able to sell to other businesses using the Internet.

Describe four reasons mobile devices used in business are difficult to secure.

American Institute of Certified Public Accountants. Go to the Web site of the American Institute of Certified Public Accountants (AICPA; http://www.aicpa.org). Review various parts of the site (e.g.,...