Consider the following statements.

(i) Given a second-order linear ODE, the method of variation of parameters gives a particular solution in terms of an integral provided y₁ and y₂ can be found.

(ii) The Laplace Transform is an integral transform that turns the problem of solving constant coefficient ODEs into an algebraic problem. This transform is particularly useful when it comes to studying problems arising in applications where the forcing function in the ODE is piece-wise continuous but not necessarily continuous, or when it comes to studying some Volterra equations and integro-differential equations.

(iii) If P and Q are continuous on R, then we can apply the idea of the method of variation of parameters to y + P(x)y = Q(x) and assume y = u₁y₁ where y₁ solves y + P(x)y = 0. That way, we would obtain the same result the integrating factor method would give.

(iv) The Laplace Transform of 43te^t2cos(e^t2) is well-defined for some values of s.

(v) To compute solutions to a homogeneous second-order Cauchy-Euler equation, there are three different cases to be considered, depending on whether the characteristic equation associated with the ODE has roots that are real and distinct, or real and equal, or complex. If the equation is non-homogeneous, then we cannot use any method we covered to find a particular solution.

(vi) The following is an example of a piece-wise continuous function f(x) =

{	1 x	x 0
	0	x = 0

Determine which of the above statements are True (1) or False (2).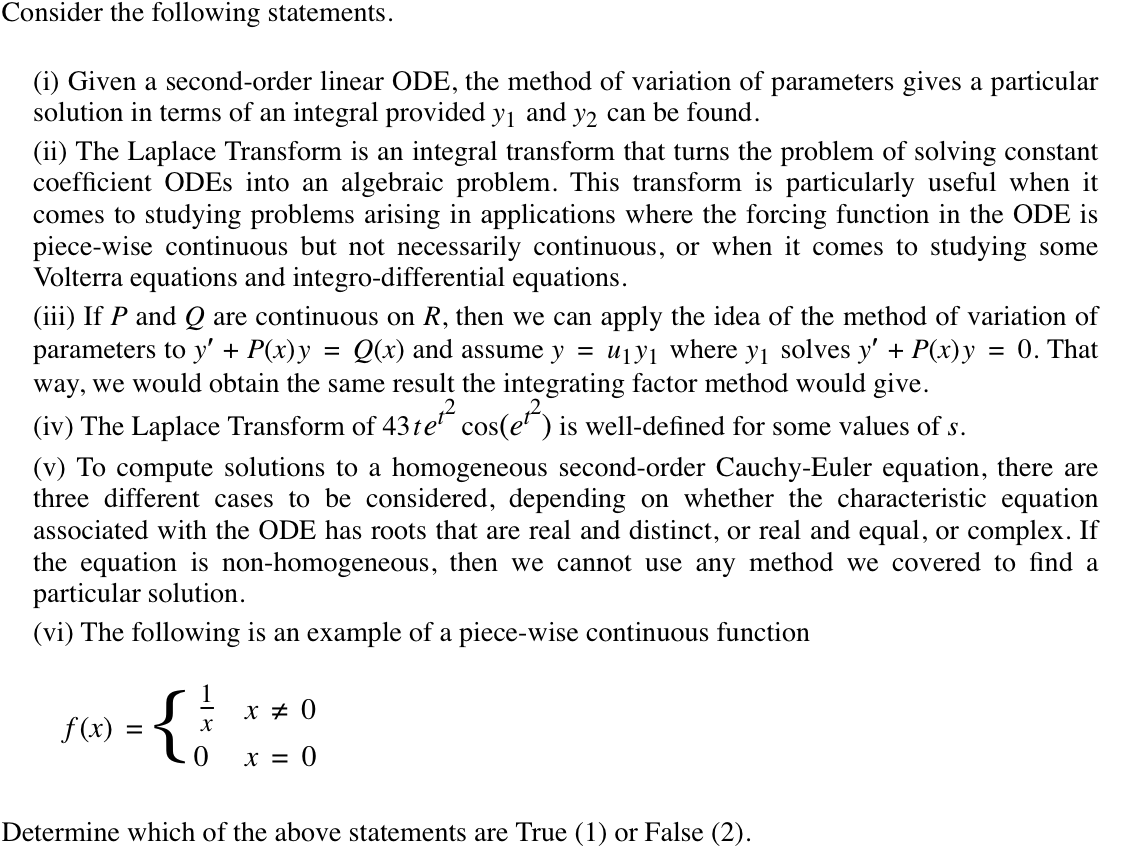

Consider the following statements. (i) Given a second-order linear ODE, the method of variation of parameters gives a particular solution in terms of an integral provided yi and y2 can be found. (ii) The Laplace Transform is an integral transform that turns the problem of solving constant coefficient ODEs into an algebraic problem. This transform is particularly useful when it comes to studying problems arising in applications where the forcing function in the ODE is piece-wise continuous but not necessarily continuous, or when it comes to studying some Volterra equations and integro-differential equations. (iii) If P and Q are continuous on R, then we can apply the idea of the method of variation of parameters to y' + P(x)y = Q(x) and assume y = ujyi where y solves y' + P(x)y = 0. That way, we would obtain the same result the integrating factor method would give. (iv) The Laplace Transform of 43 te cos(e) is well-defined for some values of s. (v) To compute solutions to a homogeneous second-order Cauchy-Euler equation, there are three different cases to be considered, depending on whether the characteristic equation associated with the ODE has roots that are real and distinct, or real and equal, or complex. If the equation is non-homogeneous, then we cannot use any method we covered to find a particular solution. (vi) The following is an example of a piece-wise continuous function XE 0 f(x) X 0 x = 0 Determine which of the above statements are True (1) or False (2)