Consider the function eax x > 0 f ( xx ) = 1/2 x =0 0 x

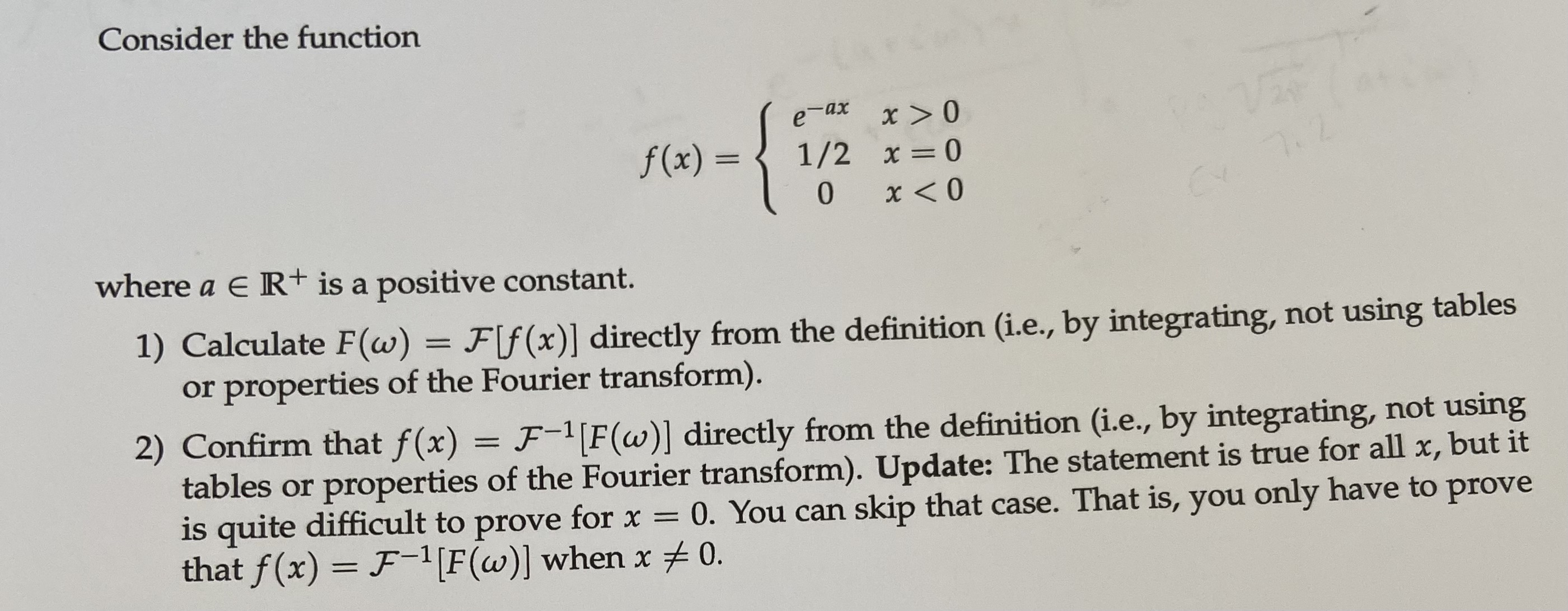
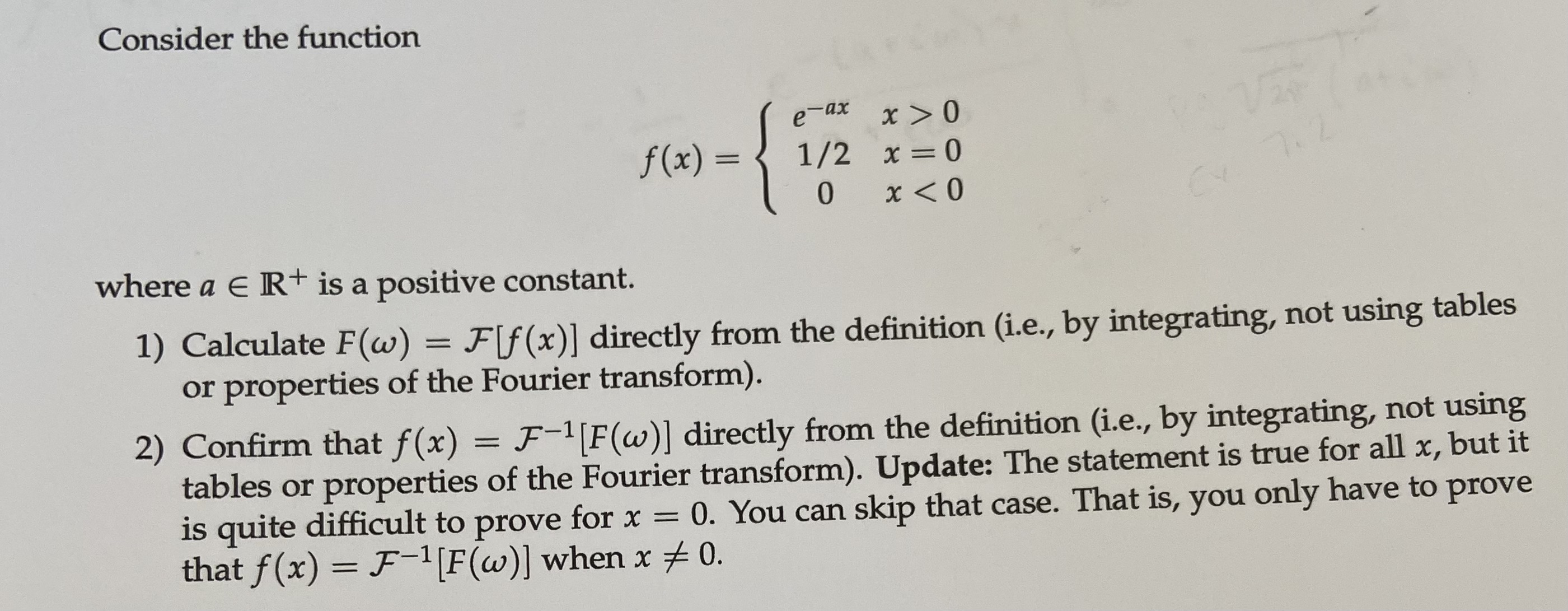
Consider the function f(x) where a e R + is a positive constant. ax e 1/2 0 1) Calculate F(,') F [f (x)] directly from the definition (i.e., by integrating, not using tables or properties of the Fourier transform). 2) Confirm that f (x) [F(w)] directly from the definition (i.e., by integrating, not using tables or properties of the Fourier transform). Update: The statement is frue for all x, but it is quite difficult to prove for x = 0. You can skip that case. That is, you only have to prove that f (x) = [F(w)] when x # 0.