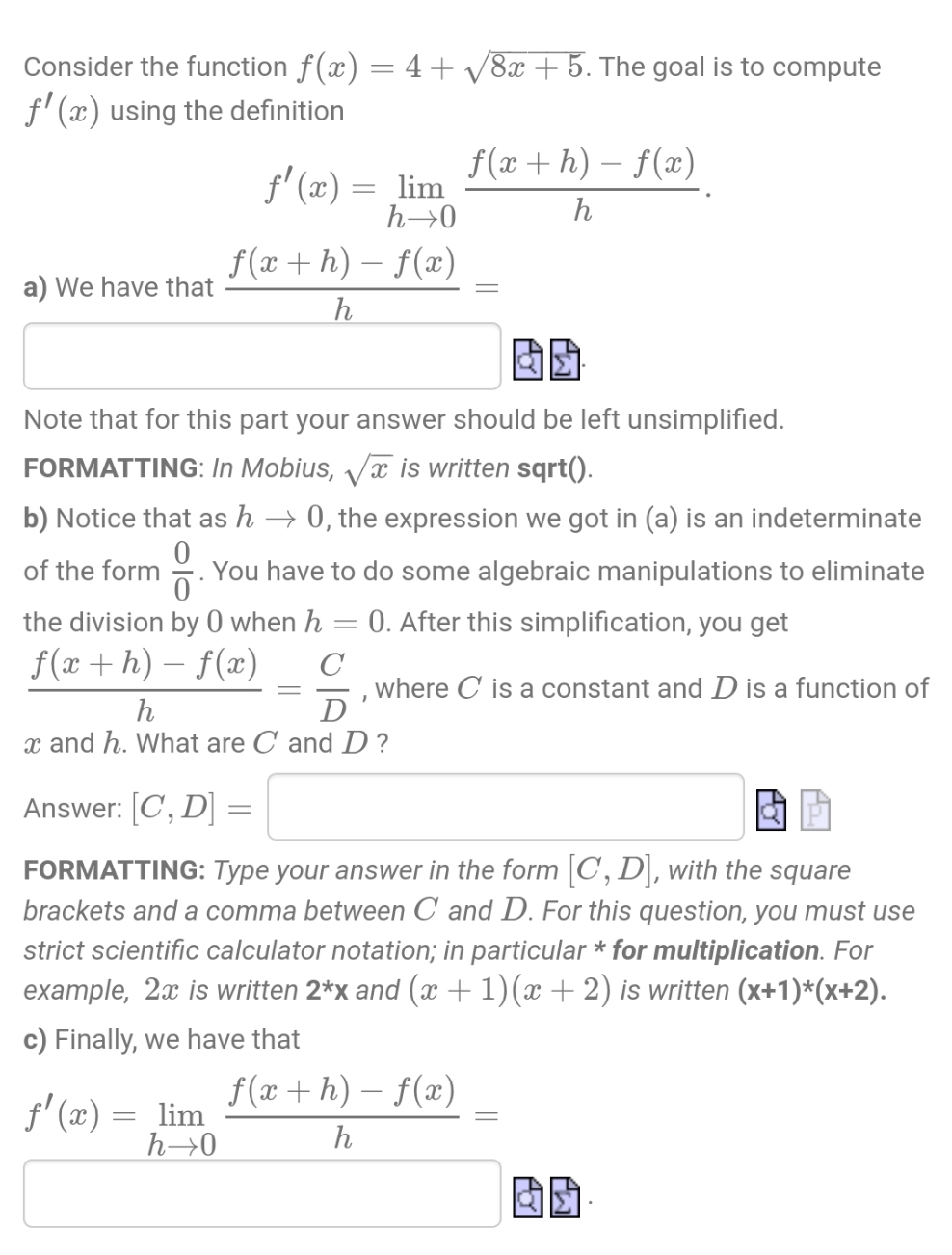
Consider the function f(x) = 4+ /8x + 5. The goal is to compute f'(x) using the definition f(x + h) f(x) = f'(x) = lim h0 h f(x + h) f(x) a) We have that h Note that for this part your answer should be left unsimplified. FORMATTING: In Mobius, is written sqrt(). b) Notice that as h0, the expression we got in (a) is an indeterminate 0 of the form You have to do some algebraic manipulations to eliminate the division by 0 when h = 0. After this simplification, you get f(x + h) f(x) h = C where C is a constant and D is a function of ' D x and h. What are C and D ? Answer: [C, D] = FORMATTING: Type your answer in the form [C,D], with the square brackets and a comma between C and D. For this question, you must use strict scientific calculator notation; in particular * for multiplication. For example, 2x is written 2*x and (x + 1)(x + 2) is written (x+1)*(x+2). c) Finally, we have that f'(x) = lim h0 - f(x + h) f(x) h =