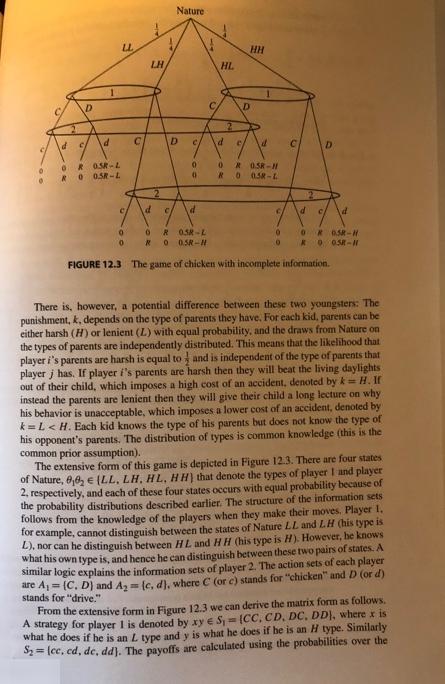
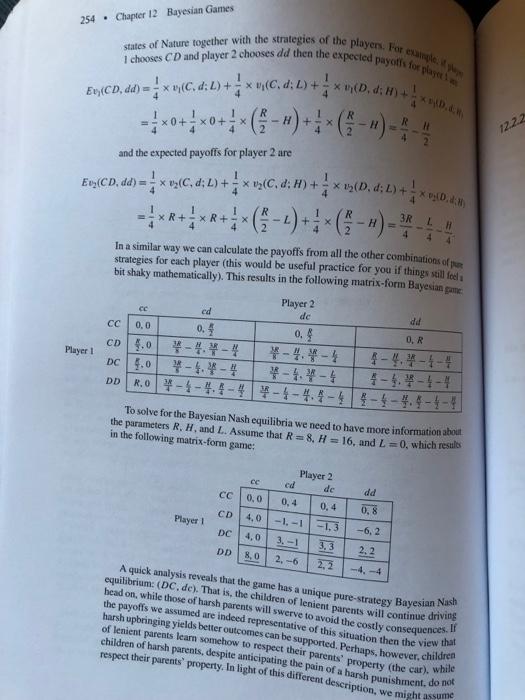
2.1 Chicken Revisited: Consider the game of chicken in Section 12.2.1 with the = parameters R = 8, H = 16, and L 0 as described there. A preacher, who knows some game theory, decides to use this model to claim that moving to a society in which all parents are lenient will have detrimental effects on the behavior of teenagers. Does equilibrium analysis support this claim? What if R = 8, H = 0, and L= 16? Com 122 252 Chapter 12 Bayesian Games 12.2 12.2.1 Just as in (12.2) we take the expectations of player i over the realizations of type 8, when player i knows his own type (hence the conditional expectation takes the form E-181, which is conditional on the realization of 8). This notation is more general in the sense that it is not restricted to a finite type space and instead can apply to a continuum of types for each player. For example, each player may have an infinite number of possible types drawn from an interval e, -10.0 with cumulative distribution F(8) and density function (0) F(0). In this case the expected payoff of player i will be written as an integral (more precisely, -1 integrals) that forms the expectations over the realizations of the other players types, and accordingly their actions as derived from their strategies. Examples This section offers two examples as illustrations of Bayesian games of incomplete information. In the first example each player has a finite number of actions and types, while in the second each player has a continuum of types. Sections 12.3-12.5 present some important applications of Bayesian games. Teenagers and the Game of Chicken It is often the case that a player who finds himself in some game of conflict will hold out and suffer in order to appear strong, instead of letting his rival get the better of him. Be it firms in the marketplace, politicians in government, countries at war, or even kids on the playground, the optimal behavior will depend on some combination of each player's tendency to be aggressive and his belief about his opponent's tendency to be aggressive. To illustrate this idea, consider a simple game of aggression that is known to many teenagers: the game of "chicken." The 1955 film Rebel Without a Cause features James Dean as a juvenile delinquent and introduced to the silver screen one rather dangerous variant of the game. In the movie two teenagers simultaneously drive their cars toward the edge of a cliff. The first one to jump out is considered chicken and loses the contest. (In the movie one dies, and coincidentally Dean himself died in a car accident just before the film was released.) Many other films have featured variations on this game of chicken, a well-known example being Grease (1978), starring John Travolta. The following example is yet another variant of the game. Two teenagers, players 1 and 2. have borrowed their parents' cars and decided to play the game of chicken as follows: They drive toward each other in the middle of a street, and just before impact they must simultaneously choose whether to be chicken and swerve to the right, or continue driving head on. If both are chicken then both gain no respect from their friends but suffer no losses; thus both get a payoff of 0. If i continues to drive while ji plays chicken then i gains all the respect, which is a payoff of R. and j gets no respect, which is worth 0. In this case both players suffer no additional losses. Finally if both continue to drive head on then they split the respect (because respect is considered to be relative), but an accident is bound to happen and they will at least be reprimanded by their parents, if not seriously injured. An accident imposes a personal loss of k on each player, so the payoff to each one is 4-k. 0 D d OR OSR-L RO 0.5R-L d C) LL C C 0 0 LH Nature D 2 d C c/ 0 0 d C HL d 2 HH D C d 0 R 0.5R-H R 0 0.58-L 6 C D d C/ d 0R 0.5R-L 00058- 0R0058- RO 0.5R-H FIGURE 12.3 The game of chicken with incomplete information. There is, however, a potential difference between these two youngsters: The punishment, k, depends on the type of parents they have. For each kid, parents can be either harsh (H) or lenient (L) with equal probability, and the draws from Nature on the types of parents are independently distributed. This means that the likelihood that player i's parents are harsh is equal to and is independent of the type of parents that player j has. If player i's parents are harsh then they will beat the living daylights out of their child, which imposes a high cost of an accident, denoted by k=H. If instead the parents are lenient then they will give their child a long lecture on why his behavior is unacceptable, which imposes a lower cost of an accident, denoted by k=L states of Nature together with the strategies of the players. For example, if playe 1 chooses CD and player 2 chooses dd then the expected payoffs for playet Ev,(CD, dd)= x vy(C. d; L) +x (C, d; L) + x (D. d; H)+D. +4 254 Chapter 12 Bayesian Games = Player 1 and the expected payoffs for player 2 are = x(C. d; L) + x v(C. d; H) + 1 1 (D. d; L) + x 03/0.48) - **+ ** + 1 (-) + 1 ( -#) - -4 -4 3R Eu, (CD, dd) = In a similar way we can calculate the payoffs from all the other combinations of pur strategies for each player (this would be useful practice for you if things still feel a bit shaky mathematically). This results in the following matrix-form Bayesian game cc ed 0.4 CC CD 4.0-4.*-* DC 4.0 *-.*-# DD 0,0 Player 1 Player 2 de 0. , *-.*-4 R.O-4-4-4-4-4-4-4 To solve for the Bayesian Nash equilibria we need to have more information about the parameters R, H, and L. Assume that R= 8, H=16, and L=0, which results in the following matrix-form game: Player 2 de ed 0,4 0,4 CC 0,0 CD 4,0 -1.-1 DC 4,0 3.-1 DD 8,0 2.-6 dd 0, R 4-4.*-4-4 4-4.*-1-1 4-4-4-4-4-4 -1.3 3,3 2,2 dd 0,8 -6,2 2,2 -4,14 A quick analysis reveals that the game has a unique pure-strategy Bayesian Nash equilibrium: (DC, de). That is, the children of lenient parents will continue driving head on, while those of harsh parents will swerve to avoid the costly consequences. If the payoffs we assumed are indeed representative of this situation then the view that harsh upbringing yields better outcomes can be supported. Perhaps, however, children of lenient parents learn somehow to respect their parents' property (the car), while children of harsh parents, despite anticipating the pain of a harsh punishment, do not respect their parents' property. In light of this different description, we might assume 12.2.2 12.2 Examples 255 that L=16 and H=0, which would result in the opposite prediction. Hence, as in any game, we need to get the payoffs right in order to feel comfortable offering policy recommendations.