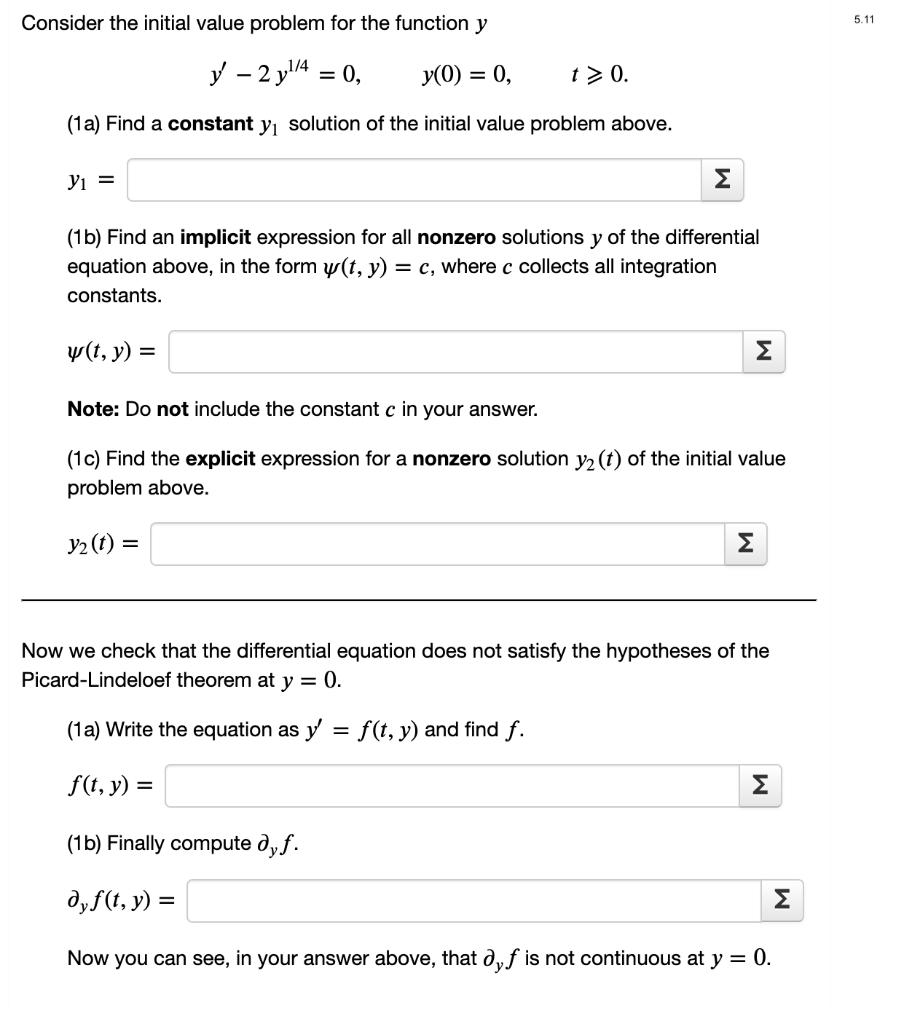
Consider the initial value problem for the function y y' - 2y1/4 = 0, y(0) = 0, t0. (1a) Find a constant y solution of the initial value problem above. y = (1b) Find an implicit expression for all nonzero solutions y of the differential equation above, in the form w(t, y) = c, where c collects all integration constants. y(t, y) = Note: Do not include the constant c in your answer. (1c) Find the explicit expression for a nonzero solution 12 (t) of the initial value problem above. y2(t) = Now we check that the differential equation does not satisfy the hypotheses of the Picard-Lindeloef theorem at y = 0. (1a) Write the equation as y' = f(t, y) and find f. f(t, y) = (1b) Finally compute dyf. dyf(t, y) = Now you can see, in your answer above, that dyf is not continuous at y = 0. 5.11