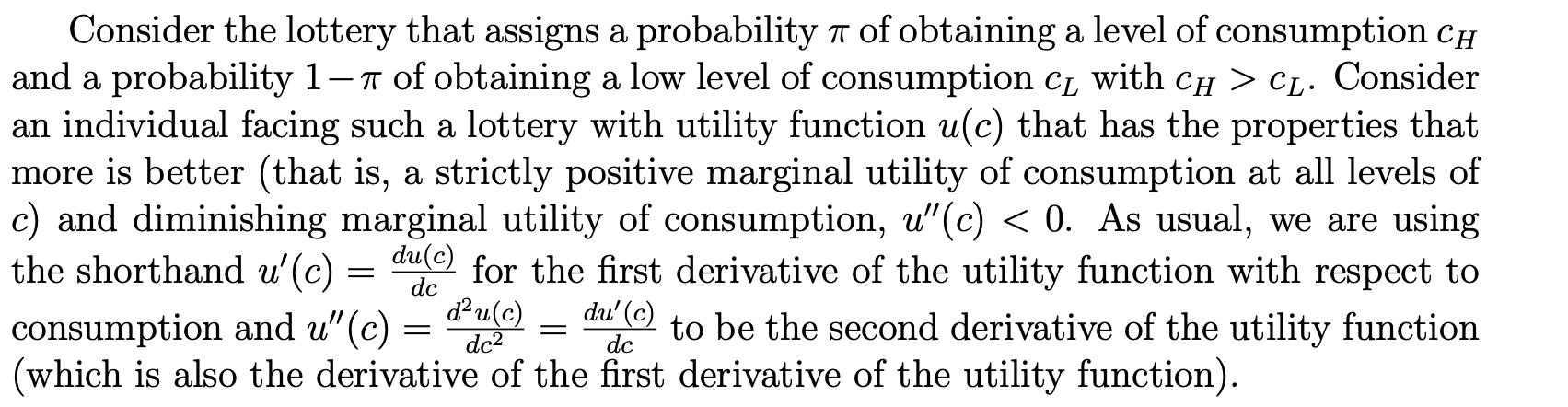
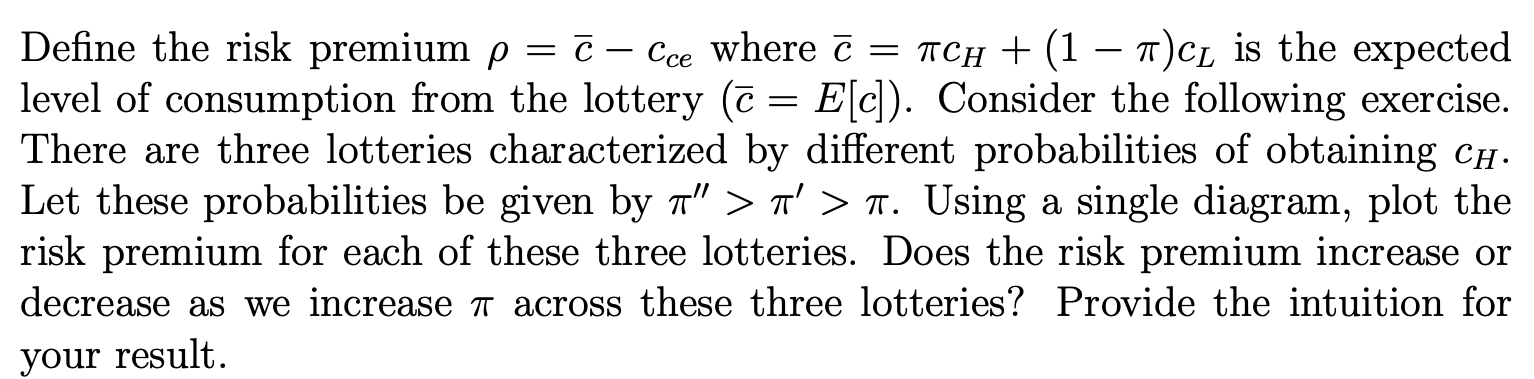
Consider the lottery that assigns a probability of obtaining a level of consumption CH and a probability 1-7 of obtaining a low level of consumption c with c > CL. Consider an individual facing such a lottery with utility function u(c) that has the properties that more is better (that is, a strictly positive marginal utility of consumption at all levels of c) and diminishing marginal utility of consumption, u"(c) < 0. As usual, we are using the shorthand u'(c) du(c) for the first derivative of the utility function with respect to du(c) du' (c) to be the second derivative of the utility function (which is also the derivative of the first derivative of the utility function). dc consumption and u"(c) dc dc = = - C c Define the risk premium p = - Cce where = TCH + (1 - )C is the expected level of consumption from the lottery (c = E[c]). Consider the following exercise. There are three lotteries characterized by different probabilities of obtaining c. Let these probabilities be given by " > ' > . Using a single diagram, plot the risk premium for each of these three lotteries. Does the risk premium increase or decrease as we increase across these three lotteries? Provide the intuition for your result.